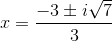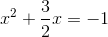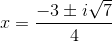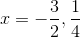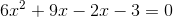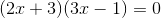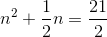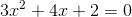All High School Math Resources
Example Questions
Example Question #1 : Completing The Square
Find the vertex of the parabola by completing the square.
To find the vertex of a parabola, we must put the equation into the vertex form:
The vertex can then be found with the coordinates (h, k).
To put the parabola's equation into vertex form, you have to complete the square. Completing the square just means adding the same number to both sides of the equation -- which, remember, doesn't change the value of the equation -- in order to create a perfect square.
Start with the original equation:
Put all of the 
Now we know that we have to add something to both sides in order to create a perfect square:
In this case, we need to add 4 on both sides so that the right-hand side of the equation factors neatly.
Now we factor:
Once we isolate 
Thus, the parabola's vertex can be found at 
Example Question #2 : Completing The Square
Complete the square:
Begin by dividing the equation by 

Square the value in front of the 
Factor the left side of the equation:
Take the square root of both sides and simplify:
Example Question #1623 : High School Math
Use factoring to solve the quadratic equation:
Factor and solve:
Factor like terms:
Combine like terms:
Example Question #91 : Intermediate Single Variable Algebra
Complete the square:
Begin by dividing the equation by 

Square the value in front of the 
Factor the left side of the equation:
Take the square root of both sides and simplify:
Example Question #3 : Completing The Square
Complete the square:
Begin by dividing the equation by 

Square the value in front of the 
Factor the left side of the equation:
Take the square root of both sides and simplify:
Example Question #91 : Intermediate Single Variable Algebra
Solve using the quadratic formula:
Use the quadratic formula to solve:
Example Question #92 : Intermediate Single Variable Algebra
Solve using the quadratic formula:
Use the quadratic formula to solve:
Example Question #93 : Intermediate Single Variable Algebra
Solve using the quadratic formula:
Use the quadratic formula to solve:
Example Question #94 : Intermediate Single Variable Algebra
Solve using the quadratric formula:
Use the quadratic formula to solve:
Example Question #92 : Intermediate Single Variable Algebra
A baseball that is thrown in the air follows a trajectory of 


Between 3 and 3.5 seconds
Between 2 and 2.5 seconds
Between 3.5 and 4 seconds
Between 2.5 and 3 seconds
Between 4 and 4.5 seconds
Between 3 and 3.5 seconds
To solve this, we look at the equation 
Setting the equation equal to 0 we get 
Once in this form, we can use the Quadratic Formula to solve for 
The quadratic formula says that if 

Plugging in our values:
Therefore 

Certified Tutor
All High School Math Resources


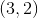


![\small f(x)=a[b(x-h)]^{2}+k](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/108179/gif.latex)




%5E%7B2%7D)