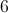The circumference of any circle is 2πr, where r is the radius.

Therefore:

The radius of the smaller circle with a circumference of 4π is 2 (from 2πr = 4π).

The radius of the larger circle with a circumference of 10π is 5 (from 2πr = 10π).

The difference of the two radii is 5-2 = 3.

In order to find the area of the unshaded region, we will need to find the area of the rectangle and then subtract the area of the semicircle. However, to find the area of the rectangle, we will need to find both its length and its width. We can use the circle to find the length of the rectangle, because the length of the rectangle is equal to the diameter of the circle. 

First, we can use the formula for the area of a circle in order to find the circle's radius. When we double the radius, we will have the diameter of the circle and, thus, the length of the rectangle. Then, once we have the rectangle's length, we can find its width because we know the rectangle's perimeter. 

Area of a circle = πr²

Area of a semicircle = (1/2)πr² = 18π

Divide both sides by π, then multiply both sides by 2.

r² = 36

Take the square root.

r = 6.

The radius of the circle is 6, and therefore the diameter is 12. Keep in mind that the diameter of the circle is also equal to the length of the rectangle.

If we call the length of the rectangle l, and we call the width w, we can write the formula for the perimeter as 2l + 2w.

perimeter of rectangle = 2l + 2w

40 = 2(12) + 2w

Subtract 24 from both sides.

16 = 2w

w = 8.

Since the length of the rectangle is 12 and the width is 8, we can now find the area of the rectangle.

Area = l x w = 12(8) = 96.

Finally, to find the area of just the unshaded region, we must subtract the area of the circle, which is 18π, from the area of the rectangle.

area of unshaded region = 96 – 18π.

The answer is 96 – 18π.

The formula for circumference of a circle is , so we can solve for r:

We now know that the hypotenuse of the right triangle's length is 13.5. We can form a right triangle from the unit circle that fits the Pythagorean theorem as such:

Or, in this case:

To find the radius of a circle given the circumference we must first know the equation for the circumference of a circle which is

Then we plug in the circumference into the equation yielding 

We then divide each side by  giving us 

The answer is .

We know that the formula for the area of a circle is πr². Therefore, we must set 36π equal to this formula to solve for the radius of the circle.

36π = πr²

36 = r²

6 = r

Find the total area of the circle, then use the area formula to find the radius.

Area of section A = section B = section C

Area of circle X = A + B + C = 12π+ 12π + 12π = 36π

Area of circle =  where r is the radius of the circle

36π = πr²

36 = r²

√36 = r

6 = r 

To Find your answer, we would use the formula:  C=2πr. We are given that C = 29.5. Thus we can plug in to get  [29.5]=2πr and then multiply 2π to get 29.5=(6.28)r.  Lastly, we divide both sides by 6.28 to get 4.70=r.   (The information given of 22 ounces is useless) 

The formula for circumference is .

Plug in our given information.

Divide both sides by .

Since the formula for the area of a triangle is 

plug in the given area and isolate for . This yields 13. 

When you know the circumference of a circle, you can determine its diameter by dividing the circumference by .  Then, when you have the diameter, you can determine the radius by dividing the diameter by 2.