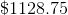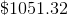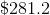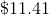All GRE Math Resources
Example Questions
Example Question #1 : How To Use Scientific Notation
The speed of light is approximately 
In scientific notation how many kilometers per hour is the speed of light?
For this problem we need to convert meters into kilometers and seconds into hours. Therefore we get,
Multiplying this out we get
Example Question #11 : Algebra
If one mile is equal to 5,280 feet, how many feet are 100 miles equal to in scientific notation?
100 miles = 528,000 feet. To put a number in scientific notation, we put a decimal point to the right of our first number, giving us 5.28. We then multiply by 10 to whatever power necessary to make our decimal equal the value we are looking for. For 5.28 to equal 528,000 we must multiply by 10^5.
Therefore, our final answer becomes:
Example Question #13 : Algebra
This question requires you to have an understanding of scientific notation. Begin by multiplying the two numbers:
To use scientific notation, the number to the left of the decimal has to be between 1 and 10. In this case, we are looking to move the decimal place until we are left with 9 on the left of the decimal. Count the number of places that the decimal will have to move. In this case, it is five. Therefore:
Note: The notation is raised to a negative power because we moved the decimal from left to right.
Example Question #14 : Algebra
A five-year bond is opened with 

Each year, you can calculate your interest by multiplying the principle (

For two years, it would be:

Therefore, you can solve for a five year period by doing:
Using your calculator, you can expand the 


Example Question #15 : Algebra
Jack has 



First, break the problem into two segments: the amount Jack invests in the high-yield savings, and the amount Jack invests in the simple interest account (10,000 and 5,000 respectively).
Now let's work with the high-yield savings account. $10,000 is invested at an annual rate of 8%, compounded quarterly. We can use the compound interest formula to solve:
Plug in the values given:
Therefore, Jack makes $824.32 off his high-yield savings account. Now let's calculate the other interest:
Add the two together, and we see that Jack makes a total of, 
Example Question #16 : Algebra
If a cash deposit account is opened with 

It is easiest to break this down into steps. For each year, you will multiply by 
After year 1: 
After year 2: 

After year 3: 

Thus, the positive difference of the interest from the last period and the interest from the first period is:
Example Question #11 : Exponents
Quantitative Comparison
Quantity A: x2
Quantity B: x3
Quantity A is greater.
The relationship cannot be determined from the information given.
The two quantities are equal.
Quantity B is greater.
The relationship cannot be determined from the information given.
Let's pick numbers. For quantitative comparisons with exponents, it's good to try 0, a negative number, and a fraction.
0: 02 = 0, 03 = 0, so the two quantities are equal.
–1: (–1)2 = 1, (–1)3 = –1, so Quantity A is greater.
Already we have a contradiction so the answer cannot be determined.
Example Question #1 : Pattern Behaviors In Exponents
If 
We know that the expression must be negative. Therefore one or all of the terms x7, y8 and z10 must be negative; however, even powers always produce positive numbers, so y8 and z10 will both be positive. Odd powers can produce both negative and positive numbers, depending on whether the base term is negative or positive. In this case, x7 must be negative, so x must be negative. Thus, the answer is x < 0.
Example Question #12 : Algebra
Which quantity is the greatest?
Quantity A

Quantity B
Quantity B is greater.
Quantity A is greater.
The two quantities are equal.
The realationship cannot be determined from the information given.
Quantity A is greater.
First rewrite quantity B so that it has the same base as quantity A.



Now we can compare the two quantities.


Example Question #3 : How To Find Patterns In Exponents
Simplify the following:
With problems like this, it is always best to break apart your values into their prime factors. Let's look at the numerator and the denominator separately:
Numerator
Continuing the simplification:
Now, these factors have in common a 
Denominator
This is much simpler:
Now, return to your fraction:
Cancel out the common factors of 
All GRE Math Resources