GMAT Math : Triangles

Study concepts, example questions & explanations for GMAT Math

Create An Account

All GMAT Math Resources

22 Diagnostic Tests 693 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Calculating Whether Acute / Obtuse Triangles Are Congruent

Is it true that $\Delta ABC \cong \Delta DEF$ ?

Suppose you want to answer this question, and you know that $\overline{AB} \cong \overline{DE}$ and $\overline{AC} \cong \overline{DF}$ . Which of the following additional facts would help you to answer this question one way or the other?

Possible Answers:

$\angle C\cong \angle F$

$\overline{AB} \cong \overline{DF}$

None of these statements would be sufficient to answer the question.

$\overline{BC} \cong \overline{EF}$

$\angle B\cong \angle E$

Correct answer:

$\overline{BC} \cong \overline{EF}$

Explanation:

If you know either that $\angle B\cong \angle E$ or $\angle C\cong \angle F$ , you have three congruencies - two sides and a nonincluded angle. This is not enough to establish triangle congruence,

If you know that $\overline{AB} \cong \overline{DF}$ , this, along with the other two statements, establishes that $\overline{AB} \cong \overline{AC} \cong \overline{DE} \cong \overline{DF}$ ; all this proves is that both triangles are isosceles.

If you also know that $\overline{BC} \cong \overline{EF}$ , however, the three statements together make three side congruencies, setting up the Side-Side-Side criterion for triangle congruence.

Report an Error

Example Question #2 : Calculating Whether Acute / Obtuse Triangles Are Congruent

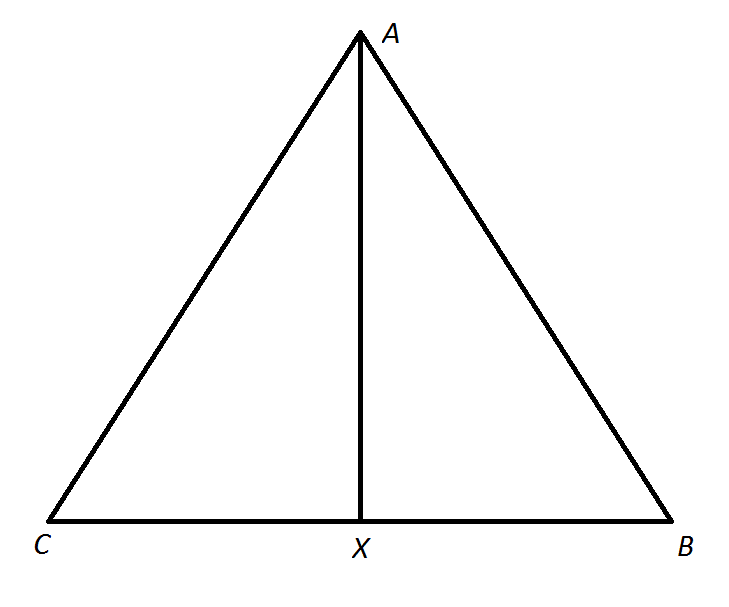
Altitude

Note: Figure NOT drawn to scale

Refer to the above diagram. Which of the following statements is NOT a consequence of the fact that $\Delta ABX \cong \Delta ACX$ ?

Possible Answers:

$\overline{AX}$ is an altitude of $\Delta ABC$

is the midpoint of $\overline{BC}$

$\angle AXC$ is a right angle

$\overline{AX}$ bisects $\angle BAC$

$\Delta ABC$ is an equilateral triangle

Correct answer:

$\Delta ABC$ is an equilateral triangle

Explanation:

We use the congruence of corresponding sides and angles of congruent triangles to prove four of these statements:

$\angle CAX \cong \angle BAX$ , so $\overline{AX}$ bisects $\angle BAC$ .

$\overline{BX} \cong \overline{CX}$ , so is the midpoint of $\overline{BC}$ .

$\angle AXC \cong \angle AXB$ , and, since they form a linear pair, they are supplementary - therefore, they are right angles. Also, by definition, this makes $\overline{AX}$ an altitude.

However, nothing in this congruence proves that $\overline{BC}$ is congruent to the other two sides of $\Delta ABC$ (which are congruent). The correct statement to exclude is that $\Delta ABC$ is equilateral.

Report an Error

Example Question #1 : Calculating An Angle In An Acute / Obtuse Triangle

Which of the following cannot be the measure of a base angle of an isosceles triangle?

Possible Answers:

$80 ^{\circ }$

$50^{\circ }$

$1 ^{\circ }$

$100 ^{\circ }$

Each of the other choices can be the measure of a base angle of an isosceles triangle.

Correct answer:

$100 ^{\circ }$

Explanation:

An isosceles triangle has two congruent angles by the Isosceles Triangle Theorem; these are the base angles. Since at least two angles of any triangle must be acute, a base angle must be acute - that is, it must measure under $90^{\circ }$ . The only choice that does not fit this criterion is $100 ^{\circ }$ , making this the correct choice.

Report an Error

Example Question #81 : Triangles

Let the three interior angles of a triangle measure x, x+30 , and 2x - 10 . Which of the following statements is true about the triangle?

Possible Answers:

The triangle is scalene and acute.

The triangle is scalene and right.

The triangle is scalene and obtuse.

The triangle is isosceles and acute.

The triangle is isosceles and obtuse.

Correct answer:

The triangle is isosceles and acute.

Explanation:

If these are the measures of the interior angles of a triangle, then they total $180^{\circ }$ . Add the expressions, and solve for .

$x +\left ( x + 30 \right ) +\left ( 2x - 10 \right ) = 180$

4x +20 = 180

4x = 160

x = 40

One angle measures $40^{\circ }$ . The others measure:

$x + 30 = 40 + 30 = 70^{\circ }$

$2x-10 = 2 \cdot 40 - 10 = 80 - 10 = 70 ^{\circ }$

All three angles measure less than $90 ^{\circ }$ , so the triangle is acute. Also, there are two congruent angles, so by the converse of the Isosceles Triangle Theorem, two sides are congruent, and the triangle is isosceles.

Report an Error

Example Question #1 : Calculating An Angle In An Acute / Obtuse Triangle

Two angles of an isosceles triangle measure $(x + 20) ^{\circ}$ and $\left ( 100-x\right )^{ \circ}$ . What are the possible values of ?

Possible Answers:

$x = 40 \textrm{ or }x = 80$

x = 40

x = 60

x = 80

$x = 60 \textrm{ or } x = 80$

Correct answer:

x = 40

Explanation:

In an isosceles triangle, at least two angles measure the same. Therefore, one of three things happens:

Case 1: The two given angles have the same measure.

x + 20 = 100 - x

x+ 20 + x = 100 - x + x

2x+ 20 = 100

2x + 20 - 20= 100 -20

2x = 80

$2x \div 2 =80 \div 2$

x = 40

The angle measures are $60 ^{\circ}, 60 ^{\circ}, 60 ^{\circ}$ , making the triangle equianglular and, subsequently, equilateral. An equilateral triangle is considered isosceles, so this is a possible scenario.

Case 2: The third angle has measure $(x + 20) ^{\circ}$ .

Then, since the sum of the angle measures is 180,

$\left (x + 20 \right ) +\left (x +20 \right )+ \left ( 100-x \right ) = 180$

x + 140= 180

x + 140 - 140 = 180- 140

x = 40

as before

Case 3: The third angle has measure $\left ( 100-x\right )^{ \circ}$

$\left (x + 20 \right ) +\left (100-x \right )+ \left ( 100-x \right ) = 180$

220 -x = 180

220 -x -220 = 180-220

-x = -40

x = 40

as before.

Thus, the only possible value of is 40.

Report an Error

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

Two angles of an isosceles triangle measure $\left (x + 30 \right )^{\circ }$ and $\left (x + 36 \right )^{\circ }$ . What are the possible value(s) of ?

Possible Answers:

x= 12

x=6

$x=26 \textrm{ or }x=28$

$x= 12, \; x=26 \textrm{, or }x=28$

$x= 6, \; x=26 \textrm{, or }x=28$

Correct answer:

$x=26 \textrm{ or }x=28$

Explanation:

In an isosceles triangle, at least two angles measure the same. Therefore, one of three things happens:

Case 1: The two given angles have the same measure.

x + 30 = x + 36

x + 30-x = x + 36-x

30=36

This is a false statement, indicating that this situation is impossible.

Case 2: The third angle has measure $\left (x + 30 \right )^{\circ }$ .

Then, since the sum of the angle measures is 180,

$\left (x + 30 \right ) +\left (x + 30 \right )+ \left ( x + 36 \right ) = 180$

3x + 96 = 180

3x =84

x=28

This makes the angle measures $58^{\circ },58^{\circ },64^{\circ }$ , a plausible scenario.

Case 3: the third angle has measure $\left (x + 36 \right )^{\circ }$

Then, since the sum of the angle measures is 180,

$\left (x + 30 \right ) +\left (x + 36 \right )+ \left ( x + 36 \right ) = 180$

3x +102 = 180

3x =78

x=26

This makes the angle measures $56^{\circ }, 62^{\circ }, 62^{\circ }$ , a plausible scenario.

Therefore, either x=26 or x=28

Report an Error

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

$\Delta ABC \sim \Delta XYZ$

$m \angle A = 20 ^{\circ }; m \angle Y = 120 ^{\circ }$

Which of the following is true of $\Delta ABC$ ?

Possible Answers:

$\Delta ABC$ may be scalene or isosceles, but it is acute,

$\Delta ABC$ is isosceles and obtuse.

$\Delta ABC$ is scalene and acute.

$\Delta ABC$ may be scalene or isosceles, but it is obtuse.

$\Delta ABC$ is scalene and obtuse.

Correct answer:

$\Delta ABC$ is scalene and obtuse.

Explanation:

By similarity, $m \angle B = m \angle Y = 120 ^{\circ }$ .

Since measures of the interior angles of a triangle total $180 ^{\circ }$ ,

$m \angle A + m \angle B + m \angle C = 180$

$20 + 120 + m \angle C = 180$

$140 + m \angle C = 180$

$140 + m \angle C -140 = 180-140$

$m \angle C =40^{\circ }$

Since the three angle measures of $\Delta ABC$ are all different, no two sides measure the same; the triangle is scalene. Also, since $m \angle B = 120 ^{\circ } > 90^{\circ }$ , the angle is obtuse, and $\Delta ABC$ is an obtuse triangle.

Report an Error

Example Question #82 : Triangles

Which of the following is true of a triangle with three angles whose measures have an arithmetic mean of $90^{\circ }$ ?

Possible Answers:

The triangle must be obtuse but may be scalene or isosceles.

The triangle must be right but may be scalene or isosceles.

The triangle must be right and isosceles.

The triangle may be right or obtuse but must be scalene.

The triangle cannot exist.

Correct answer:

The triangle cannot exist.

Explanation:

The sum of the measures of three angles of any triangle is 180; therefore, their mean is $180 \div 3 = 60$ , making a triangle with angles whose measures have mean 90 impossible.

Report an Error

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

Two angles of a triangle measure $47 ^{\circ }$ and $23^{\circ }$ . What is the measure of the third angle?

Possible Answers:

$70^{\circ }$

$20^{\circ }$

$160^{\circ }$

$35^{ \circ }$

$110^{\circ }$

Correct answer:

$110^{\circ }$

Explanation:

The sum of the degree measures of the angles of a triangle is 180, so we can subtract the two angle measures from 180 to get the third:

$(180-47-23)^{\circ } = 110^{\circ }$

Report an Error

Example Question #332 : Geometry

The angles of a triangle measure $x^{\circ }, x^{\circ },\left ( x -54 \right ) ^{\circ }$ . Evaluate .

Possible Answers:

x = 96

x = 112

x = 78

x = 84

x = 72

Correct answer:

x = 78

Explanation:

The sum of the measures of the angles of a triangle total $180^{\circ }$ , so we can set up and solve for in the following equation:

x + x + (x-54) = 180

3x-54 = 180

3x-54 + 54 = 180+ 54

3x =234

$3x\div 3 =234 \div 3$

x = 78

Report an Error

All GMAT Math Resources

22 Diagnostic Tests 693 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in Philadelphia, Computer Science Tutors in Houston, GRE Tutors in San Diego, GMAT Tutors in Philadelphia, GMAT Tutors in Atlanta, Math Tutors in New York City, SAT Tutors in Boston, GMAT Tutors in Seattle, Math Tutors in Dallas Fort Worth, GMAT Tutors in Phoenix

Popular Courses & Classes

ACT Courses & Classes in Boston, ACT Courses & Classes in Dallas Fort Worth, LSAT Courses & Classes in Atlanta, Spanish Courses & Classes in Atlanta, SSAT Courses & Classes in Houston, LSAT Courses & Classes in Los Angeles, GMAT Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in Phoenix, SSAT Courses & Classes in San Diego, Spanish Courses & Classes in Phoenix

Popular Test Prep

GRE Test Prep in New York City, SSAT Test Prep in Boston, ISEE Test Prep in Seattle, LSAT Test Prep in Houston, SAT Test Prep in Philadelphia, ISEE Test Prep in San Diego, ACT Test Prep in Los Angeles, GMAT Test Prep in New York City, ISEE Test Prep in Dallas Fort Worth, GRE Test Prep in Washington DC

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

Email address:
Your name:
Feedback:

GMAT Math : Triangles

Study concepts, example questions & explanations for GMAT Math

All GMAT Math Resources

Example Questions

Example Question #1 : Calculating Whether Acute / Obtuse Triangles Are Congruent

Example Question #2 : Calculating Whether Acute / Obtuse Triangles Are Congruent

Example Question #1 : Calculating An Angle In An Acute / Obtuse Triangle

Example Question #81 : Triangles

Example Question #1 : Calculating An Angle In An Acute / Obtuse Triangle

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

Example Question #82 : Triangles

Example Question #2 : Calculating An Angle In An Acute / Obtuse Triangle

Example Question #332 : Geometry

All GMAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details