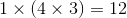All Common Core: 3rd Grade Math Resources
Example Questions
Example Question #21 : Understanding Properties Of Multiplication And The Relationship Between Multiplication And Division
Select the answer that demonstrates the associative property of multiplication for






The associative property of multiplication says that we can group numbers in any order to multiply them and our product, or answer, will be the same.

Example Question #392 : How To Multiply
Select the answer that demonstrates the associative property of multiplication for






The associative property of multiplication says that we can group numbers in any order to multiply them and our product, or answer, will be the same.

Example Question #21 : Understanding Properties Of Multiplication And The Relationship Between Multiplication And Division
Select the answer that demonstrates the associative property of multiplication for






The associative property of multiplication says that we can group numbers in any order to multiply them and our product, or answer, will be the same.

Example Question #391 : How To Multiply
Select the answer that demonstrates the associative property of multiplication for






The associative property of multiplication says that we can group numbers in any order to multiply them and our product, or answer, will be the same.

Example Question #1 : Understand Division As An Unknown Factor Problem: Ccss.Math.Content.3.Oa.B.6
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

Example Question #2 : Understand Division As An Unknown Factor Problem: Ccss.Math.Content.3.Oa.B.6
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

Example Question #23 : Understanding Properties Of Multiplication And The Relationship Between Multiplication And Division
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

Example Question #24 : Understanding Properties Of Multiplication And The Relationship Between Multiplication And Division
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

Example Question #1 : Understand Division As An Unknown Factor Problem: Ccss.Math.Content.3.Oa.B.6
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

Example Question #581 : Common Core Math: Grade 3
Solve 
A factor is a number that is multiplied by another number to produce a given number.
In this case, the unknown factor is the number multiplied by 

All Common Core: 3rd Grade Math Resources