Call Now to Set Up Tutoring:

(888) 888-0446

AP Statistics : Confidence Intervals and Regression

Study concepts, example questions & explanations for AP Statistics

Create An Account

All AP Statistics Resources

4 Diagnostic Tests 140 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find Confidence Intervals For The Slope Of A Least Squares Regression Line

You estimate a regression model with $\beta = 3.4$ $\displaystyle \beta = 3.4$ and $\displaystyle SE = .024$ , where $\beta$ $\displaystyle \beta$ is the beta coefficient and $\displaystyle SE$ is the standard error. Construct 95% confidence intervals for $\beta$ $\displaystyle \beta$ .

Possible Answers:

$\left [ 3.35,3.45 \right ]$ $\displaystyle \left [ 3.35,3.45 \right ]$

$\left [ 3.1,3.45 \right ]$ $\displaystyle \left [ 3.1,3.45 \right ]$

$\left [ 3.4,3.45 \right ]$ $\displaystyle \left [ 3.4,3.45 \right ]$

Correct answer:

$\left [ 3.35,3.45 \right ]$ $\displaystyle \left [ 3.35,3.45 \right ]$

Explanation:

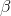
To construct 95% confidence intervals for $\beta$ $\displaystyle \beta$ , we simply take the coefficient and add/subtract $1.96\times the\ standard\ error\ of\ \beta$ $\displaystyle 1.96\times the\ standard\ error\ of\ \beta$ . This is because $\beta$ $\displaystyle \beta$ is assumed to follow a symmetrical distribution (the normal), and 95% of the values in the sampling distribution are contained within 1.96 standard errors of $\beta$ $\displaystyle \beta$ .

Report an Error

Example Question #1 : How To Find Confidence Intervals

Which of the following statements are correct about confidence intervals?

Possible Answers:

The width of a confidence interval increases as the sample size increases and increases as the confidence level decreases.

The width of a confidence interval does not change as the sample size increases and increases as the confidence level increases.

The width of a confidence interval decreases as the sample size increases and increases as the confidence level increases.

The width of a confidence interval increases as the sample size increases and increases as the confidence level increases.

The width of a confidence interval decreases as the sample size increases and increases as the confidence level decreases.

Correct answer:

The width of a confidence interval decreases as the sample size increases and increases as the confidence level increases.

Explanation:

Larger samples give narrower intervals. We are able to estimate a population proportion more precisely with a larger sample size.

As the confidence level increases the width of the confidence interval also increases. A larger confidence level increases the chance that the correct value will be found in the confidence interval. This means that the interval is larger.

Report an Error

Example Question #12 : Confidence Intervals

You are asked to create a $95\%$ $\displaystyle 95\%$ confidence interval with a margin of error no larger than $\displaystyle 3$ while sampling from a normally distributed population with a standard deviation of $\displaystyle 5$ . What is the minimum required sample size?

Possible Answers:

$\displaystyle 11$

$\displaystyle 14$

$\displaystyle 13$

$\displaystyle 10$

$\displaystyle 12$

Correct answer:

$\displaystyle 11$

Explanation:

Keep in mind that the margin of error for a confidence interval based on a normal population is equal to $z^{*}\left(\frac{\sigma}{\sqrt{n}}\right)$ $\displaystyle z^{*}\left(\frac{\sigma}{\sqrt{n}}\right)$ , where $z^{*}$ $\displaystyle z^{*}$ is the $\displaystyle z$ -score corresponding to the desired confidence level.

From the problem, we can tell that $z^{*} = 1.96$ $\displaystyle z^{*} = 1.96$ and $\sigma = 5$ $\displaystyle \sigma = 5$ . We can then solve for $\displaystyle n$ algebraically:

$3 = 1.96\left(\frac{5}{\sqrt{n}}\right)$ $\displaystyle 3 = 1.96\left(\frac{5}{\sqrt{n}}\right)$

$\frac{3}{1.96} = \frac{5}{\sqrt{n}}$ $\displaystyle \frac{3}{1.96} = \frac{5}{\sqrt{n}}$

$\frac{3}{1.96}\cdot \frac{1}{5} = \frac{1}{\sqrt{n}}$ $\displaystyle \frac{3}{1.96}\cdot \frac{1}{5} = \frac{1}{\sqrt{n}}$

$n =\left ( \frac{3}{1.96 \cdot 5} \right )^{-2} = 10.671$ $\displaystyle n =\left ( \frac{3}{1.96 \cdot 5} \right )^{-2} = 10.671$

The minimum sample size is 10.671 $\displaystyle 10.671$ rounded up, which is $\displaystyle 11$ . If you are unsure on problems like these, you can check the margin of error for your answer rounded down and then rounded up (in this case, for n = 10 $\displaystyle n = 10$ and n = 11 $\displaystyle n = 11$ .)

Report an Error

Example Question #61 : Ap Statistics

Jim calculated a $95\%$ $\displaystyle 95\%$ confidence interval for the mean height in inches of boys in his high school. He is not sure how to interpret this interval. Which of the following explains the meaning of $95\%$ $\displaystyle 95\%$ confidence.

Possible Answers:

More information is needed.

There is a $95\%$ $\displaystyle 95\%$ chance that Jim's interval contains the true mean height.

$95\%$ $\displaystyle 95\%$ of boys' heights fall with the interval Jim calculated.

$95\%$ $\displaystyle 95\%$ of all possible sample means fall within Jim's interval.

In the long run, $95\%$ $\displaystyle 95\%$ of all confidence intervals calculated from the same population will contain the true mean height.

Correct answer:

In the long run, $95\%$ $\displaystyle 95\%$ of all confidence intervals calculated from the same population will contain the true mean height.

Explanation:

95% confidence means that the methods Jim uses to calculate his confidence interval give him correct results 95% of the time. It does not mean that there is a 95% chance that the true mean will be inside the interval. It also does not mean that 95% of all heights or possible sample means fall within the interval.

Report an Error

Example Question #2 : Confidence Intervals And Regression

The number of hamburgers served by McGregors per day is normally distributed and has a mean of 3,250 $\displaystyle 3,250$ hamburgers and a standard deviation of 320. $\displaystyle 320.$ Find the range of customers served on the middle $\displaystyle 50$ percent of days.

Possible Answers:

$\displaystyle (2,610, 3,890)$

$\displaystyle (2,930, 3,570)$

$\displaystyle (2,450, 4050)$

$\displaystyle (3,034, 3,466)$

$\displaystyle (2,290, 4,210)$

Correct answer:

$\displaystyle (3,034, 3,466)$

Explanation:

First, find the first quartile of the distribution.

$P(X < Q_{1}) = 0.25 \Rightarrow Q_{1} = \mu - Z_{0.25}\sigma = 3,250 - 0.675(320) = 3,034$ $\displaystyle P(X < Q_{1}) = 0.25 \Rightarrow Q_{1} = \mu - Z_{0.25}\sigma = 3,250 - 0.675(320) = 3,034$

Then, find the third quartile of the distribution.

$P(X < Q_{3}) = 0.75 \Rightarrow Q_{3} = \mu + Z_{0.25}\sigma = 3,250 + 0.675(320) = 3,466$ $\displaystyle P(X < Q_{3}) = 0.75 \Rightarrow Q_{3} = \mu + Z_{0.25}\sigma = 3,250 + 0.675(320) = 3,466$

Report an Error

View AP Statistics Tutors

Robert
Certified Tutor

Daytona State College, Bachelor in Arts, Teaching English as a Second Language (ESL).

View AP Statistics Tutors

Ying
Certified Tutor

Iowa State University, Master of Science, Mathematics.

View AP Statistics Tutors

Ana Maria
Certified Tutor

Florida International University, Bachelor of Science, Mathematics Teacher Education.

All AP Statistics Resources

4 Diagnostic Tests 140 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Computer Science Tutors in Los Angeles, LSAT Tutors in Phoenix, SSAT Tutors in Dallas Fort Worth, MCAT Tutors in Seattle, Reading Tutors in Los Angeles, SSAT Tutors in Los Angeles, Chemistry Tutors in Seattle, Spanish Tutors in New York City, ISEE Tutors in Denver, LSAT Tutors in Houston

Popular Courses & Classes

Spanish Courses & Classes in Dallas Fort Worth, SSAT Courses & Classes in New York City, SAT Courses & Classes in New York City, GMAT Courses & Classes in Boston, GMAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Atlanta, LSAT Courses & Classes in Phoenix, ISEE Courses & Classes in Chicago, MCAT Courses & Classes in Philadelphia

Popular Test Prep

SAT Test Prep in Chicago, GRE Test Prep in Dallas Fort Worth, GMAT Test Prep in Miami, GMAT Test Prep in Boston, GRE Test Prep in Los Angeles, SSAT Test Prep in Chicago, ISEE Test Prep in Boston, GMAT Test Prep in Houston, ACT Test Prep in Chicago, GMAT Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Statistics : Confidence Intervals and Regression

Study concepts, example questions & explanations for AP Statistics

All AP Statistics Resources

Example Questions

Example Question #1 : How To Find Confidence Intervals For The Slope Of A Least Squares Regression Line

Example Question #1 : How To Find Confidence Intervals

Example Question #12 : Confidence Intervals

Example Question #61 : Ap Statistics

Example Question #2 : Confidence Intervals And Regression

All AP Statistics Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: