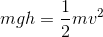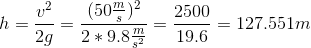All AP Physics C: Mechanics Resources
Example Questions
Example Question #1 : Understanding Gravity
A block with a mass of 

Round to the nearest whole number.
Set the gravitational potential energy and kinetic energy equal to each other and solve for the height.
Mass cancels.
Isolate the height and plug in our values.
Rounding this gives 
Example Question #2 : Gravity
An object of mass 


To find terminal velocity, set the magnitude of the drag force equal to the magnitude of the force of gravity since when these forces are equal and opposite, the object will stop accelerating:
Solve for
Example Question #3 : Gravity
A ship of mass 




The ship's equation of velocity (found by solving the first-order differential equation) is
Substitute 
Example Question #4 : Gravity
A spherical asteroid has a hole drilled through the center as diagrammed below:

Refer to the diagram above. An object that is much smaller than the asteroid is released from rest at the surface of the asteroid, at point a. How do the velocity and acceleration of the object compare at point b at the surface, and point a, located at the center of the asteroid?






Because the gravitational force depends only on the mass beneath the object (which is the gravitational version of Gauss's Law for charge), the acceleration steadily decreases as the object falls, and drops to zero at the center. Nevertheless, the velocity keeps increasing as the object falls, it just does so more slowly.
Example Question #1 : Gravity
With what minimum velocity must a rocket be launched from the surface of the moon in order to not fall back down due to the moon's gravity?
The mass of the moon is 

Relevant equations:
For the rocket to escape the moon's gravity, its minimum total energy is zero. If the total energy is zero, the rocket will have zero final velocity when it is infinitely far from the moon. If total energy is less than zero, the rocket will fall back to the moon's surface. If total energy is greater than zero, the rocket will have some final velocity when it is infinitely far away.
For the minimum energy case as the rocket leaves the surface:
Rearrange energy equation to isolate the velocity term.
Substitute in the given values to solve for the velocity.
Example Question #2 : Gravity
What is the gravitational force of the sun on a 


Relevant equations:
Use the given values to solve for the force.
Example Question #1 : Calculating Gravitational Forces
Two spheres of equal mass are isolated in space, and are separated by a distance 
No change
Newton's law of universal gravitation states:
We can write two equations for the gravity experienced before and after the doubling:
The equation for gravity after the doubling can be simplified:
Because the masses of the spheres remain the same, as does the universal gravitation constant, we can substitute the definition of Fg1 into that equation:
The the gravitational force decreases by a factor of 4 when the distance between the two spheres is doubled.
Example Question #2 : Calculating Gravitational Forces
Two spheres of equal mass are isolated in space. If the mass of one sphere is doubled, by what factor does the gravitational force experienced by the two spheres change?
Newton's law of universal gravitation states that:
We can write two equations representing the force of gravity before and after the doubling of the mass:
The problem gives us 
Substituting these defintions into the second equation:
This equation simplifies to:
Substituting the definition of Fg1, we see:
Thus the gravitational forces doubles when the mass of one object doubles.
Example Question #4 : Gravity
You are riding in an elevator that is accelerating upwards at 

What does the spring scale read when the elevator is descending at constant speed?
When the elevator accelerates upward, we know that an object would appear heavier. The normal force is the sum of all the forces added up, and in this case it is 


When substituting in the values, we get
Solving for 
Since the elevator is descending at constant speed, no additional force is applied, therefore the force that the spring scale reads is only due to gravity, which is calculated by:

Example Question #5 : Gravity
The mass and radius of a planet’s moon are 

With what minimum speed would a bullet have to be fired horizontally near the surface of this moon in order for it to never hit the ground?
(Note: You can treat the moon as a smooth sphere, and assume there’s no atmosphere.)
To do this problem we have to realize that the force of gravity acting on the bullet is equal to the centripetal force. The equations for gravitational force and centripetal force are as follows:
If we set the two equations equal to each other, the small 






If we plug everything in, we get

Certified Tutor
Certified Tutor
All AP Physics C: Mechanics Resources