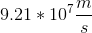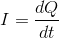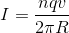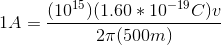Call Now to Set Up Tutoring:

(888) 888-0446

AP Physics C Electricity : Calculating Current

Study concepts, example questions & explanations for AP Physics C Electricity

Create An Account

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Current

A lamp has a 60W $\displaystyle 60W$ bulb. If the house wiring provides 110V $\displaystyle 110V$ to light up that bulb, how much current does the bulb draw?

Possible Answers:

$0.72\ \text{A}$ $\displaystyle 0.72\ \text{A}$

$0.55\ \text{A}$ $\displaystyle 0.55\ \text{A}$

$1.37\ \text{A}$ $\displaystyle 1.37\ \text{A}$

$5.83\ \text{A}$ $\displaystyle 5.83\ \text{A}$

$0.03\ \text{A}$ $\displaystyle 0.03\ \text{A}$

Correct answer:

$0.55\ \text{A}$ $\displaystyle 0.55\ \text{A}$

Explanation:

The formula for power is , P=IV $\displaystyle P=IV$and we are given the following values.

$P=60\ \text{W}$ $\displaystyle P=60\ \text{W}$

$V=110\ \text{V}$ $\displaystyle V=110\ \text{V}$

Solve for the current, $\displaystyle I$.

$I=\frac{P}{V}=\frac{60\ \text{W}}{110\ \text{V}}=0.55\ \text{A}$ $\displaystyle I=\frac{P}{V}=\frac{60\ \text{W}}{110\ \text{V}}=0.55\ \text{A}$

Report an Error

Example Question #13 : Electric Circuits

A particle accelerator with a radius of 500 meters can have up to $10^{15}$ $\displaystyle 10^{15}$ protons circulating within it at once.

$q_{proton}=1.60*10^{-19}C$ $\displaystyle q_{proton}=1.60*10^{-19}C$

How fast must the protons in the accelerator move in order to produce a current of 1A?

Possible Answers:

$9.21*10^{7}\frac{m}{s}$ $\displaystyle 9.21*10^{7}\frac{m}{s}$

$3.00*10^8\frac{m}{s}$ $\displaystyle 3.00*10^8\frac{m}{s}$

$2.04*10^8\frac{m}{s}$ $\displaystyle 2.04*10^8\frac{m}{s}$

$1.95*10^6\frac{m}{s}$ $\displaystyle 1.95*10^6\frac{m}{s}$

$1.96*10^7\frac{m}{s}$ $\displaystyle 1.96*10^7\frac{m}{s}$

Correct answer:

$1.96*10^7\frac{m}{s}$ $\displaystyle 1.96*10^7\frac{m}{s}$

Explanation:

The current produced is the total charge that circulates the particle accelerator per unit time.

$I=\frac{dQ}{dt}$ $\displaystyle I=\frac{dQ}{dt}$

We calculate this by the equation:

$I = \frac{nqv}{2\pi R}$ $\displaystyle I = \frac{nqv}{2\pi R}$

$\displaystyle n$ is the number of protons, $\displaystyle q$ is the charge per proton, $\displaystyle v$ is the velocity of each proton, and $\displaystyle R$ is the radius of the particle accelerator.

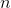
Using the given current, we then solve for the velocity:

$1A = \frac{(10^{15})(1.60*10^{-19}C)v}{2\pi (500m)}$ $\displaystyle 1A = \frac{(10^{15})(1.60*10^{-19}C)v}{2\pi (500m)}$

$v=\frac{(1A)2\pi(500m)}{(10^{15})(1.60*10^{-19}C)}= 1.96*10^7\frac{m}{s}$ $\displaystyle v=\frac{(1A)2\pi(500m)}{(10^{15})(1.60*10^{-19}C)}= 1.96*10^7\frac{m}{s}$

Report an Error

View Tutors

Sundeep
Certified Tutor

Indian Institute of Technology, Engineering in Computer Science, Electrical Engineering. New York University, Masters in Busi...

View Tutors

George
Certified Tutor

Northern Arizona University, Bachelors, Chemistry. Purdue University-Main Campus, PHD, Chemistry.

View Tutors

Robert
Certified Tutor

Rensselaer Polytechnic Institute, Bachelors, Electrical Engineering. Brooklyn Polytechnic, Masters, Computer Science.

All AP Physics C Electricity Resources

1 Diagnostic Test 46 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Reading Tutors in Philadelphia, ISEE Tutors in Chicago, GMAT Tutors in Boston, Algebra Tutors in Denver, ISEE Tutors in Washington DC, GMAT Tutors in San Francisco-Bay Area, French Tutors in Phoenix, GMAT Tutors in San Diego, GRE Tutors in San Diego, GMAT Tutors in Los Angeles

Popular Courses & Classes

SAT Courses & Classes in San Diego, GMAT Courses & Classes in Atlanta, SAT Courses & Classes in Seattle, GMAT Courses & Classes in Phoenix, ISEE Courses & Classes in San Francisco-Bay Area, Spanish Courses & Classes in Boston, GRE Courses & Classes in Washington DC, ISEE Courses & Classes in Denver, ISEE Courses & Classes in Seattle, MCAT Courses & Classes in Chicago

Popular Test Prep

ACT Test Prep in Washington DC, LSAT Test Prep in Seattle, ISEE Test Prep in Seattle, MCAT Test Prep in Philadelphia, MCAT Test Prep in Houston, LSAT Test Prep in Chicago, LSAT Test Prep in Dallas Fort Worth, GMAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Seattle, GMAT Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Physics C Electricity : Calculating Current

Study concepts, example questions & explanations for AP Physics C Electricity

All AP Physics C Electricity Resources

Example Questions

Example Question #1 : Current

Example Question #13 : Electric Circuits

All AP Physics C Electricity Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: