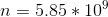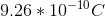All AP Physics 2 Resources
Example Questions
Example Question #1 : Circuit Components

Four arrangements of capacitors are pictured. Each has an equivalent capacitance. Rank these four arrangements from highest equivalent capacitance to lowest. Assume that all capacitors are identical.
Let's go through all of them and find the equivalent capacitance.
(A) This is just
(B) There are two capacitors in series, so this is
(C) These capacitors are in parallel, so
(D) These are a combination of series and parallel. Two are in series and they are in parallel with a third,
So, ranking them we get
Example Question #1 : Capacitors And Capacitance
If the maximum amount of charge held by a capacitor at a voltage of 12V is 36C, what is the capacitance of this capacitor?
In this question, we're told the maximum amount of charge that a capacitor can hold at a given voltage. We're then asked to determine the capacitance. To do this, we'll need to use the expression for capacitance.
Plug in the values given to us in the question stem:
Example Question #1 : Capacitors And Capacitance
Imagine a capacitor with a magnitude of charge Q on either plate. This capacitor has area A, separation distance D, and is connected to a battery of voltage V. If some external agent pulls the capacitor apart such that D doubles, did the voltage difference between the plates increase, decrease or stay the same?
Stay the same
Decrease
Increase
It depends on the voltage of the battery
Stay the same
The fact that the system is still connected to the battery indicate a constant V so regardless what happens to the capacitor, V stays fixed.
Example Question #2 : Capacitors And Capacitance
Imagine a capacitor with a magnitude of charge Q on either plate. This capacitor has area A, separation distance D, and is connected to a battery of voltage V. If some external agent pulls the capacitor apart such that D doubles, did the charge on each plate increase, decrease or stay the same?
Stays constant
The charge is zero
Decrease
Increase
Decrease
Relevant equations:
Plug the second equation into the first:
Considering all the variables in the numerator are held fixed for this problem, we see that increasing D will decrease the charge stored on each plate.
Example Question #2 : Capacitors And Capacitance
Imagine a capacitor with a magnitude of charge Q on either plate. This capacitor has area A, separation distance D, and is not connected to a battery of voltage V. If some external agent pulls the capacitor apart such that D doubles, did the charge on each plate increase, decrease or stay the same?
Increase
Decrease
Stays constant
We need to know the capacitance of the capacitor
Stays constant
The charge has no where to go. Without the battery connected, the charge has no physical avenue on or off the plates.
Example Question #11 : Circuit Components
Imagine a capacitor with a magnitude of charge Q on either plate. This capacitor has area A, separation distance D, and is connected to a battery of voltage V. If some external agent pulls the capacitor apart such that D doubles, how did the capacitance change? Also, do we need to use the information provided about the battery?
Decrease, yes
Increase, no
Decrease, no
Increase, yes
Decrease, no
As D increases, C will decrease
Does the battery matter? No. Capacitance is a "geometric" quantity. This means that it can be determined solely by physical parameters like the area and separation distance. The charge on the plates, voltage difference, electric field and any other quantity you could think of does not influence the capacitance other than A or D.
Example Question #12 : Capacitors And Capacitance
Consider the given diagram. If 

None of these
The voltage rise through the source must be the same as the drop through the capacitor.
The voltage drop across the capacitor is the equal to the electric field multiplied by the distance.
Combine equations and find the electric field:
Convert mm to m and plugg in values:
Use the electric field in a capacitor equation:
Convert 

The magnitude of total charge on the positive plate is equal to the total charge on the negative plate, so to find the number of excess elections:
Example Question #283 : Electricity And Magnetism

Consider the given diagram. If 


The voltage rise through the source must be the same as the drop through the capacitor.
The voltage drop across the capacitor is the equal to the electric field multiplied by the distance.
Combine equations and solve for the electric field:
Convert mm to m and plug in values:
Using the electric field in a capacitor equation:
Rearrange to solve for the charge:
Convert 

Example Question #14 : Capacitors And Capacitance
Suppose I have a uniform electric field within a parallel plate capacitor with field strength of 
Suppose the capacitor's plates are 


What is the voltage difference across the capacitor?
Voltage difference 



For this problem,
Example Question #15 : Capacitors And Capacitance
Suppose I have a uniform electric field within a parallel plate capacitor with field strength of 
Suppose the capacitor's plates are 


Determine the capacitance of this system given that the space in between is a vacuum, and that the permittivity of empty space 

The formula for capacitance 

Where 



To determine 

Putting it all together,
Certified Tutor
All AP Physics 2 Resources