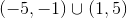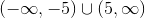To determine the values at which the function has a relative maximum, we must determine the intervals on which the function's first derivative changes from positive to negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero. The first derivative can never equal zero, so the function can have no relative maxima. 

To determine the x-values at which the function has a relative maximum, we must determine the intervals on which the function's first derivative changes from positive to negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, on the third interval, the first derivative is positive, on the fourth interval, the first derivative is negative, and on the fifth interval, the first derivative is positive. Thus, the function has relative maxima at . 

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, , 

Next, we must find the critical values, at which the first derivative is equal to zero:

Note the critical values are entirely within the given interval of x in the problem statement.

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, on the third interval, the first derivative is positive, and on the fourth interval, the first derivative is negative. Thus, the intervals on which the function is increasing are . 

To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero. The first derivative function can never equal zero, so the function is never decreasing. 

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, on the third interval, the first derivative is positive, on the fourth interval, the first derivative is negative, and on the fifth interval, the first derivative is positive. Therefore, the function is increasing on the intervals . 

To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is negative, and on the second interval, the first derivative is positive. We know, therefore, that the function is decreasing on the first interval, . 

To determine the values at which the function has a relative minimum, we must determine the intervals on which the function's first derivative changes from negative to positive. 

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, and on the third interval, the first derivative is positive. Thus, the x value at which the function has a relative minimum is , because here the function's first derivative changed from negative to positive.

Note that the derivative cannot be negative, because that would mean  is decreasing. If  decreases from the point  and moves to , we went to the right so , but we decreased, so we know. This goes against the given conditions, as if   we must have 

Carrying out the same analysis but with a positive derivative, we see that if , we have  which is acceptable by our given conditions.

Lastly, if the derivative is 0, then the function value didn't change. Thus, this would mean moving from  to  where . And, of course, if , it's also true that , so we have  and  as desired.

Thus, as long as we're in , our derivative can be positive or 0, but not negative.

Explain whether f(x) is increasing or decreasing when , and choose the correct explanation for its behavior.

To determine increasing or decreasing behavior, we need to know the sign of the first derivative at the given point. To do this, we will first find the first derivative, and then plug in our given point. This will tell us the slope of the tangent line to the original function at a particular point. Let's begin by finding our derivative.

To find our derivative, we need to recall two rules.

And

Using these two rules, we can find the derivative of f(x).

Our first term can be derived using our first rule. The derivative of e to the x is just e to the x.

This means that our first term will remain 16e to x.

For our other three terms, we follow the second rule. We will decrease each term's exponent by 1, and then multiply the coefficient by the old exponent.

Notice that the 13 will drop out. It is a constant term, and as such when we multiply it by it's original exponent (0) it wil be reduced to zero as well.

Clean up the above to get:

Now, we need to plug in 5 for x and see what our sign is.

Now, all that really matters for this question is the ultimate sign. Can you guess what it will be without calculating?

It is hugely positive! This makes sense, because we are raising two positive numbers to the fifth power. The only negative number we have comes from a linear term, so there is no way it will overcome the large positive terms.

So, our first derivative is positive at x=5. This means that f(x) is increasing when , because 

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, , 

Next, we must find the critical values, at which the first derivative is equal to zero:

Note that the critical values are bounded by the interval in the problem statement.

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, on the third interval, the first derivative is positive, and on the fourth interval, the first derivative is negative. So, the function is increasing on .