All AP Calculus AB Resources
Example Questions
Example Question #31 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find f'(x) for the following f(x).
Since the function f(x) is a quotient of two separate functions, sin(x) + cos(x) and e^x, use the quotient rule to take the derivative.
Recall the quotient rule for two functions u and v:
Let's define u and v for this function as follows:
Take the derivative of u and v:
Now that we've defined u, u', v, and v' we can substitute them into the quotient rule formula.
Factor out e^x from to the top and bottom and simplify into the final form:
Example Question #32 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find f'(x) for the following f(x).
Since the function f(x) is a product of two functions, 5x^4 and sec(x), use the product rule to take this derivative.
Recall the product rule where u and v are two separate functions:
Let's define u and v as follows:
Take the derivative of u and v:
Now that we've found values of u, u', v, and v' we can substitute them into the product rule formula for the final answer.
Example Question #33 : Derivative Rules For Sums, Products, And Quotients Of Functions
Differentiate the function:
First notice that the function is a product of two functions 

_______________________________________________________________
The second term will require the chain rule. Recall that the derivative for a radical function-of-a-function 
_______________________________________________________________
Example Question #34 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative
The derivative can be computed using the quotient rule:
Example Question #35 : Derivative Rules For Sums, Products, And Quotients Of Functions
Differentiate
The function is a product of the functions 

The derivative in the first term is 
The derivative in the second term required the use of the chain rule. First we write the derivative of 





Therefore,
Example Question #36 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative of the following equation:
Given that there are 2 terms in the numerator and only one in the denominator, one can split up the equation into 2 separate derivatives:

Now we simplify these, and proceed to solve:
Example Question #37 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative of the following equation:
None of the other answers.
Because this problem contains two functions multiplied together that can't be simplified any further, it calls for the product rule, which states that![]()
By applying this rule to the equation

we get
Example Question #38 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative of the following function:
None of the other answers
Because we are dealing with a quotient that cannot be simplified, we use the quotient rule, which states that if


By observing the given equation

we can see that

and

Therefore, the derivative is

Example Question #39 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative of the following equation:
None of the other answers.
Because we are differentiating a quotient that cannot be simplified, we must use the quotient rule, which states that if

then

By observing the given equation,

we see that in this case,

and

Given this information, the quotient rule tells us that

Example Question #40 : Derivative Rules For Sums, Products, And Quotients Of Functions
Find the derivative of the following equation:
None of the other answers.
This problem is a quotient rule inside of a chain rule. First, let's look at the chain rule:
![]() .
.
Given this, we can deduce that since


and

By plugging these into the chain rule formula, we get

To find the derivative of the
second term, we must use the quotient rule, which states that the the derivative
of a quotient is ((denominator)(derivative of numerator)-(numerator)(derivative
of denominator))/(denominator squared). Using this rules we find that

By plugging this back in, we find the final derivative to be
All AP Calculus AB Resources





























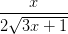



















![y = \frac{f(x)}{g(x)}\: \: \: \: \: \:\ \Rightarrow \: \: \: \: \: \:\frac{dy}{dx}=\frac{g(x)f'(x)-g'(x)f(x)}{[g(x)]^2 }](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/933467/gif.latex)
![\frac{dy}{dx}=\frac{\sqrt{x^2+4}\left[\frac{d}{dx}(2x-1) \right ]-\left[\frac{d}{dx}\sqrt{x^2+4} \right ](2x-1)}{x^2+4}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/927897/gif.latex)
















































