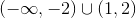To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rule:

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

On the first interval, the first derivative is negative, while on the remaining intervals, the first derivative is positive. Thus, the function is decreasing on the first interval, . 

To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rule:

Next, we must find the critical values, at which the first derivative is equal to zero:

The square root of a negative number is not valid for a critical value, so we only have one critical value for this function.

Using the critical value, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

On the first interval, the first derivative is positive, and on the second interval, the first derivative is positive as well. The function is therefore never decreasing because the first derivative is never negative. 

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, ,  

Next, we must find the critical values, at which the first derivative is equal to zero:

Note that we stopped writing critical values based on the given interval in the problem statement.

Using the critical values, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is negative, while on the second interval, the first derivative is positive. Thus, the function is increasing on the second interval, .

To determine the local maxima of the function, we must determine the points at which the function's first derivative changes from positive to negative.

The first derivative of the function is

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical value as an endpoint, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, and on the second interval, the first derivative is positive as well. There is no sign change of the first derivative, thus there are no local maxima (the function is always increasing).

To determine the local minima of the function, we must determine the points at which the function's first derivative changes from negative to positive.

The first derivative of the function is

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical value as an endpoint, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is negative, and on the second interval, the first derivative is positive. There is a sign change from negative to positive of the first derivative at , thus a local minima exists here. 

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals on which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, and on the third interval, the first derivative is positive. Thus, the function is increasing on the first and third intervals, .

To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

(Note that the method of completing the square was shown for solving for the critical values. One could use the quadratic formula as well.)

Using the critical values, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative. and on the third interval, the first derivative is positive. Thus, the interval on which the first derivative is negative is the interval where the function is decreasing, .

To determine the intervals on which the function is decreasing, we must determine the intervals on which the function's first derivative is negative.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Note that factoring by grouping was used to find the critical values.

Using the critical values, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is negative, on the second interval, the first derivative is positive, on the third interval, the first derivative is negative, and on the fourth interval, the first derivative is positive. The intervals on which the function is decreasing are the intervals on which the first derivative was negative, .

To determine the intervals on which the function is increasing, we must determine the intervals on which the function's first derivative is positive.

The first derivative of the function is equal to

and was found using the following rules:

, 

Next, we must find the critical values, at which the first derivative is equal to zero:

Using the critical values, we now create intervals over which to evaluate the sign of the first derivative:

Notice how at the bounds of the intervals, the first derivative is neither positive nor negative.

Evaluating the sign simply by plugging in any value on the given interval into the first derivative function, we find that on the first interval, the first derivative is positive, on the second interval, the first derivative is negative, and on the third interval, the first derivative is positive. The function is therefore increasing on two intervals, , on which we just showed the first derivative was positive.