All AP Calculus AB Resources
Example Questions
Example Question #47 : Chain Rule And Implicit Differentiation
Find the derivative of the following function:

Here, we need to take the derivative of a square root function first. Then, we will multiply that by the derivative of the function under the square root.
Remember: 
Now, let's make use of our trigonometric identities:
Therefore, we can simplify our answer:
Note, you could have used this trigonometric identity from the very beginning, making the problem much easier!
Example Question #48 : Chain Rule And Implicit Differentiation
Find
For implicit differentiation, we need to take a derivative from left to right of our function. The only difference is that any time we take the derivative of a 


Example Question #49 : Chain Rule And Implicit Differentiation
Find 
For implicit differentiation, we need to take the derivative of every term, including numbers. Whenever we take a derivative of a function of 

In the last step, I canceled all of the negative signs.
Example Question #141 : Computation Of The Derivative
Determine the derivative of
This is a pure problem on understanding how chain rules work for derivatives.
First thing we need to remember is that the derivative of 

When we are taking the derivative of 

![[\tan(x^2)]^2](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/22906/gif.latex)
This way, the derivative will become 
which is 
Example Question #231 : Derivatives
Find 
To find 
Taking 
using the following rules:


Note that for every derivative of a function with y, the additional term 

Using algebra to rearrange, we get
Example Question #232 : Derivatives
Find the derivative:
The derivative of the function is equal to
and was found using the following rules:



Before simplification, the derivative we get is
Note that the square root, the exponential, and the tangent function all utilize chain rule when taking their derivatives.
Example Question #233 : Derivatives
Find the first derivative of the following function:
The derivative of the function is equal to
and was found using the following rules:



Note that the first rule - the chain rule - was used three times for the function: the cosine, contained the exponential which itself was raised to a function. (Note that sometimes the exponential rule is written as 
Example Question #234 : Derivatives
A reaction is modeled by the following equation:
where 
What is 
The derivative of the function is equal to
and was found using the following rules:


The chain rule was used for the function contained in the exponential function (or, as written as a rule, 
Example Question #235 : Derivatives
For the equation, 

The equation given is not written and 



"With respect to 



First we must differentiate both sides with respect to 
We have multiple terms on the left hand side, so we will differentiate each term individually.
For the first term, ![\frac{\mathrm{d} }{\mathrm{d} x}[y^2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967672/gif.latex)

The blue part is the power rule. The red is from the chain rule. The red part is the derivative of y with respect to x, which is currently unknown. Remember that y is some unknown function of x, whose derivative is also unknown. We can only write 
Now we find the second term's derivative,
This is a product rule. To help with the product rule, the two pieces are color coded. Remember that the power rule is
Applying this, we get
Simplifying gives us
For the third term, 

remember that the derivative of 


The right hand side of the equation is a constant, so its derivative is zero.
Assembling all the parts back together, we have
Now that we have differentiated the equation, we need to algebraically solve for 
First, we should move all terms with a 

Then we will will factor out the common factor of 
Then, to isolate 
![[2y + 2x^2 + 5xy^{-2}]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/969713/gif.latex)
Now we need to simplify and get rid of the negative exponents. To do this, we can simply multiply the numerator and denominator by 
Which is the correct answer.
Example Question #236 : Derivatives
Given the function 
Given the function 
where 




All AP Calculus AB Resources


































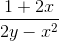



































![\frac{\mathrm{d} }{\mathrm{d} x}[y^2 + 2x^2y - \frac{5x}{y}] = \frac{\mathrm{d} }{\mathrm{d} x}[2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967670/gif.latex)
![\frac{\mathrm{d} }{\mathrm{d} x}[y^2] + \frac{\mathrm{d} }{\mathrm{d} x}[2x^2y] + \frac{\mathrm{d} }{\mathrm{d} x}[\frac{-5x}{y}] = \frac{\mathrm{d} }{\mathrm{d} x}[2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/969699/gif.latex)
![\frac{\mathrm{d} }{\mathrm{d} x}[y^2]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967674/gif.latex)
![{\color{Blue} 2[y]^{2-1}}{\color{DarkRed} (\frac{\mathrm{d} y}{\mathrm{d} x})}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967675/gif.latex)

![\frac{\mathrm{d} }{\mathrm{d} x}[{\color{Blue} 2x^2}{\color{DarkRed} y}]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967678/gif.latex)
![\frac{\mathrm{d} }{\mathrm{d} x}[{\color{Blue} f(x)}\cdot{\color{DarkRed} g(x)}] ={\color{Blue} f(x)}\cdot {\color{DarkRed} g'(x)} + {\color{Blue} f'(x)} \cdot {\color{DarkRed} g(x)}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/967679/gif.latex)


![\frac{\mathrm{d} }{\mathrm{d} x}[{\color{Blue} -5x}{\color{DarkRed} y^{-1}}]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/969702/gif.latex)




![\frac{\mathrm{d} y}{\mathrm{d} x}[2y + 2x^2 + 5xy^{-2}] = -4xy + 5y^{-1}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/969711/gif.latex)



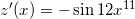



![\small [f(g(x))]'=f'(g(x))g'(x)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/970854/gif.latex)




