All AP Calculus AB Resources
Example Questions
Example Question #2 : Calculus I — Derivatives
Consider the function
Find the minimum of the function on the interval ![\left [ 0,1 \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/90588/gif.latex)
To find potential minima of the function, take the first derivative of 
Set the derivative to 0:
We solve for 

We can double check that 
which means the function is concave up, so that the point we found is a minimum.
Example Question #1 : Techniques Of Antidifferentiation
Find the x coordinate of the minimum of 
![\left [ -2, 2\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/106125/gif.latex)
First, find the derivative, which is:
Set that equal to 0 to find your critical points:
Evaluate f at each critical point and endpoint over [-2, 2]:
The least of those numbers is the minimum. Because f(-2)=-32, that is the minimum.
Example Question #1 : Antiderivatives Following Directly From Derivatives Of Basic Functions
What is the local minimum of 

There is no local minimum in that range.
The y-value is constant throughout that range.
To find the maximum, we need to look at the first derivative.
To find the first derivative, we can use the power rule. To do that, we lower the exponent on the variables by one and multiply by the original exponent.
We're going to treat 

Notice that 
When looking at the first derivative, remember that if the output of this equation is positive, the original function is increasing. If the derivative is negative, then the function is decreasing.
Because we want the MINIMUM, we want to see where the derivative changes from negative to positive.
Notice that 


Example Question #1 : Techniques Of Antidifferentiation
Integrate,


Integrate
1) Apply the sum rule for integration,
2) Integrate each individual term and include a constant of integration,
Further Discussion
Since indefinite integration is essentially a reverse process of differentiation, check your result by computing its' derivative.
This is the same function we integrated, which confirms our result. Also, because the derivative of a constant is always zero, we must include "C" in our result since any constant added to any function will produce the same derivative.
Example Question #5 : Techniques Of Antidifferentiation
Example Question #1 : Antiderivatives Following Directly From Derivatives Of Basic Functions
Example Question #2 : Antiderivatives Following Directly From Derivatives Of Basic Functions
Example Question #3 : Antiderivatives Following Directly From Derivatives Of Basic Functions
Example Question #4 : Antiderivatives Following Directly From Derivatives Of Basic Functions
Example Question #5 : Antiderivatives Following Directly From Derivatives Of Basic Functions
Certified Tutor
Certified Tutor
All AP Calculus AB Resources


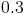
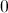

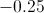






















![\int[x^2 +\sin(x)-3]dx](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/749356/gif.latex)




![\int[x^2 +\sin(x)-3]dx](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/749357/gif.latex)








![\begin{align*}&\text{In performing an integral, familiarity with derivative rules}\\&\text{can prove useful. After all, an integral is essentially the}\\&\text{opposite operation of a derivative. Consider how in a derivative}\\&\text{we’d use the chain rule to multiply the derivative of the “inside”}\\&\text{of a function by the derivative of the outside? Integrals, in}\\&\text{similar fashion, entail a division.}\\&\text{Utilizing integral rules:}\\&\int[cos(ax)]=\frac{sin(ax)}{a}\\&\int_{8}^{11.5}(\frac{(27cos(x + 1))}{8})dx=\frac{(27sin(x + 1))}{8}|_{8}^{11.5}\\&\text{Now we just subtract the function value at the lower bound from that of the upper bound:}\\&\int_{8}^{11.5}(\frac{(27cos(x + 1))}{8})dx=\frac{(27sin((11.5) + 1))}{8}-(\frac{(27sin((8) + 1))}{8})\\&\int_{8}^{11.5}(\frac{(27cos(x + 1))}{8})dx=-1.61\end{align*}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/931547/gif.latex)











![\begin{align*}&\text{In performing an integral, familiarity with derivative rules}\\&\text{can prove useful. After all, an integral is essentially the}\\&\text{opposite operation of a derivative. Consider how in a derivative}\\&\text{we’d use the chain rule to multiply the derivative of the “inside”}\\&\text{of a function by the derivative of the outside? Integrals, in}\\&\text{similar fashion, entail a division.}\\&\text{Utilizing integral rules:}\\&\int[b^{ax}]=\frac{b^{ax}}{aln(b)}\\&\int_{-6}^{-3}(\frac{(10\cdot 2^{(\frac{x}{4})})}{7})dx=\frac{(40\cdot 2^{(\frac{x}{4})})}{(7ln(2))}|_{-6}^{-3}\\&\text{Now we just subtract the function value at the lower bound from that of the upper bound:}\\&\int_{-6}^{-3}(\frac{(10\cdot 2^{(\frac{x}{4})})}{7})dx=\frac{(40\cdot 2^{(\frac{(-3)}{4})})}{(7ln(2))}-(\frac{(40\cdot 2^{(\frac{(-6)}{4})})}{(7ln(2))})\\&\int_{-6}^{-3}(\frac{(10\cdot 2^{(\frac{x}{4})})}{7})dx=1.99\end{align*}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/931641/gif.latex)

















![\begin{align*}&\text{In performing an integral, familiarity with derivative rules}\\&\text{can prove useful. After all, an integral is essentially the}\\&\text{opposite operation of a derivative. Consider how in a derivative}\\&\text{we’d use the chain rule to multiply the derivative of the “inside”}\\&\text{of a function by the derivative of the outside? Integrals, in}\\&\text{similar fashion, entail a division.}\\&\text{Utilizing integral rules:}\\&\int[cos(ax)]=\frac{sin(ax)}{a}\\&\int_{-16}^{-14}(\frac{(9cos(x + 2))}{11})dx=\frac{(9sin(x + 2))}{11}|_{-16}^{-14}\\&\text{Now we just subtract the function value at the lower bound from that of the upper bound:}\\&\int_{-16}^{-14}(\frac{(9cos(x + 2))}{11})dx=\frac{(9sin((-14) + 2))}{11}-(\frac{(9sin((-16) + 2))}{11})\\&\int_{-16}^{-14}(\frac{(9cos(x + 2))}{11})dx=1.25\end{align*}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/931631/gif.latex)



