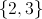All Algebra II Resources
Example Questions
Example Question #1 : Solving Equations And Inequallities
Give all real solutions of the following equation:
By substituting 

We are looking to factor the quadratic expression as 


Substitute back:
The first factor cannot be factored further. The second factor, however, can itself be factored as the difference of squares:
Set each factor to zero and solve:

Since no real number squared is equal to a negative number, no real solution presents itself here.
The solution set is 
Example Question #1 : Cube Roots
Which of the following displays the full real-number solution set for 
Rewriting the equation as 






Example Question #1 : Solving Non Quadratic Polynomials
Factor by grouping.
The first step is to determine if all of the terms have a greatest common factor (GCF). Since a GCF does not exist, we can move onto the next step.
Create smaller groups within the expression. This is typically done by grouping the first two terms and the last two terms.
Factor out the GCF from each group:
At this point, you can see that the terms inside the parentheses are identical, which means you are on the right track!
Since there is a GCF of (5x+1), we can rewrite the expression like this:
And that is your answer! You can always check your factoring by FOILing your answer and checking it against the original expression.
Example Question #2 : Solving Non Quadratic Polynomials
Factor completely:
The polynomial is prime.
This can be most easily solved by setting 



The quadratic factors do not fit any factoring pattern and are prime, so this is as far as the polynomial can be factored.
Example Question #4 : Solving Non Quadratic Polynomials
If 



To find 

We can now use this value to find 
Our final answer is therefore
Example Question #1 : Solving Non Quadratic Polynomials
Factor:
Using the difference of cubes formula:
Find x and y:
Plug into the formula:
Which Gives:
And cannot be factored more so the above is your final answer.
Example Question #2 : Solving Non Quadratic Polynomials
Factor 
First, we can factor a 
Now we can make a clever substitution. If we make 
This makes it much easier to see how we can factor (difference of squares):
The last thing we need to do is substitute 


Substituting back in gives us a result of:
Example Question #5201 : Algebra Ii
Simplify:
Use the difference of squares to factor out the numerator.
The term 

Replace the fraction.
Simplify the top and bottom.
The answer is:
All Algebra II Resources