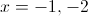Call Now to Set Up Tutoring:

(888) 888-0446

Algebra II : Understanding Rational Expressions

Study concepts, example questions & explanations for Algebra II

Create An Account

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Understanding Rational Expressions

Which of the following fractions is NOT equivalent to $- \frac{x-5}{2x + 3}$ ?

Possible Answers:

$\frac{x+5}{2x + 3}$

$\frac{x-5}{-2x-3}$

$\frac{-x+5}{2x+3}$

$\frac{x-5}{\left -(2x+3 \right )}$

$\frac{\left -(x-5 \right )}{2x+3}$

Correct answer:

$\frac{x+5}{2x + 3}$

Explanation:

We know that $-\frac{a}{b}$ is equivalent to $\frac{-a}{b}$ or $\frac{a}{-b}$ .

By this property, there is no way to get $\frac{x+5}{2x+3}$ from $-\frac{x-5}{2x+3}$ .

Therefore the correct answer is $\frac{x+5}{2x+3}$ .

Report an Error

Example Question #2 : Understanding Rational Expressions

Determine the domain of

$\frac{x+1}{x-1}$

Possible Answers:

x=1

$x\neq1$

$x\neq-1$

All real numbers

Correct answer:

$x\neq1$

Explanation:

Because the denominator cannot be zero, the domain is all other numbers except for 1, or

$x\neq1$

Report an Error

Example Question #2 : Definition Of Rational Expression

Simplify:

$\frac{x^2-1}{x^2+4x+2}\div\frac{x^2+2x+1}{x^2+3x+2}$

Possible Answers:

x-1

$\frac{1}{\frac{1}{x+1}}$

$\frac{x+2}{x-1}$

$\frac{x+2}{x+1}$

$\frac{x-1}{x+2}$

Correct answer:

$\frac{x-1}{x+2}$

Explanation:

This problem is a lot simpler if we factor all the expressions involved before proceeding:

$\frac{x^2-1}{x^2+4x+2}\div\frac{x^2+2x+1}{x^2+3x+2} \vspace{5mm}\\ = \frac{(x+1)(x-1)}{(x+2)(x+2)}\div\frac{(x+1)(x+1)}{(x+2)(x+1)}$

Next let's remember how we divide one fraction by another—by multiplying by the reciprocal:

$\frac{(x+1)(x-1)}{(x+2)(x+2)}\div\frac{(x+1)(x+1)}{(x+2)(x+1)} \vspace{5mm}\\ = \frac{(x+1)(x-1)}{(x+2)(x+2)}\times\frac{(x+2)(x+1)}{(x+1)(x+1)}$

In this form, we can see that a lot of terms are going to start canceling with each other. All that we're left with is just $\frac{x-1}{x+2}$ .

Report an Error

Example Question #4 : Rational Expressions

Which of the following is the best definition of a rational expression?

Possible Answers:

$\textup{The ratio of any two real numbers.}$

$\textup{The ratio of any two imaginary numbers.}$

$\textup{The ratio of two fractions that may have a zero denominator.}$

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

$\textup{The ratio of two polynomials that must be different from each other.}$

Correct answer:

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

Explanation:

The rational expression is a ratio of two polynomials.

$\frac{A(x)}{B(x)}$

The denominator cannot be zero.

An example of a rational expression is:

$\frac{x^2+x-5}{x+8}$

The answer is:

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

Report an Error

Example Question #3 : Understanding Rational Expressions

Find the values of which will make the given rational expression undefined:

$\frac{x+5}{\left ( x-1 \right )\left ( x+2 \right )}$

Possible Answers:

x = -5, +2

x = -5, 1

x = -1, 2

x = -1, -2

x = 1, -2

Correct answer:

x = 1, -2

Explanation:

If $\left ( x-1 \right ) = 0$ or $\left ( x+2 \right ) =0$ , the denominator is 0, which makes the expression undefined.

This happens when x = 1 or when x = -2.

Therefore the correct answer is x = 1, -2 .

Report an Error

Example Question #2 : Properties Of Fractions

Simply the expression:

$\frac{4}{x+6}+ \frac{9}{x+4}$

Possible Answers:

$\frac{18x+60}{x^{2}-10x+28}$

$\frac{15x+70}{x^{2}+4x+18}$

$\frac{12x+40}{x^{2}-4x+10}$

$\frac{13x+70}{x^{2}+10x+24}$

$\frac{14x+20}{x^{2}+9x+15}$

Correct answer:

$\frac{13x+70}{x^{2}+10x+24}$

Explanation:

In order to simplify the expression $\frac{4}{x+6}+ \frac{9}{x+4}$ , we need to ensure that both terms have the same denominator. In order to do so, find the Least Common Denominator (LCD) for both terms and simplify the expression accordingly:

$\frac{4(x+4)}{(x+6)(x+4)} + \frac{9(x+6)}{(x+4)(x+6)}$

$=\frac{4(x+4) +9(x+6)}{(x+6)(x+4)}$

$=\frac{4x+16+9x+54}{x^{2}+10x+24}$

$=\frac{13x+70}{x^{2}+10x+24}$

Report an Error

Example Question #1 : Properties Of Fractions

Simplify the expression:

$\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$

Possible Answers:

$\frac{7x+63}{x^{2}+8x+16}$

$\frac{x^{2}-40}{x^{2}+10x+21}$

$\frac{9x-63}{x^{2}+6x+5}$

$\frac{7x-49}{x^{2}-6x-7}$

$\frac{9x+18}{x^{2}+10x+9}$

Correct answer:

$\frac{x^{2}-40}{x^{2}+10x+21}$

Explanation:

In order to simplify the expression $\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$ , first note that the denominators in both terms share a factor:

$\frac{x-7}{x+3}+ \frac{9}{(x+3)(x+7)}$

Find the Least Common Denominator (LCD) of both terms:

$\frac{(x-7)(x+7)}{(x+3)(x+7)}+ \frac{9}{(x+3)(x+7)}$

$=\frac{x^{2}-49}{x^{2}+10x+21}+ \frac{9}{x^{2}+10x+21}$

Finally, combine like terms:

$\frac{x^{2}-49+9}{x^{2}+10x+21}$

$=\frac{x^{2}-40}{x^{2}+10x+21}$

Report an Error

Example Question #1663 : Algebra Ii

Simplify the expression:

$\frac{5}{x+2} -\frac{10}{x+3}$

Possible Answers:

$\frac{-5}{x^{2}+5x+6}$

$\frac{-5x+5}{x^{2}-5x-6}$

$\frac{-5}{x}$

$\frac{-5x-5}{x^{2}+5x+6}$

$\frac{5x-5}{x^{2}+5x-6}$

Correct answer:

$\frac{-5x-5}{x^{2}+5x+6}$

Explanation:

$\frac{5}{x+2} -\frac{10}{x+3}$

1. Create a common denominator between the two fractions.

$\frac{5(x+3)}{(x+2)(x+3)} -\frac{10(x+2)}{(x+3)(x+2)}$

2. Simplify.

$=\frac{5(x+3)-10(x+2)}{(x+2)(x+3)}$

$=\frac{5x+15-10x-20}{x^{2}+5x+6}$

$=\frac{-5x-5}{x^{2}+5x+6}$

Report an Error

Example Question #4 : Understanding Rational Expressions

Find the values of which will make this rational expression undefined:

$\frac{x-1}{(x+2)(x-10)}$

Possible Answers:

x=-1,2

x=-10,2

x=-2,1,10

x=-2,10

x=-2,1

Correct answer:

x=-2,10

Explanation:

For a rational expression to be undefined, the denominator must be equal to .

1. Set the denominator equal to .

(x+2)(x-10)=0

2. Set the factors equal to and solve for .

x+2=0

x=-2

and

x-10=0

x=10

Report an Error

Example Question #5 : Understanding Rational Expressions

Which value of makes the following expression undefined?

$\frac{x+1}{x-1}$

Possible Answers:

$\text{All real numbers}$

$\text{Cannot be determined}$

Correct answer:

Explanation:

A rational expression is undefined when the denominator is zero.

x-1=0

x=1

The denominator is zero when x=1 .

Report an Error

View Algebra 2 Tutors

James
Certified Tutor

St. Mary of the Plains College, Bachelors, Mathematics. Baker University, Masters, Business Administration and Management.

View Algebra 2 Tutors

Anne
Certified Tutor

University of Maryland-Baltimore, Doctor of Science, Accounting.

View Algebra 2 Tutors

Satweka
Certified Tutor

The University of Texas at Dallas, Master of Science, Biomedical Engineering.

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Calculus Tutors in Miami, Physics Tutors in Los Angeles, Statistics Tutors in Miami, LSAT Tutors in Miami, GMAT Tutors in Miami, Computer Science Tutors in New York City, English Tutors in Chicago, Physics Tutors in Boston, Spanish Tutors in Seattle, SSAT Tutors in Seattle

Popular Courses & Classes

SAT Courses & Classes in Washington DC, SAT Courses & Classes in Philadelphia, GRE Courses & Classes in Washington DC, LSAT Courses & Classes in Denver, LSAT Courses & Classes in Houston, SSAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Boston, Spanish Courses & Classes in Los Angeles, GMAT Courses & Classes in Denver, GRE Courses & Classes in Boston

Popular Test Prep

MCAT Test Prep in San Diego, MCAT Test Prep in Seattle, GMAT Test Prep in Miami, ISEE Test Prep in Chicago, GRE Test Prep in Philadelphia, SAT Test Prep in Houston, ISEE Test Prep in Dallas Fort Worth, MCAT Test Prep in Washington DC, ACT Test Prep in San Diego, LSAT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Algebra II : Understanding Rational Expressions

Study concepts, example questions & explanations for Algebra II

All Algebra II Resources

Example Questions

Example Question #1 : Understanding Rational Expressions

Example Question #2 : Understanding Rational Expressions

Example Question #2 : Definition Of Rational Expression

Example Question #4 : Rational Expressions

Example Question #3 : Understanding Rational Expressions

Example Question #2 : Properties Of Fractions

Example Question #1 : Properties Of Fractions

Example Question #1663 : Algebra Ii

Example Question #4 : Understanding Rational Expressions

Example Question #5 : Understanding Rational Expressions

All Algebra II Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: