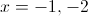We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Algebra II : Properties of Fractions

Study concepts, example questions & explanations for Algebra II

Create An Account

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 Next →

Example Question #5 : Rational Expressions

Find the values of which will make the given rational expression undefined:

$\frac{x+5}{\left ( x-1 \right )\left ( x+2 \right )}$

Possible Answers:

x = -5, 1

x = 1, -2

x = -1, -2

x = -5, +2

x = -1, 2

Correct answer:

x = 1, -2

Explanation:

If $\left ( x-1 \right ) = 0$ or $\left ( x+2 \right ) =0$ , the denominator is 0, which makes the expression undefined.

This happens when x = 1 or when x = -2.

Therefore the correct answer is x = 1, -2 .

Report an Error

Example Question #6 : Rational Expressions

Simply the expression:

$\frac{4}{x+6}+ \frac{9}{x+4}$

Possible Answers:

$\frac{14x+20}{x^{2}+9x+15}$

$\frac{15x+70}{x^{2}+4x+18}$

$\frac{13x+70}{x^{2}+10x+24}$

$\frac{12x+40}{x^{2}-4x+10}$

$\frac{18x+60}{x^{2}-10x+28}$

Correct answer:

$\frac{13x+70}{x^{2}+10x+24}$

Explanation:

In order to simplify the expression $\frac{4}{x+6}+ \frac{9}{x+4}$ , we need to ensure that both terms have the same denominator. In order to do so, find the Least Common Denominator (LCD) for both terms and simplify the expression accordingly:

$\frac{4(x+4)}{(x+6)(x+4)} + \frac{9(x+6)}{(x+4)(x+6)}$

$=\frac{4(x+4) +9(x+6)}{(x+6)(x+4)}$

$=\frac{4x+16+9x+54}{x^{2}+10x+24}$

$=\frac{13x+70}{x^{2}+10x+24}$

Report an Error

Example Question #7 : Rational Expressions

Simplify the expression:

$\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$

Possible Answers:

$\frac{9x+18}{x^{2}+10x+9}$

$\frac{9x-63}{x^{2}+6x+5}$

$\frac{x^{2}-40}{x^{2}+10x+21}$

$\frac{7x+63}{x^{2}+8x+16}$

$\frac{7x-49}{x^{2}-6x-7}$

Correct answer:

$\frac{x^{2}-40}{x^{2}+10x+21}$

Explanation:

In order to simplify the expression $\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$ , first note that the denominators in both terms share a factor:

$\frac{x-7}{x+3}+ \frac{9}{(x+3)(x+7)}$

Find the Least Common Denominator (LCD) of both terms:

$\frac{(x-7)(x+7)}{(x+3)(x+7)}+ \frac{9}{(x+3)(x+7)}$

$=\frac{x^{2}-49}{x^{2}+10x+21}+ \frac{9}{x^{2}+10x+21}$

Finally, combine like terms:

$\frac{x^{2}-49+9}{x^{2}+10x+21}$

$=\frac{x^{2}-40}{x^{2}+10x+21}$

Report an Error

Example Question #8 : Rational Expressions

Simplify the expression:

$\frac{5}{x+2} -\frac{10}{x+3}$

Possible Answers:

$\frac{-5x-5}{x^{2}+5x+6}$

$\frac{-5}{x^{2}+5x+6}$

$\frac{5x-5}{x^{2}+5x-6}$

$\frac{-5x+5}{x^{2}-5x-6}$

$\frac{-5}{x}$

Correct answer:

$\frac{-5x-5}{x^{2}+5x+6}$

Explanation:

$\frac{5}{x+2} -\frac{10}{x+3}$

1. Create a common denominator between the two fractions.

$\frac{5(x+3)}{(x+2)(x+3)} -\frac{10(x+2)}{(x+3)(x+2)}$

2. Simplify.

$=\frac{5(x+3)-10(x+2)}{(x+2)(x+3)}$

$=\frac{5x+15-10x-20}{x^{2}+5x+6}$

$=\frac{-5x-5}{x^{2}+5x+6}$

Report an Error

Example Question #9 : Rational Expressions

Find the values of which will make this rational expression undefined:

$\frac{x-1}{(x+2)(x-10)}$

Possible Answers:

x=-2,1

x=-2,1,10

x=-2,10

x=-10,2

x=-1,2

Correct answer:

x=-2,10

Explanation:

For a rational expression to be undefined, the denominator must be equal to .

1. Set the denominator equal to .

(x+2)(x-10)=0

2. Set the factors equal to and solve for .

x+2=0

x=-2

and

x-10=0

x=10

Report an Error

Example Question #10 : Rational Expressions

Which value of makes the following expression undefined?

$\frac{x+1}{x-1}$

Possible Answers:

$\text{Cannot be determined}$

$\text{All real numbers}$

Correct answer:

Explanation:

A rational expression is undefined when the denominator is zero.

x-1=0

x=1

The denominator is zero when x=1 .

Report an Error

Example Question #1 : Properties Of Fractions

Compute: $\frac{x}{z}+\frac{y}{x}+\frac{z}{y}$

Possible Answers:

$\frac{x^2y+y^2z+xz^2}{xyz}$

xyz

$\frac{x^2+y^2+z^2}{xyz}$

$\frac{1}{xyz}$

Correct answer:

$\frac{x^2y+y^2z+xz^2}{xyz}$

Explanation:

The terms cannot be added unless the denominators are common. To get the same denominator for each term, find the LCD by multiplying , , and .

The denominator for the three terms are xyz . Evaluate each term of the given problem.

$\frac{x}{z}+\frac{y}{x}+\frac{z}{y}=\frac{x^2y}{xyz}+\frac{y^2z}{xyz}+\frac{xz^2}{xyz}=\frac{x^2y+y^2z+xz^2}{xyz}$

Report an Error

Example Question #2 : Properties Of Fractions

Bob is left with a whole pizza for dinner. He eats $\frac{1}{3}$ of this pizza. Afterward, Wendy eats the rest of the pizza except the crusts, and leaves $\frac{1}{20}$ of the whole pizza remaining. What fraction did Wendy eat?

Possible Answers:

$\frac{3}{40}$

$\frac{37}{60}$

$\frac{13}{20}$

$\frac{37}{40}$

$\frac{59}{60}$

Correct answer:

$\frac{37}{60}$

Explanation:

A whole pizza is one unit.

After Bob ate $\frac{1}{3}$ of this pizza, $\frac{2}{3}$ will remain.

Wendy eats an amount of the two-thirds of the pizza, and leaves $\frac{1}{20}$ of the whole pizza. Write the equation and solve for .

$\frac{2}{3}-x=\frac{1}{20}$

$\frac{40}{60}-x=\frac{3}{60}$

$x=\frac{37}{60}$

Report an Error

Example Question #3 : Properties Of Fractions

Simplify, if possible: $\frac{1}{a}-\frac{2a+2b}{a-b}$

Possible Answers:

$\frac{-4a^2-2a+2ab}{a^2-ab}$

$\frac{a-b-2a^2+2ab}{a^2-ab}$

$\frac{1-2a-2b}{b}$

$\frac{a-b-2a^2-2ab}{a^2-ab}$

$\frac{1}{a-b}$

Correct answer:

$\frac{a-b-2a^2-2ab}{a^2-ab}$

Explanation:

In order to subtract the numerators, first find the least common denominator in the problem. This can usually be found by multiplying the unlike denominators together.

a(a-b)=a^2-ab

For each term, multiply the numerator with what was multiplied on the denominator to get the least common denominator.

$\frac{1(a-b)}{a(a-b)}-\frac{(2a+2b)(a)}{(a-b)(a)}$

Simplify.

$=\frac{a-b}{a^2-ab}-\frac{2a^2+2ab}{a^2-ab}$

$= \frac{a-b-(2a^2+2ab)}{a^2-ab}$

$= \frac{a-b-2a^2-2ab}{a^2-ab}$

There are no other terms or common factors here that can be simplified. Since there are no like terms in the numerator or denominator, this is fully simplified.

The answer is:

$\frac{a-b-2a^2-2ab}{a^2-ab}$

Report an Error

Example Question #4 : Properties Of Fractions

Simplify: $\frac{1}{3x}-\frac{1}{3-x}$

Possible Answers:

$\frac{2x-4}{3x^2-9x}$

$\frac{x}{9-x}$

$\frac{3-4x}{ 9x-3x^2}$

$\frac{3-2x}{ 9x-3x^2}$

$-\frac{4x}{9}$

Correct answer:

$\frac{3-4x}{ 9x-3x^2}$

Explanation:

In order to simplify, we will need a common denominator.

Multiply the denominators together.

3x(3-x) = 9x-3x^2

This will be the new denominator.

Convert the fractions.

$\frac{1}{3x}-\frac{1}{3-x}=\frac{3-x}{ 9x-3x^2}-\frac{3x}{ 9x-3x^2}$

Combine the fractions as a single fraction.

$\frac{3-x-(3x)}{ 9x-3x^2}$

Subtract the numerators.

The answer is: $\frac{3-4x}{ 9x-3x^2}$

Report an Error

← Previous 1 2 Next →

View Algebra 2 Tutors

Emilie
Certified Tutor

Western Governors University, Bachelor of Science, Accounting and Business Management. Western Governors University, Master o...

View Algebra 2 Tutors

Louis
Certified Tutor

University of California-Los Angeles, Bachelor in Arts, Film Production.

View Algebra 2 Tutors

Anthony
Certified Tutor

George Washington University, Bachelor of Science, Biology, General. Columbia University in the City of New York, Master of S...

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in Atlanta, Chemistry Tutors in Houston, MCAT Tutors in Los Angeles, LSAT Tutors in New York City, ISEE Tutors in Miami, Statistics Tutors in Miami, GRE Tutors in Miami, GRE Tutors in Philadelphia, Spanish Tutors in Washington DC, ACT Tutors in Phoenix

Popular Courses & Classes

GMAT Courses & Classes in New York City, ISEE Courses & Classes in Houston, ACT Courses & Classes in Houston, Spanish Courses & Classes in Boston, GRE Courses & Classes in Phoenix, SAT Courses & Classes in Chicago, GRE Courses & Classes in Boston, LSAT Courses & Classes in Denver, MCAT Courses & Classes in Denver, SSAT Courses & Classes in Washington DC

Popular Test Prep

GMAT Test Prep in Washington DC, SSAT Test Prep in Miami, SAT Test Prep in Phoenix, GRE Test Prep in Los Angeles, ACT Test Prep in Houston, LSAT Test Prep in Washington DC, SSAT Test Prep in Atlanta, LSAT Test Prep in Los Angeles, SSAT Test Prep in Denver, SSAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Algebra II : Properties of Fractions

Study concepts, example questions & explanations for Algebra II

All Algebra II Resources

Example Questions

Example Question #5 : Rational Expressions

Example Question #6 : Rational Expressions

Example Question #7 : Rational Expressions

Example Question #8 : Rational Expressions

Example Question #9 : Rational Expressions

Example Question #10 : Rational Expressions

Example Question #1 : Properties Of Fractions

Example Question #2 : Properties Of Fractions

Example Question #3 : Properties Of Fractions

Example Question #4 : Properties Of Fractions

All Algebra II Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: