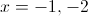Call Now to Set Up Tutoring:

(888) 888-0446

Algebra II : Algebra II

Study concepts, example questions & explanations for Algebra II

Create An Account

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #7 : Quadratic Inequalities

Solve:

$7x^2+29x-30\geq0$

Possible Answers:

$(-\infty, -5]$

$[-5, \frac{6}{7}]$

$[\frac{6}{7}, \infty)$

$(-\infty, -5]\cup[\frac{6}{7}, \infty)$

Correct answer:

$(-\infty, -5]\cup[\frac{6}{7}, \infty)$

Explanation:

First, set the inequality to zero and solve for .

7x^2+29x-30=0

(7x-6)(x+5)

$x=\frac{6}{7}, x=-5$

Now, plot these two numbers on to a number line.

Notice how these numbers divide the number line into three regions:

$(-\infty, -5], [-5, \frac{6}{7}], [\frac{6}{7}, \infty)$

Now, you will choose a number from each of these regions to test to plug back into the inequality to see if the inequality holds true.

For $(-\infty, -5]$ , let x=-6

(7(6)-6)(6+5)=396

Since this solution is greater than or equal to , the solution can be found in this region.

For $[-5, \frac{6}{7}]$ , let x=0

(7(0)-6)(0+5)=-6

Since this is less than or equal to , the solution cannot be found in this region.

For $[\frac{6}{7}, \infty)$ , let x=1

(7(1)-6)(1+5)=6

Since this is greater than or equal to , the solution can be found in this region.

Because the solution can be found in every single region, the answer to this inequality is $(-\infty, -5]\cup[\frac{6}{7}, \infty)$

Report an Error

Example Question #10 : Quadratic Inequalities

Which value for would satisfy the inequality $\small \small x^2 -5x -36< 0$ ?

Possible Answers:

$\small x = -5$

$\small x = 10$

$\small x = 1$

$\small x = -4$

Not enough information to solve

Correct answer:

$\small x = 1$

Explanation:

First, we can factor the quadratic to give us a better understanding of its graph. Factoring gives us: $\small (x+4)(x-9)< 0$ . Now we know that the quadratic has zeros at and . Furthermore this information reveals that the quadratic is positive. Using this information, we can sketch a graph like this:

Sketch inequality

We can see that the parabola is below the x-axis (in other words, less than ) between these two zeros and .

The only x-value satisfying the inequality $\small \small -4 < x < 9$ is $\small x=1$ .

The value of $\small x=-4$ would work if the inequality were inclusive, but since it is strictly less than instead of less than or equal to , that value will not work.

Report an Error

Example Question #1 : How To Find The Solution Of A Rational Equation With A Binomial Denominator

Which of the following fractions is NOT equivalent to $- \frac{x-5}{2x + 3}$ ?

Possible Answers:

$\frac{x-5}{-2x-3}$

$\frac{x-5}{\left -(2x+3 \right )}$

$\frac{\left -(x-5 \right )}{2x+3}$

$\frac{-x+5}{2x+3}$

$\frac{x+5}{2x + 3}$

Correct answer:

$\frac{x+5}{2x + 3}$

Explanation:

We know that $-\frac{a}{b}$ is equivalent to $\frac{-a}{b}$ or $\frac{a}{-b}$ .

By this property, there is no way to get $\frac{x+5}{2x+3}$ from $-\frac{x-5}{2x+3}$ .

Therefore the correct answer is $\frac{x+5}{2x+3}$ .

Report an Error

Example Question #1 : Rational Expressions

Determine the domain of

$\frac{x+1}{x-1}$

Possible Answers:

$x\neq1$

x=1

All real numbers

$x\neq-1$

Correct answer:

$x\neq1$

Explanation:

Because the denominator cannot be zero, the domain is all other numbers except for 1, or

$x\neq1$

Report an Error

Example Question #2 : Definition Of Rational Expression

Simplify:

$\frac{x^2-1}{x^2+4x+2}\div\frac{x^2+2x+1}{x^2+3x+2}$

Possible Answers:

x-1

$\frac{1}{\frac{1}{x+1}}$

$\frac{x+2}{x-1}$

$\frac{x+2}{x+1}$

$\frac{x-1}{x+2}$

Correct answer:

$\frac{x-1}{x+2}$

Explanation:

This problem is a lot simpler if we factor all the expressions involved before proceeding:

$\frac{x^2-1}{x^2+4x+2}\div\frac{x^2+2x+1}{x^2+3x+2} \vspace{5mm}\\ = \frac{(x+1)(x-1)}{(x+2)(x+2)}\div\frac{(x+1)(x+1)}{(x+2)(x+1)}$

Next let's remember how we divide one fraction by another—by multiplying by the reciprocal:

$\frac{(x+1)(x-1)}{(x+2)(x+2)}\div\frac{(x+1)(x+1)}{(x+2)(x+1)} \vspace{5mm}\\ = \frac{(x+1)(x-1)}{(x+2)(x+2)}\times\frac{(x+2)(x+1)}{(x+1)(x+1)}$

In this form, we can see that a lot of terms are going to start canceling with each other. All that we're left with is just $\frac{x-1}{x+2}$ .

Report an Error

Example Question #4 : Rational Expressions

Which of the following is the best definition of a rational expression?

Possible Answers:

$\textup{The ratio of any two real numbers.}$

$\textup{The ratio of any two imaginary numbers.}$

$\textup{The ratio of two fractions that may have a zero denominator.}$

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

$\textup{The ratio of two polynomials that must be different from each other.}$

Correct answer:

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

Explanation:

The rational expression is a ratio of two polynomials.

$\frac{A(x)}{B(x)}$

The denominator cannot be zero.

An example of a rational expression is:

$\frac{x^2+x-5}{x+8}$

The answer is:

$\textup{The ratio of two polynomials that cannot have a zero denominator.}$

Report an Error

Example Question #1 : Properties Of Fractions

Find the values of which will make the given rational expression undefined:

$\frac{x+5}{\left ( x-1 \right )\left ( x+2 \right )}$

Possible Answers:

x = -1, 2

x = -1, -2

x = -5, 1

x = -5, +2

x = 1, -2

Correct answer:

x = 1, -2

Explanation:

If $\left ( x-1 \right ) = 0$ or $\left ( x+2 \right ) =0$ , the denominator is 0, which makes the expression undefined.

This happens when x = 1 or when x = -2.

Therefore the correct answer is x = 1, -2 .

Report an Error

Example Question #2 : Properties Of Fractions

Simply the expression:

$\frac{4}{x+6}+ \frac{9}{x+4}$

Possible Answers:

$\frac{18x+60}{x^{2}-10x+28}$

$\frac{15x+70}{x^{2}+4x+18}$

$\frac{12x+40}{x^{2}-4x+10}$

$\frac{13x+70}{x^{2}+10x+24}$

$\frac{14x+20}{x^{2}+9x+15}$

Correct answer:

$\frac{13x+70}{x^{2}+10x+24}$

Explanation:

In order to simplify the expression $\frac{4}{x+6}+ \frac{9}{x+4}$ , we need to ensure that both terms have the same denominator. In order to do so, find the Least Common Denominator (LCD) for both terms and simplify the expression accordingly:

$\frac{4(x+4)}{(x+6)(x+4)} + \frac{9(x+6)}{(x+4)(x+6)}$

$=\frac{4(x+4) +9(x+6)}{(x+6)(x+4)}$

$=\frac{4x+16+9x+54}{x^{2}+10x+24}$

$=\frac{13x+70}{x^{2}+10x+24}$

Report an Error

Example Question #1 : Properties Of Fractions

Simplify the expression:

$\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$

Possible Answers:

$\frac{7x+63}{x^{2}+8x+16}$

$\frac{x^{2}-40}{x^{2}+10x+21}$

$\frac{9x-63}{x^{2}+6x+5}$

$\frac{7x-49}{x^{2}-6x-7}$

$\frac{9x+18}{x^{2}+10x+9}$

Correct answer:

$\frac{x^{2}-40}{x^{2}+10x+21}$

Explanation:

In order to simplify the expression $\frac{x-7}{x+3}+ \frac{9}{x^{2}+10x+21}$ , first note that the denominators in both terms share a factor:

$\frac{x-7}{x+3}+ \frac{9}{(x+3)(x+7)}$

Find the Least Common Denominator (LCD) of both terms:

$\frac{(x-7)(x+7)}{(x+3)(x+7)}+ \frac{9}{(x+3)(x+7)}$

$=\frac{x^{2}-49}{x^{2}+10x+21}+ \frac{9}{x^{2}+10x+21}$

Finally, combine like terms:

$\frac{x^{2}-49+9}{x^{2}+10x+21}$

$=\frac{x^{2}-40}{x^{2}+10x+21}$

Report an Error

Example Question #1663 : Algebra Ii

Simplify the expression:

$\frac{5}{x+2} -\frac{10}{x+3}$

Possible Answers:

$\frac{-5}{x^{2}+5x+6}$

$\frac{-5x+5}{x^{2}-5x-6}$

$\frac{-5}{x}$

$\frac{-5x-5}{x^{2}+5x+6}$

$\frac{5x-5}{x^{2}+5x-6}$

Correct answer:

$\frac{-5x-5}{x^{2}+5x+6}$

Explanation:

$\frac{5}{x+2} -\frac{10}{x+3}$

1. Create a common denominator between the two fractions.

$\frac{5(x+3)}{(x+2)(x+3)} -\frac{10(x+2)}{(x+3)(x+2)}$

2. Simplify.

$=\frac{5(x+3)-10(x+2)}{(x+2)(x+3)}$

$=\frac{5x+15-10x-20}{x^{2}+5x+6}$

$=\frac{-5x-5}{x^{2}+5x+6}$

Report an Error

View Algebra 2 Tutors

Shallu
Certified Tutor

Hans Raj Mahila Maha Vidyalaya, Bachelor of Science, Computer Science. Jiwaji University, Master of Science, Computer Science.

View Algebra 2 Tutors

Anjali
Certified Tutor

Delhi University, Bachelor of Science, Physics. Kumuan University, Master of Science, Physics.

View Algebra 2 Tutors

Mariam
Certified Tutor

Yarmouk University, Bachelor of Science, Mathematics. Touro College, Master of Science, Teacher Education, Multiple Levels.

All Algebra II Resources

10 Diagnostic Tests 630 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

French Tutors in Philadelphia, English Tutors in Chicago, Chemistry Tutors in Washington DC, ISEE Tutors in Chicago, Physics Tutors in Washington DC, Math Tutors in Philadelphia, SAT Tutors in Houston, GRE Tutors in Los Angeles, Calculus Tutors in Seattle, Physics Tutors in San Diego

Popular Courses & Classes

ACT Courses & Classes in Phoenix, GMAT Courses & Classes in Houston, LSAT Courses & Classes in Boston, SAT Courses & Classes in Atlanta, GRE Courses & Classes in Los Angeles, LSAT Courses & Classes in San Diego, MCAT Courses & Classes in Dallas Fort Worth, GRE Courses & Classes in New York City, ISEE Courses & Classes in Atlanta, GMAT Courses & Classes in Boston

Popular Test Prep

ACT Test Prep in Phoenix, GMAT Test Prep in Philadelphia, GMAT Test Prep in Dallas Fort Worth, SSAT Test Prep in Boston, GMAT Test Prep in Boston, GRE Test Prep in San Diego, SSAT Test Prep in Washington DC, GRE Test Prep in Phoenix, ISEE Test Prep in Washington DC, SSAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Algebra II : Algebra II

Study concepts, example questions & explanations for Algebra II

All Algebra II Resources

Example Questions

Example Question #7 : Quadratic Inequalities

Example Question #10 : Quadratic Inequalities

Example Question #1 : How To Find The Solution Of A Rational Equation With A Binomial Denominator

Example Question #1 : Rational Expressions

Example Question #2 : Definition Of Rational Expression

Example Question #4 : Rational Expressions

Example Question #1 : Properties Of Fractions

Example Question #2 : Properties Of Fractions

Example Question #1 : Properties Of Fractions

Example Question #1663 : Algebra Ii

All Algebra II Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: