All ACT Math Resources
Example Questions
Example Question #1 : Cubes
Our backyard pool holds 10,000 gallons. Its average depth is 4 feet deep and it is 10 feet long. If there are 7.48 gallons in a cubic foot, how wide is the pool?
100 ft
33 ft
30 ft
133 ft
7.48 ft
33 ft
There are 7.48 gallons in cubic foot. Set up a ratio:
1 ft3 / 7.48 gallons = x cubic feet / 10,000 gallons
Pool Volume = 10,000 gallons = 10,000 gallons * (1 ft3/ 7.48 gallons) = 1336.9 ft3
Pool Volume = 4ft x 10 ft x WIDTH = 1336.9 cubic feet
Solve for WIDTH:
4 ft x 10 ft x WIDTH = 1336.9 cubic feet
WIDTH = 1336.9 / (4 x 10) = 33.4 ft
Example Question #2 : Cubes
A cube has a volume of 64cm3. What is the area of one side of the cube?
16cm2
16cm3
4cm
16cm
4cm2
16cm2
The cube has a volume of 64cm3, making the length of one edge 4cm (4 * 4 * 4 = 64).
So the area of one side is 4 * 4 = 16cm2
Example Question #1 : How To Find The Length Of An Edge Of A Cube
A cube as the volume of 
Find the length of a side of this cube.
The formula to find the volume of the cube is
Since we know the volume, we can set up the equation
Example Question #1 : Cubes
A cube has a surface area of 
To find the length of the side of a square given the surface area, use the surface area formula and solve for 



Example Question #3 : How To Find The Length Of An Edge Of A Cube
A certain cubic box when unfolded and laid flat on a table covers exactly 
To find the length of the edge of a cube from its surface area, remember that 

So, the box is 
Example Question #651 : Geometry
Given the volume of a cube is 
To find side length, simply realize that volume is the side length cubed. Thus,
Example Question #652 : Geometry
Find the length of the edge of a cube given the volume is 
To solve, simply take the cube root of the volume. Thus,
Example Question #1 : How To Find The Diagonal Of A Cube
Find the length of the diagonal of a cube with side length of 
We begin with a picture, noting that the diagonal, labeled as 
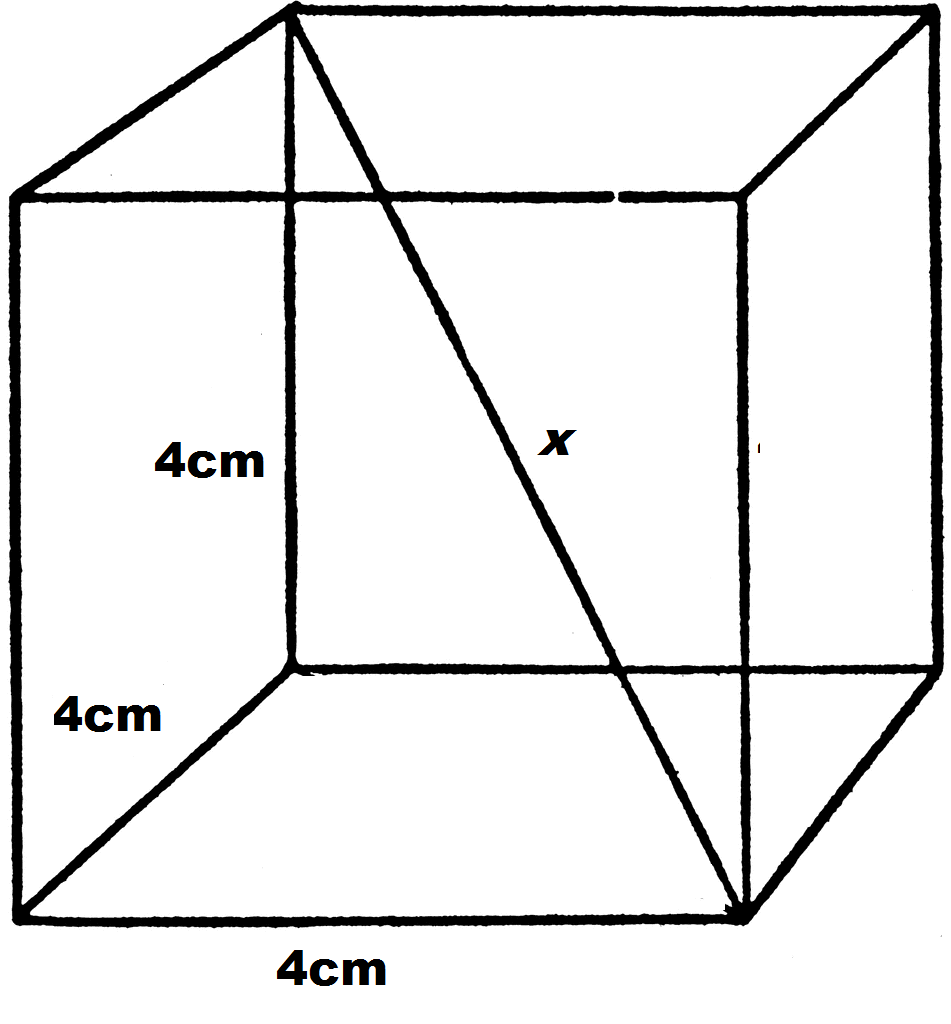
However, the trick to solving the problem is to also draw in the diagonal of the bottom face of the cube, which we labeled 

Note that this creates two right triangles. Though our end goal is to find 

Now that we know the value of 

Taking the square root of both sides and simplifying gives the answer.
Example Question #24 : Solid Geometry
What is the diagonal length for a cube with volume of 







Recall that the volume of a cube is computed using the equation


So, for our data, we know:
Using your calculator, take the cube root of both sides. You can always do this by raising 

If you get 



This is 

Example Question #1 : How To Find The Diagonal Of A Cube
What is the length of the diagonal of a cube with a volume of 
Recall that the diagonal of a cube is most easily found when you know that cube's dimensions. For the volume of a cube, the pertinent equation is:


Now, you could factor this by hand or use your calculator. You will see that 

Now, we find the diagonal by using a three-dimensional version of the Pythagorean Theorem / distance formula:

You can rewrite this:
Certified Tutor
All ACT Math Resources








![\sqrt[3]64=4](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/250153/gif.latex)

















![s=\sqrt[3]{V}=\sqrt[3]{64}=\sqrt[3]{4^3}=4](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/541733/gif.latex)




![s=\sqrt[3]{V}=\sqrt[3]{27}=3](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/566739/gif.latex)
















![\sqrt[3]{91.125} = \sqrt[3]{s^3}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/282618/gif.latex)













