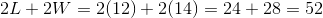A rhombus is a quadrilateral with two sets of parallel sides as well as equal opposite angles. Since the lines drawn inside the rhombus are diagonals,  and  are each bisected into two equal angles.

Therefore,  , which creates a triangle in the upper right quadrant of the kite. The sum of angles in a triangle is 180 degreees.

Thus,

Since  is only half of ,

In a rhombus, opposite angles are equal to each other. Therefore we can set  and  equal to one another and solve for :

Therefore,

A rhombus, like any other quadrilateral, has a sum of angles of 360 degrees.

In order to solve this problem we need the following key piece of knowledge: the interior angles of a quadrilateral add up to 360 degrees. Now, we can write the following equation:

When we combine like terms, we get the following:

We will need to subtract 71 from both sides of the equation:

Now, we will divide both sides of the equation by 17.

We now have a value for the x-variable; however, the problem is not finished. The question asks for the measure of the smallest angle. We know that the smallest angle will be one of the following: 

 or 

In order to find out, we will substitute 17 degrees for the x-variable.

Because 51 degrees is less than 71 degrees, the measure of the smallest angle is the following:

To answer this question, we must find the perimeter of the fence the homeowner is wanting to create.

To find the perimeter of a rectangle, we multiply the length by two, multiply the width by two, and add these two numbers together. The equation can be represented as this:

We must then plug in our values of  and  given to us for the length and width.

So for this data:

Therefore, the amount of fencing needed to fully surround the dog's enclosure is .

The key here is to know that a rhombus has two pairs of congruent angles. In other words, the two acute angles of a rhombus are equal and the two obtuse angles are equal. 

In this problem, since the two acute angles add up to  and they must both be the same amount, each of the acute angles must be .

It is also important to know that the four angles of a rhombus add up to . If the two acute angles add up to , then that means that the two obtuse angles must add up to , or .

Finally, because the obtuse angles add up to  and they must be congruent, each of the obtuse angles must be .

First, consider that the sum total of the four interior angles in any rhombus must equal . Furthermore, a rhombus must have two sets of equivalent opposite interior angles, and a rhombus must have two sets of adjacent interior angles. The adjacent interior angles must be supplementary—meaning they have a sum total of . 

One way to approach this problem is to realize that each of the remaining two angles must have the same measurement, and that each will be supplementary angles with .  Find the difference between  and  to find the solution. 

The correct answer is: 

A rhombus must have two sets of equivalent opposite interior angles, and a rhombus must have two sets of adjacent interior angles. The adjacent interior angles must be supplementary—meaning they have a sum total of .

If a rhombus has an interior angle that has a measurement of , the adjacent angle must equal: 


 

 The sum total of the four interior angles in any rhombus must equal . Furthermore, a rhombus must have two sets of equivalent opposite interior angles, and a rhombus must have two sets of adjacent interior angles. The adjacent interior angles must be supplementary—meaning they have a sum total of .

Since, two of the opposite interior angles in this rhombus have a sum measurement of , the sum of the remaining two angles must equal:



To check your answer note: 

 (meaning the sum of the four interior angles equals ).

 A rhombus must have two sets of equivalent opposite interior angles, and a rhombus must have two sets of adjacent interior angles. The adjacent interior angles must be supplementary—meaning they have a sum total of .

Since this problem involves supplementary angles, the solution is: 

The sum total of the four interior angles in any rhombus must equal . Furthermore, a rhombus must have two sets of equivalent opposite interior angles, and a rhombus must have two sets of adjacent interior angles. The adjacent interior angles must be supplementary—meaning they have a sum total of . 

One way to approach this problem is to realize that each of the remaining two angles must have the same measurement, and that each will be supplementary angles with .

Find the difference between  and  to find the solution.