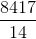We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

ACT Math : Algebraic Fractions

Study concepts, example questions & explanations for ACT Math

Create An Account

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #33 : Algebraic Fractions

Simplify:

$\frac{\frac{1}{4}+\frac{5}{7}}{\frac{2}{5}}$

Possible Answers:

$\frac{81}{7}$

$\frac{27}{70}$

$\frac{135}{56}$

$\frac{47}{5}$

$\frac{98}{71}$

Correct answer:

$\frac{135}{56}$

Explanation:

Begin by simplifying the numerator.

$\frac{1}{4}+\frac{5}{7}$ has a common denominator of . Therefore, we can rewrite it as:

$\frac{7}{28}+\frac{20}{28}=\frac{27}{28}$

Now, in our original problem this is really is:

$\frac{\frac{27}{28}}{\frac{2}{5}}$

When you divide by a fraction, you really multiply by the reciprocal:

$\frac{\frac{27}{28}}{\frac{2}{5}}=\frac{27}{28} \cdot\frac{5}{2}=\frac{135}{56}$

Report an Error

Example Question #34 : Algebraic Fractions

Simplify:

$\frac{\frac{81}{5}+\frac{7}{25}}{\frac{1}{9}-\frac{2}{7}}$

Possible Answers:

$\frac{8414}{41}$

$-\frac{25956}{275}$

$-\frac{4532}{1575}$

$-\frac{551}{12}$

$\frac{8417}{14}$

Correct answer:

$-\frac{25956}{275}$

Explanation:

Begin by simplifying the numerator and the denominator.

Numerator

$\frac{81}{5}+\frac{7}{25}$ has a common denominator of . Therefore, we have:

$\frac{405}{25}+\frac{7}{25} = \frac{412}{25}$

Denominator

$\frac{1}{9}-\frac{2}{7}$ has a common denominator of . Therefore, we have:

$\frac{7}{63}-\frac{18}{63}=-\frac{11}{63}$

Now, reconstructing our fraction, we have:

$\frac{\frac{412}{25}}{-\frac{11}{63}}$

To make this division work, you multiply the numerator by the reciprocal of the denominator:

$\frac{\frac{412}{25}}{-\frac{11}{63}} = \frac{412}{25} \cdot -\frac{63}{11} =-\frac{25956}{275}$

Report an Error

Example Question #31 : Algebraic Fractions

Simplify: $(\frac{x-4}{0.5})\div (\frac{1}{x+4})$

Possible Answers:

$\frac{3}{2x^2}-16$

$\frac{1}{2}$

2x^2-32

None of the other answer choices are correct.

Correct answer:

2x^2-32

Explanation:

Recall that dividing is equivalent multiplying by the reciprocal. Therefore, ((x - 4) / (1 / 2)) / (1 / (x + 4)) = ((x - 4) * 2) * (x + 4) / 1.

Let's simplify this further:

(2x – 8) * (x + 4) = 2x² – 8x + 8x – 32 = 2x² – 32

Report an Error

Example Question #32 : Algebraic Fractions

Solve for :

$\frac{3x}{8}+12=\frac{1}{3}-2x$

Possible Answers:

$\frac{100}{21}$

$-\frac{81}{13}$

$-\frac{280}{57}$

$\frac{43}{2}$

$-\frac{5}{7}$

Correct answer:

$-\frac{280}{57}$

Explanation:

Begin by isolating the variables:

$\frac{3x}{8}+2x=\frac{1}{3}-12$

Now, the common denominator of the variable terms is . The common denominator of the constant values is . Thus, you can rewrite your equation:

$\frac{3x}{8}+\frac{16x}{8}=\frac{1}{3}-\frac{36}{3}$

Simplify:

$\frac{19x}{8}=-\frac{35}{3}$

Cross-multiply:

19x * 3 = -35*8

Simplify:

57x = -280

Finally, solve for :

$x = -\frac{280}{57}$

Report an Error

Example Question #23 : Algebraic Fractions

Evaluate when x=11. Round to the nearest tenth.

Possible Answers:

1.8

1.9

0.2

0.3

Correct answer:

1.8

Explanation:

Wherever there is an x, plug in 11 and perform the given operations. The numerator will be equal to 83 and the denominator will be equal to 46. 83 divided by 46 is equal to 1.804… and since the second decimal place is not greater than or equal to 5, the first decimal place stays the same when rounding so the final answer is 1.8.

Report an Error

Example Question #45 : Algebraic Fractions

Find the inverse equation of:

3y-2x=20

Possible Answers:

$\frac{2x-20}{3}=y$

$\frac{3x-20}{2}=y$

$\frac{1}{2}x+10=y$

$\frac{2x+20}{3}=y$

Correct answer:

$\frac{3x-20}{2}=y$

Explanation:

To solve for an inverse, we switch x and y and solve for y. Doing so yields:

$\frac{3x-20}{2}=y$

Report an Error

Example Question #2 : How To Find Inverse Variation

Find the inverse equation of 5x-4y=18 .

Possible Answers:

$y=\frac{4x+5}{18}$

x=y

$x=\frac{4y+18}{5}$

$y=\frac{4x+18}{5}$

$y=\frac{x+18}{4}$

Correct answer:

$y=\frac{4x+18}{5}$

Explanation:

5x-4y=18

1. Switch the and variables in the above equation.

5y-4x=18

2. Solve for :

5y-4x=18

5y-4x+4x=18+4x

5y=4x+18

$\frac{5y}{5}=\frac{4x+18}{5}$

$y=\frac{4x+18}{5}$

Report an Error

Example Question #3 : How To Find Inverse Variation

$\begin{matrix} & \x\ & \y\ & \\ & \\ & \end{matrix}$ When x=2 , y=18 .

When x=9 , y=4 .

If varies inversely with , what is the value of when x=12 ?

Possible Answers:

y=3

y=1.5

y=6

y=1

y=x

Correct answer:

y=3

Explanation:

If varies inversely with , $y=\frac{K}{x}$ .

1. Using any of the two x,y combinations given, solve for :

Using (2,18) :

$18=\frac{K}{2}$

K=36

2. Use your new equation $y=\frac{36}{x}$ and solve when x=12 :

$y=\frac{36}{12}=3$

Report an Error

Example Question #33 : Algebraic Fractions

x	y

If varies inversely with , what is the value of ?

Possible Answers:

Correct answer:

Explanation:

An inverse variation is a function in the form: xy = k or $y = \frac{k}{x}$ , where is not equal to 0.

Substitute each $\left ( x,y \right )$ in xy = k .

5(4.8) = 24

6.4(3.75) = 24

20(1.2) = 24

Therefore, the constant of variation, , must equal 24. If varies inversely as , must equal 24. Solve for .

3n = 24

n = 8

Report an Error

Example Question #1 : How To Find Inverse Variation

Two numbers and vary inversely, and x=3 when y=8 . If this is true, what is the value of when y =6 ?

Possible Answers:

Correct answer:

Explanation:

If x=3 when y=8 , and the variation is direct, then xy = 24 . Using this, we know that if y=6 , $x= \frac{24}{6} = 4$ .

Report an Error

View ACT Math Tutors

Dejuan
Certified Tutor

University of Illinois at Urbana-Champaign, Bachelor of Science, Electrical Engineering.

View ACT Math Tutors

Leonard
Certified Tutor

Florida State University, Bachelor of Science, Environmental Science.

View ACT Math Tutors

Yucheng
Certified Tutor

The University of Texas at Austin, Bachelor of Science, Mechanical Engineering.

All ACT Math Resources

14 Diagnostic Tests 767 Practice Tests Question of the Day Flashcards Learn by Concept

ACT Math Tutors in Top Cities:

Atlanta ACT Math Tutors, Austin ACT Math Tutors, Boston ACT Math Tutors, Chicago ACT Math Tutors, Dallas Fort Worth ACT Math Tutors, Denver ACT Math Tutors, Houston ACT Math Tutors, Kansas City ACT Math Tutors, Los Angeles ACT Math Tutors, Miami ACT Math Tutors, New York City ACT Math Tutors, Philadelphia ACT Math Tutors, Phoenix ACT Math Tutors, San Diego ACT Math Tutors, San Francisco-Bay Area ACT Math Tutors, Seattle ACT Math Tutors, St. Louis ACT Math Tutors, Tucson ACT Math Tutors, Washington DC ACT Math Tutors

Popular Courses & Classes

LSAT Courses & Classes in San Diego, GMAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Seattle, GRE Courses & Classes in Chicago, GRE Courses & Classes in San Diego, ISEE Courses & Classes in San Francisco-Bay Area, ISEE Courses & Classes in New York City, ACT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Philadelphia

Popular Test Prep

ACT Test Prep in Atlanta, GRE Test Prep in New York City, GRE Test Prep in Dallas Fort Worth, GRE Test Prep in Washington DC, ISEE Test Prep in Dallas Fort Worth, MCAT Test Prep in Washington DC, SSAT Test Prep in Boston, SSAT Test Prep in Seattle, LSAT Test Prep in New York City, GMAT Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ACT Math : Algebraic Fractions

Study concepts, example questions & explanations for ACT Math

All ACT Math Resources

Example Questions

Example Question #33 : Algebraic Fractions

Example Question #34 : Algebraic Fractions

Example Question #31 : Algebraic Fractions

Example Question #32 : Algebraic Fractions

Example Question #23 : Algebraic Fractions

Example Question #45 : Algebraic Fractions

Example Question #2 : How To Find Inverse Variation

Example Question #3 : How To Find Inverse Variation

Example Question #33 : Algebraic Fractions

Example Question #1 : How To Find Inverse Variation

All ACT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: