To add complex numbers, we must combine like terms: real with real, and imaginary with imaginary. 

In order to solve this problem, we must combine real numbers with real numbers and imaginary numbers with imaginary numbers. Be careful to distribute the subtraction sign to all terms in the second set of parentheses.

To solve this problem, make sure you set it up to multiply the entire parentheses by itself (a common mistake it to try to simply distribute the exponent 2 to each of the terms in the parentheses.)

 (recall that )

Please note that while the answer choice  is not incorrect, it is not fully simplified and therefore not the correct choice.

While these terms may not look like they follow the typical format of , don't let them fool you! We can read 5 as  and we can read 3i as . Now recalling that the complex conjugate of  is , we can see that the complex conjugate of  is just  and the complex conjugate of  is 

To perform division on complex numbers, multiple both the numerator and the denominator of the fraction by the complex conjugate of the denominator. This looks like:

To represent complex numbers graphically, we treat the x-axis as the "axis of reals" and the y-axis as the "axis of imaginaries." To plot , we want to move 6 units on the x-axis and -3 units on the y-axis. We can plot the point P to represent , but we can also represent it by drawing a vector from the origin to point P. Both representations are in the diagram below. 

We can take any complex number  and graph it as a vector, measuring  units in the x direction and  units in the y direction. Therefore . Likewise, . Then, we can add these two vectors together, summing their real parts and their imaginary parts to create their resultant vector . Therefore the correct answer is .

The above image is a graphic representation of subtraction of complex numbers (which are represented by vectors  and . We can take any complex number  and graph it as a vector, measuring  units in the x direction and  units in the y direction. Therefore . Likewise, .

To help us visualize subtraction, instead of thinking about taking , we should instead visualize . The below figure shows  with a dotted line. Visually, the resultant vector  lies in between the vectors  and . Algebraically, we get   or  . Either way you think about it, the resulting vector is 

You can draw the following right triangle using the information given by the question:

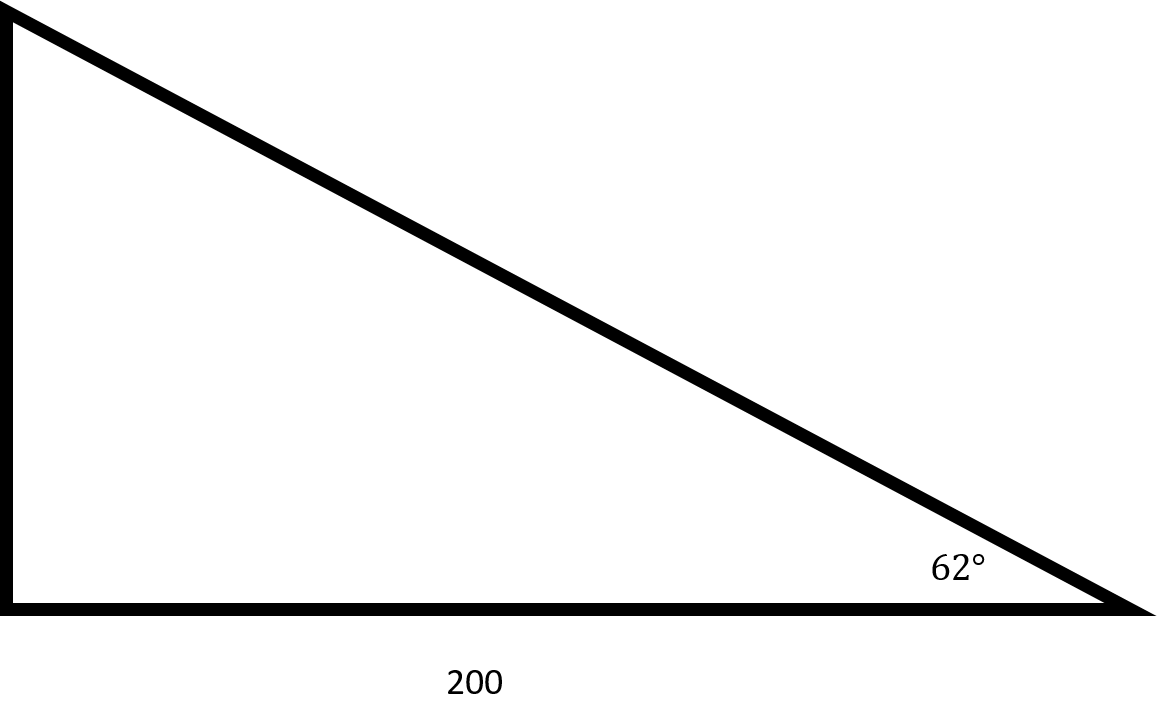
Since you want to find the height of the platform, you will need to use tangent.

Make sure to round to  places after the decimal.

You can draw the following right triangle from the information given by the question.

In order to find the height of the flagpole, you will need to use tangent.

Make sure to round to  places after the decimal.

The flagpole is  feet tall.