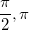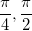All Trigonometry Resources
Example Questions
Example Question #1 : Angles In The Unit Circle
What are the ways to write 360o and 720o in radians?


Example Question #2 : Angles In The Unit Circle
Which of the following is not an angle in the unit circle?
The unit circle is in two increments: 






Example Question #3 : Angles In The Unit Circle
What is the equivalent of 
To convert from degrees to radians, use the equality


From here we use 
Example Question #3 : Angles In The Unit Circle
What is the value of 
To help with this one, draw a 45-45-90 triangle. With legs equal to 1 and a hypotenuse of 
Then, use the definition of tangent as opposite over adjacent to find the value.
Since the legs are congruent, we get that the ratio is 1.
Example Question #4 : Angles In The Unit Circle
What is 
Recall that on the unit circle, sine represents the y coordinate of the unit circle.
Then, since we are at 90˚, we are at the positive y axis, the point (0,1).
At this point on the unit circle, the y value is 1.
Thus 
Example Question #6 : Angles In The Unit Circle
Which of the following is NOT a special angle on the unit circle?
For an angle to be considered a special angle, the angle must be able to produce a 

The only angle that is not capable of the special angles formation is 
Example Question #7 : Angles In The Unit Circle
If 


The solution does not lie in the given interval.
We first make the substitution 
In the interval 


Solving for 

Example Question #3 : Angles In The Unit Circle
What is the value of 
From the unit circle, the value of

This can be found using the coordinate pair associated with the angle 

Recall that the 

Example Question #9 : Angles In The Unit Circle
What is

using the unit circle?
Recall that the unit circle can be broken down into four quadrants. Each quadrant has similar coordinate pairs basic on the angle. The only difference between the actual coordinate pairs is the sign on them. In quadrant I all signs are positive. In quadrant II only sine and cosecant are positive. In quadrant III tangent and cotangent are positive and quadrant IV only cosine and secant are positive.



From the unit circle, the coordinate point of


Example Question #10 : Angles In The Unit Circle
Give the exact value.
Use the unit circle to find:
None of the above
Locate 
Sine is related to the why y value of the coordinate point because it is opposite/Hyp.
In other words the 

The coordinate pair for 

All Trigonometry Resources