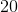All TACHS Math Resources
Example Questions
Example Question #1 : Perimeter Of Triangles, Squares, And Rectangles
Find the perimeter of a square with the following side length:
Perimeters can be calculated by adding together the side lengths of a polygon. A square has four sides that are all the same length, therefore, we can write the following formula to solve for the perimeter.
We can rewrite this equation as the following:
In these equations the variable, 
Substitute the known side length and solve.
Example Question #1 : Perimeter/Circumference
Find the circumference of a circle with the following radius:
In order to find the circumference of a circle we will use the following formula:
In this equation, the variable, 
Substitute in for the circle's radius.
Simplify and solve.
Example Question #1 : Area Of Squares And Rectangles
A square has perimeter 24 feet. Give its area.
36 square feet
576 square feet
144 square feet
18 square feet
36 square feet
The perimeter of a figure is the sum of the lengths of its sides. A square comprises four sides of equal length, so, if the perimeter of the square is 24 feet, then each side has length

The area of the square is equal to the length of a side multiplied by itself, so the area of this square is

Example Question #2 : Area Of Squares And Rectangles
Jennifer wants to wallpaper her room which is made up for four rectangular walls each measuring at 


Start by finding out the total square footage of wallpaper needed.
Find the area of one wall. Recall that in order to find the area of a rectangle, you must multiply the length by the width.
Since we have four identical walls,
Now divide this by the amount of square feet covered by each roll of wallpaper to find how many rolls are needed.
Example Question #1 : Area Of A Circle
Find the area of a circle with the following radius:
The area of a circle can be calculated using the following formula:
In this formula the radius is denoted by the variable, 
Substitute in the known variables and solve for the circle's area.
Example Question #2 : Area Of A Circle
Which is equal to the radius of a circle with area
The formula for the area 




To find the radius 

Now, find the square root of both sides. Since 

The radius of the given circle is 20.
Example Question #3 : Area Of A Circle
To determine whether a machine on an assembly line is filling bottles with the correct amount of soda, twenty bottles are selected. The tenth bottle and every tenth bottle after that are taken off the line and examined.
This is an example of which kind of sampling?
Cluster sampling
Convenience sampling
Systematic sampling
Stratified sampling
Systematic sampling
The sample in this scenario is selected from the population by choosing obects that occur at regular intervals. That makes this an example of systematic sampling.
Example Question #4 : Area Of A Circle
What is the area of a circle that has a diameter of 
Recall how to find the area of a circle:
To find the length of the radius, divide the diameter by two.
Now, plug it into the equation for the area of a circle.
Example Question #1 : Volume
Calculate the volume of a sphere with the following radius:
We can calculate the the volume of a sphere using the following formula:
in this formula the variable, 
Substitute in the known radius and calculate the volume.
Simplify.
Solve.
All TACHS Math Resources