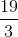All SSAT Upper Level Math Resources
Example Questions
Example Question #1 : Slope
What is the slope of the line that passes through the points 
Use the following formula to find the slope:
Substituting the values from the points given, we get the following slope:
Example Question #201 : Coordinate Geometry
Find the slope of a line that passes through the points 

To find the slope of the line that passes through the given points, you can use the slope equation.
Example Question #2 : Slope
Find the slope of the line that passes through the points 

To find the slope of the line that passes through the given points, you can use the slope equation.
Example Question #201 : Coordinate Geometry
A line has the equation 
You need to put the equation in 
Since 
Example Question #3 : How To Find Slope
Find the slope of the line that goes through the points 

Even though there are variables involved in the coordinates of these points, you can still use the slope formula to figure out the slope of the line that connects them.
Example Question #121 : Grade 8
The equation of a line is 
To find the slope, you will need to put the equation in 

Subtract 
Divide each side by 
You can now easily identify the value of 
Example Question #3 : Slope
Find the slope of the line that passes through the points 

You can use the slope formula to figure out the slope of the line that connects these two points. Just substitute the specified coordinates into the equation and then subtract:
Example Question #8 : How To Find Slope
Find the slope of the following function:
Rewrite the equation in slope-intercept form, 
The slope is the 

Example Question #9 : How To Find Slope
Find the slope of the line given the two points:
Write the formula to find the slope.
Either equation will work. Let's choose the latter. Substitute the points.
Example Question #131 : Grade 8
What is the slope of the line with the equation
To find the slope, put the equation in the form of 
Since 
Certified Tutor
All SSAT Upper Level Math Resources