SSAT Middle Level Math : SSAT Middle Level Quantitative (Math)
Study concepts, example questions & explanations for SSAT Middle Level Math
All SSAT Middle Level Math Resources
Example Questions
Example Question #243 : Geometry
The above depicts a rectangular swimming pool for an apartment. The pool is five feet deep everywhere.
An apartment manager wants to paint the four sides and the bottom of the swimming pool. One one-gallon can of the paint he wants to use covers 
The bottom of the swimming pool has area
There are two sides whose area is
and two sides whose area is
Add the areas:
One one-gallon can of paint covers 350 square feet, so divide:
Seven full gallons and part of another are required, so eight is the correct answer.
Example Question #84 : Quadrilaterals
You are putting in a new carpet in your living room. The dimensions of the the room are 
To find the area of a rectangle, you must multiply the two different side lengths. For this room the answer would be 
Example Question #82 : Quadrilaterals
Refer to the above figures. The square at left has area 160. Give the area of the rectangle at right.
The area of the square, whose sides have length 
The square and the rectangle have the same area, so the correct response is 160.
Example Question #41 : How To Find The Area Of A Rectangle
Figure NOT drawn to scale.
Figure 1 and Figure 2 have the same area. The shaded portion of Figure 1 has area 64. What is the area of the shaded portion of Figure 2?
Figure 1 is a rectangle divided into 24 squares of equal size; 3 of the squares are shaded, which means that 
Figure 2 is a circle divided into 8 sectors of equal size; 1 is shaded, which means that 
Since the two figures are of the same area, the two shaded portions, each of which have an area that is the same fraction of this common area, must themselves have the same area. Since the shaded portion of Figure 1 has area 64, so does the shaded portion of Figure 2.
Example Question #351 : Ssat Middle Level Quantitative (Math)
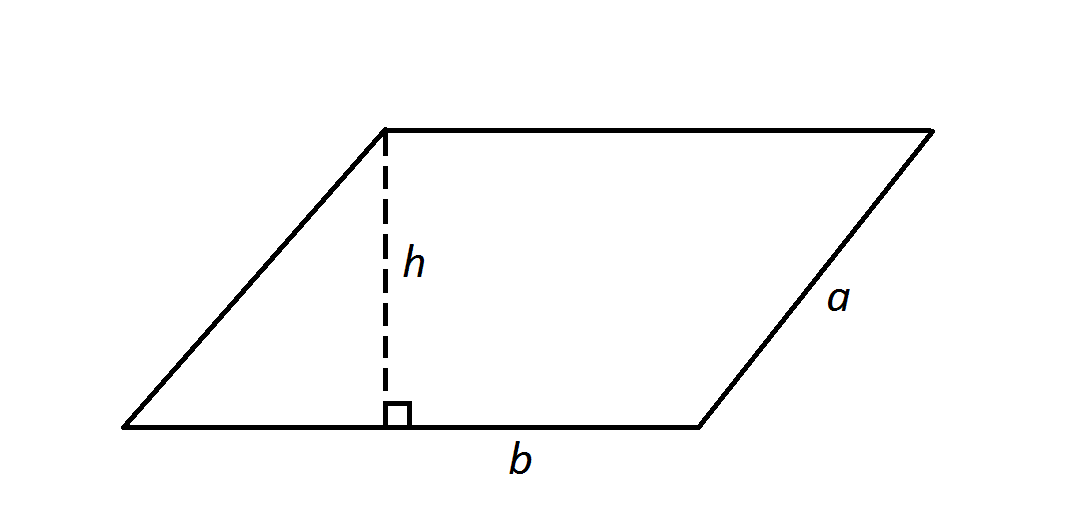
Note: Figure NOT drawn to scale
In the above diagram,
Give the area of the parallelogram.
The area of a parallelogram is its base multiplied by its height - represented by 
Note that the value of 
Example Question #2 : Parallelograms
A given parallelogram has a base 
The formula for the area of a parallelogram is 
Example Question #351 : Ssat Middle Level Quantitative (Math)
A parallelogram has a height of 
Given base 
Substituting the values from our question:
Example Question #3 : Parallelograms
A parallelogram has a base of length 
Given base
Substituting the values from our question:
Example Question #4 : Parallelograms
Find the area of a parallelogram with a height of 
The area 
Example Question #3 : How To Find The Area Of A Parallelogram
Find the area of a parallelogram with a height of 
The area 
Certified Tutor
Certified Tutor
All SSAT Middle Level Math Resources
