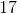Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Middle Level Math : How to find range

Study concepts, example questions & explanations for SSAT Middle Level Math

Create An Account

All SSAT Middle Level Math Resources

10 Diagnostic Tests 225 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Find Measures Of Center, Variability, And Patterns In Data: Ccss.Math.Content.6.Sp.B.5c

The difference between 2996 and 4515 is closest to

Possible Answers:

1500 $\displaystyle 1500$

2000 $\displaystyle 2000$

1000 $\displaystyle 1000$

2500 $\displaystyle 2500$

3000 $\displaystyle 3000$

Correct answer:

1500 $\displaystyle 1500$

Explanation:

Round 2996 up to 3000 and 4515 down to 4500. The difference between 4500 and 3000 is 1500.

Report an Error

Example Question #1 : Find Range

Find the range of this set of numbers:

89, 54, 76, 89, 94.

Possible Answers:

$\displaystyle 40$

$\displaystyle 78$

$\displaystyle 54$

$\displaystyle 89$

$\displaystyle 94$

Correct answer:

$\displaystyle 40$

Explanation:

First, order the numbers from least to greatest, then find the difference of the highest number and the lowest number.

$\displaystyle 54, 76, 89, 89, 94$

$\displaystyle 94-54=40$

Answer: The range of this set of numbers is 40.

Report an Error

Example Question #1 : Find Range

Find the range of this set of numbers:

525, 436, 142, 213, 641.

Possible Answers:

436 $\displaystyle 436$

428 $\displaystyle 428$

499 $\displaystyle 499$

391 $\displaystyle 391$

Correct answer:

499 $\displaystyle 499$

Explanation:

First, order the numbers from least to greatest. Then, find the difference between the largest and the smallest number.

$\displaystyle 142, 213, 436, 525, 641.$

$\displaystyle 641-142=499$

Answer: The range is 499. $\displaystyle 499.$

Report an Error

Example Question #1 : Find Range

What is the range of the set of numbers $\displaystyle 45$ , $\displaystyle 6$ , $\displaystyle 32$ , $\displaystyle 11$ , $\displaystyle 2$ ?

Possible Answers:

$\displaystyle 34$

$\displaystyle 26$

$\displaystyle 30$

$\displaystyle 43$

19.2 $\displaystyle 19.2$

Correct answer:

$\displaystyle 43$

Explanation:

The range of a set of numbers is the difference between the largest and smallest numbers. In this set, $\displaystyle 45$ is the largest number and $\displaystyle 2$ is the smallest number. The range, or difference, between these two numbers, is $\displaystyle 43$ .

Report an Error

Example Question #2 : Find Range

Find the range of this set of numbers:

$\displaystyle 546, 321, 789, 601, 751$

Possible Answers:

468 $\displaystyle 468$

602 $\displaystyle 602$

430 $\displaystyle 430$

601 $\displaystyle 601$

Correct answer:

468 $\displaystyle 468$

Explanation:

First order the numbers in order from least to greatest:

$\displaystyle 321, 546, 601, 751, 789$

Then, find the difference of the largest and smallest number in the set:

$\displaystyle 789-321=468.$

Answer: The range is 468 $\displaystyle 468$

Report an Error

Example Question #2 : Find Range

What is the range of the set of numbers:

$\displaystyle 106, 94, 95, 89, 102$

Possible Answers:

$\displaystyle 17$

$\displaystyle 16$

106 $\displaystyle 106$

$\displaystyle 89$

$\displaystyle 15$

Correct answer:

$\displaystyle 17$

Explanation:

The ragne of a set of numbers is the difference between the largest and smallest numbers. In this set, 106 $\displaystyle 106$ is the largest number and $\displaystyle 89$ is the smallest number. The range, or difference, between these two numbers, is $\displaystyle 106-89=17$ .

Report an Error

Example Question #2 : Find Range

What is the range of the following data set?

$\small 1321, 1472, 1731, 1137, 1820$ $\displaystyle \small 1321, 1472, 1731, 1137, 1820$

Possible Answers:

$\small 1472$ $\displaystyle \small 1472$

$\small 683$ $\displaystyle \small 683$

$\small 1496$ $\displaystyle \small 1496$

544 $\displaystyle 544$

$\small 499$ $\displaystyle \small 499$

Correct answer:

$\small 683$ $\displaystyle \small 683$

Explanation:

The range of a data set is equal to the difference between the largest $\small (1820)$ $\displaystyle \small (1820)$ and the smallest $\small (1137)$ $\displaystyle \small (1137)$ numbers.

$\small range=1820-1137=683$ $\displaystyle \small range=1820-1137=683$

Report an Error

Example Question #1 : Find Range

Find the range of this set of numbers:

$\displaystyle 57,59,51,53,54$

Possible Answers:

$\displaystyle 8$

$\displaystyle 7$

$\displaystyle 54$

$\displaystyle 57$

Correct answer:

$\displaystyle 8$

Explanation:

First order the numbers from least to greatest:

$\displaystyle 51, 53, 54, 57, 59$

Then subtract the smallest number from the largest number:

59-51=8 $\displaystyle 59-51=8$

Answer: $\displaystyle 8$

Report an Error

Example Question #2 : Find Range

Given the following set of numbers, find the range.

$\textup{3, 8, 12, -5, 18, -7, 12, 2, 9}$ $\displaystyle \textup{3, 8, 12, -5, 18, -7, 12, 2, 9}$

Possible Answers:

$\displaystyle 18$

$\displaystyle 25$

$\displaystyle -7$

$\displaystyle 12$

Correct answer:

$\displaystyle 25$

Explanation:

In order to find the range for a set of data, take the largest number and subtract the smallest number.

In this case, the largest number is $\displaystyle 18$ and the smallest number is $\displaystyle -7$ .

Remember that when we subtract a negative, we are actually adding the absolute value of the numbers:

Therefore:

$\displaystyle 18 - (-7)$ is the same as 18 + 7 $\displaystyle 18 + 7$

18+7=25 $\displaystyle 18+7=25$

Report an Error

Example Question #2 : How To Find Range

Use the following data set to answer the question:

$\displaystyle 4, 8, 6, 10, 4, 9, 5, 2, 4$

Find the range.

Possible Answers:

$\displaystyle 10$

$\displaystyle 8$

$\displaystyle 6$

$\displaystyle 5$

$\displaystyle 4$

Correct answer:

$\displaystyle 8$

Explanation:

To find the range, we will find the smallest number and the largest number. Then, we will find the difference of those two numbers.

So, given the set

$\displaystyle 4, 8, 6, 10, 4, 9, 5, 2, 4$

we can see the smallest number is 2 and the largest number is 10. Now, we will find the difference. We get

10-2=8 $\displaystyle 10-2=8$

Therefore, the range of the data set is 8.

Report an Error

View SSAT- Middle Level Tutors

Jason
Certified Tutor

NJCU, Bachelor in Arts, Communication, General. Saint Peters College, Masters in Business Administration, Elementary and Midd...

View SSAT- Middle Level Tutors

Cheryl
Certified Tutor

Palm Beach Atlantic University-West Palm Beach, Bachelor of Science, Business Administration and Management. Southern New Ham...

View SSAT- Middle Level Tutors

Martine
Certified Tutor

University of South Florida-Main Campus, Bachelors, Science. Windsor University School of Medicine, Doctor of Medicine, Scien...

All SSAT Middle Level Math Resources

10 Diagnostic Tests 225 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Statistics Tutors in Denver, GRE Tutors in Houston, LSAT Tutors in Phoenix, Algebra Tutors in Philadelphia, Chemistry Tutors in Miami, SSAT Tutors in Washington DC, Computer Science Tutors in Miami, Statistics Tutors in Houston, SSAT Tutors in Los Angeles, Algebra Tutors in Washington DC

Popular Courses & Classes

GMAT Courses & Classes in Atlanta, SAT Courses & Classes in Denver, GMAT Courses & Classes in Miami, ACT Courses & Classes in Miami, MCAT Courses & Classes in Houston, GRE Courses & Classes in Boston, GMAT Courses & Classes in Seattle, Spanish Courses & Classes in New York City, ACT Courses & Classes in Chicago, LSAT Courses & Classes in Boston

Popular Test Prep

MCAT Test Prep in Los Angeles, SAT Test Prep in Phoenix, SSAT Test Prep in Dallas Fort Worth, ISEE Test Prep in San Diego, LSAT Test Prep in Atlanta, ACT Test Prep in Chicago, SAT Test Prep in Philadelphia, SSAT Test Prep in Chicago, ACT Test Prep in Miami, LSAT Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Middle Level Math : How to find range

Study concepts, example questions & explanations for SSAT Middle Level Math

All SSAT Middle Level Math Resources

Example Questions

Example Question #1 : Find Measures Of Center, Variability, And Patterns In Data: Ccss.Math.Content.6.Sp.B.5c

Example Question #1 : Find Range

Example Question #1 : Find Range

Example Question #1 : Find Range

Example Question #2 : Find Range

Example Question #2 : Find Range

Example Question #2 : Find Range

Example Question #1 : Find Range

Example Question #2 : Find Range

Example Question #2 : How To Find Range

All SSAT Middle Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: