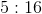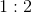SAT Math : Arithmetic
Study concepts, example questions & explanations for SAT Math
All SAT Math Resources
Example Questions
Example Question #3 : How To Find A Ratio
The ratio of 
What is the ratio of 
Since the ratios are fixed, regardless of the actual values of 
In order to convert to a form where we can relate 
Thus, we now have 
Setting the 
Example Question #21 : How To Find A Ratio
The exchange rate in some prehistoric village was 
We can use dimensional analysis to solve this problem. We will create ratios from the conversions given.
Since Joaquin cannot trade for part of a shiny rock, the most he can get is 3 shiny rocks.
Example Question #501 : Arithmetic
In a flower bed, Joaquin plants 
First, we should write a fraction for each ratio given:
Next, we will multiply these fractions by each other in such a way that will leave us with a fraction that has only Z and M, since we want a ration of these two flowers only.
So the final answer is 35:6
Example Question #23 : How To Find A Ratio
Solve for 
To solve for the missing value in this ratio problem, it is a two step process.
First cross-multiply:
From here, to isolate x take the opposite operation. In other words divide each side by two.
Example Question #1 : Fractions
A lawn can be mowed by 
The number of hours required to mow the lawn remains constant and can be found by taking the original 
Example Question #1 : How To Find Rate
A family is on a road trip from Cleveland to Virginia Beach, totaling 600 miles. If the first half of the trip is completed in 6.5 hours and the second half of the trip is completed in 5.5 hours, what is the average speed in miles per hour of the whole trip?
60 mph
50 mph
45 mph
55 mph
65 mph
50 mph
Take the total distance travelled (600 miles) and divide it by the total time travelled (6.5 hrs + 5.5 hrs = 12 hours) = 50 miles/hour
Example Question #2 : How To Find Rate
Two electric cars begin moving on circular tracks at exactly 1:00pm. If the first car takes 30 minutes to complete a loop and the second car takes 40 minutes, what is the next time they will both be at the starting point?
1:35 p.m.
4:00 p.m.
2:40 p.m.
3:30 p.m.
3:00 p.m.
3:00 p.m.
Call the cars “Car A” and “Car B”.
The least common multiple for the travel time of Car A and Car B is 120. We get the LCM by factoring. Car A’s travel time gives us 3 * 2 * 5; Car B’s time gives us 2 * 2 * 2 * 5. The smallest number that accommodates all factors of both travel times is 2 * 2 * 2 * 3 * 5, or 120. There are 60 minutes in an hour, so 120 minutes equals two hours. Two hours after 1:00pm is 3:00pm.
Example Question #2 : How To Find Rate
If Jon is driving his car at ten feet per second, how many feet does he travel in 30 minutes?
5,800
18,000
12,000
600
1800
18,000
If Jon is driving at 10 feet per second he covers 10 * 60 feet in one minute (600 ft/min). In order to determine how far he travels in thirty minutes we must multiply 10 * 60 * 30 feet in 30 minutes.
Example Question #2 : How To Find Rate
An arrow is launched at 10 meters per second. If the arrow flies at a constant velocity for an hour, how far has the arrow gone?
100 meters
3600 meters
36,000 meters
600 meters
36,000 meters
There are 60 seconds in a minute and 60 minutes in an hour, therefore 3600 seconds in an hour. The arrow will travel 3600x10= 36,000 meters in an hour.
Example Question #2 : How To Find Rate
If Jack ran at an average rate of 7 miles per hour for a 21 mile course, and Sam ran half as fast for the same distance, how much longer did it take for Sam to run the course than Jack?
1 hour
4 hours
2.5 hours
3 hours
2 hours
3 hours
Using the rate formula: Distance = Rate x Time,
Since Jack’s speed was 7 mph, Jack completed the course in 3 hours
21 = 7 x t
t = 3
Sam’s speed was half of Jack’s speed: 7/2 = 3.5
21 = 3.5 x t
t = 6
Therefore it took Sam 3 hours longer to run the course.
All SAT Math Resources
