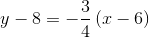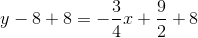Call Now to Set Up Tutoring:

(888) 888-0446

SAT II Math II : Graphing Linear Functions

Study concepts, example questions & explanations for SAT II Math II

Create An Account

All SAT II Math II Resources

2 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Graphing Linear Functions

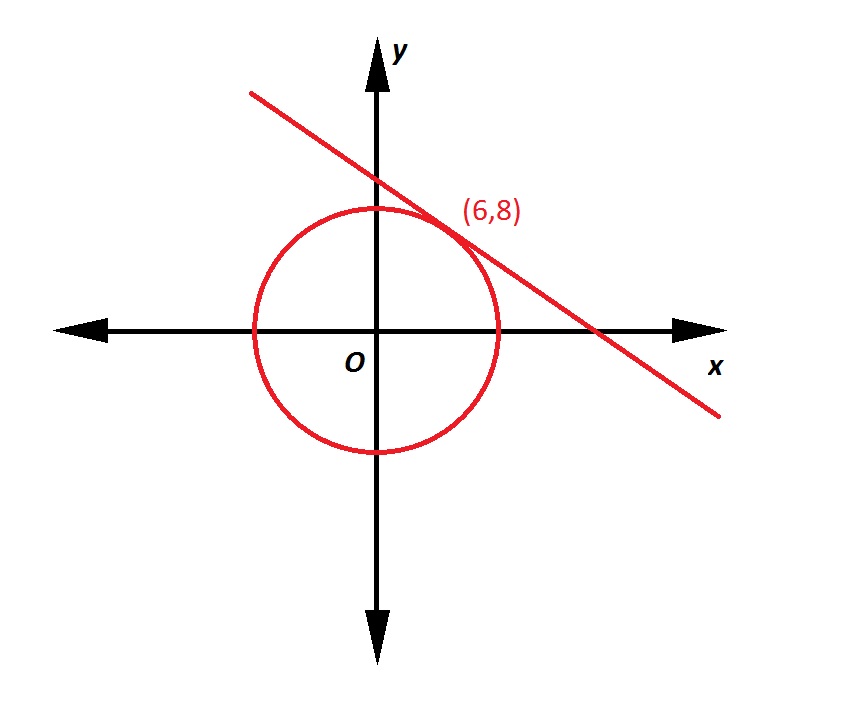
Circle

Note: Figure NOT drawn to scale.

Refer to the above figure. The circle has its center at the origin; the line is tangent to the circle at the point indicated. What is the equation of the line in slope-intercept form?

Possible Answers:

Insufficient information is given to determine the equation of the line.

$y = \frac{3}{4} x+ \frac{7}{2}$ $\displaystyle y = \frac{3}{4} x+ \frac{7}{2}$

$y = \frac{4}{3} x$ $\displaystyle y = \frac{4}{3} x$

$y = - \frac{3}{4} x+ \frac{25}{2}$ $\displaystyle y = - \frac{3}{4} x+ \frac{25}{2}$

$y = - \frac{4}{3} x+ 16$ $\displaystyle y = - \frac{4}{3} x+ 16$

Correct answer:

$y = - \frac{3}{4} x+ \frac{25}{2}$ $\displaystyle y = - \frac{3}{4} x+ \frac{25}{2}$

Explanation:

A line tangent to a circle at a given point is perpendicular to the radius from the center to that point. That radius, which has endpoints $\displaystyle (0,0),(6,8)$ , has slope

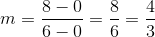
$m = \frac{8-0}{6-0} = \frac{8}{6} = \frac{4}{3}$ $\displaystyle m = \frac{8-0}{6-0} = \frac{8}{6} = \frac{4}{3}$ .

The line, being perpendicular to this radius, will have slope equal to the opposite of the reciprocal of that of the radius. This slope will be $-\frac{3}{4}$ $\displaystyle -\frac{3}{4}$ . Since it includes point (6,8) $\displaystyle (6,8)$ , we can use the point-slope form of the line to find its equation:

$y - y_{1} = m(x-x_{1})$ $\displaystyle y - y_{1} = m(x-x_{1})$

$y - 8= - \frac{3}{}4\left ( x-6 \right )$ $\displaystyle y - 8= - \frac{3}{}4\left ( x-6 \right )$

$y - 8= - \frac{3}{4} x+ \frac{9}{2}$ $\displaystyle y - 8= - \frac{3}{4} x+ \frac{9}{2}$

$y - 8+ 8 = - \frac{3}{4} x+ \frac{9}{2} + 8$ $\displaystyle y - 8+ 8 = - \frac{3}{4} x+ \frac{9}{2} + 8$

$y = - \frac{3}{4} x+ \frac{25}{2}$ $\displaystyle y = - \frac{3}{4} x+ \frac{25}{2}$

Report an Error

View SAT Subject Test in Mathematics Level 2 Tutors

Misty
Certified Tutor

Lewis-Clark State College, Bachelor in Business Administration, Business Communications. Washington State University, Masters...

View SAT Subject Test in Mathematics Level 2 Tutors

Shakeel
Certified Tutor

View SAT Subject Test in Mathematics Level 2 Tutors

Rodolfo
Certified Tutor

The University of Texas at San Antonio, Bachelor of Science, Mathematics.

All SAT II Math II Resources

2 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Algebra Tutors in Seattle, Algebra Tutors in New York City, ACT Tutors in Houston, Statistics Tutors in San Diego, Calculus Tutors in Houston, Reading Tutors in Seattle, MCAT Tutors in Boston, Chemistry Tutors in New York City, Algebra Tutors in Dallas Fort Worth, GMAT Tutors in Philadelphia

Popular Courses & Classes

SSAT Courses & Classes in Atlanta, ACT Courses & Classes in Seattle, Spanish Courses & Classes in Denver, GMAT Courses & Classes in Chicago, GRE Courses & Classes in Los Angeles, LSAT Courses & Classes in San Diego, Spanish Courses & Classes in Philadelphia, SSAT Courses & Classes in Boston, MCAT Courses & Classes in Chicago, ISEE Courses & Classes in Houston

Popular Test Prep

GMAT Test Prep in Dallas Fort Worth, SSAT Test Prep in Houston, ACT Test Prep in San Francisco-Bay Area, LSAT Test Prep in Boston, GRE Test Prep in San Diego, ISEE Test Prep in Miami, GMAT Test Prep in Chicago, SSAT Test Prep in Washington DC, LSAT Test Prep in Los Angeles, ISEE Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT II Math II : Graphing Linear Functions

Study concepts, example questions & explanations for SAT II Math II

All SAT II Math II Resources

Example Questions

Example Question #1 : Graphing Linear Functions

All SAT II Math II Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: