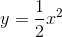All SAT II Math II Resources
Example Questions
Example Question #1 : Parabolas
Which of the following equations represent a parabola?
The parabola is represented in the form 
The only equation that has an order of two is:
Example Question #121 : Geometry
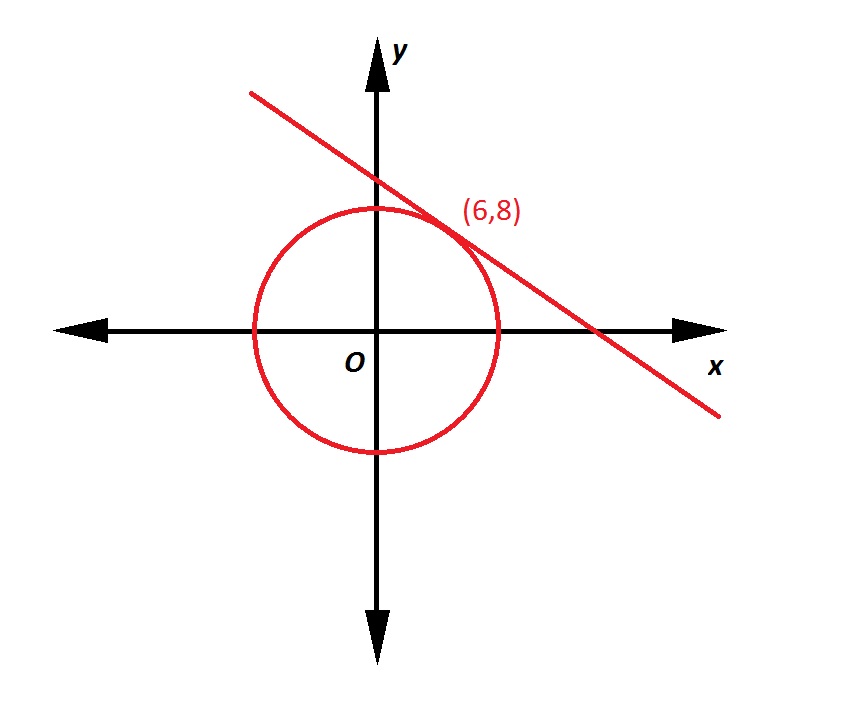
Refer to the above figure. The circle has its center at the origin. What is the equation of the circle?
The equation of a circle with center 

The center is at the origin, or 


Note that we do not actually need to find 
We can now write the equation of the circle:
Example Question #122 : Geometry

Refer to the above diagram. The circle has its center at the origin; 


First, it is necessary to determine the radius of the circle. This is the distance between 

The circumference of the circle is
Now we need to find the degree measure of the arc. We can do this best by examining this diagram:

The degree measure of 

Using a calculator, we find that 

which is the degree measure of the arc.
Now we can calculate the length of the arc:
Example Question #4 : Coordinate Geometry
On the coordinate plane, the vertices of a square are at the points with coordinates 
The figure in question is below.

The center of the circle can be seen to be the origin, so, if the radius is 

The circle passes through the midpoints of the sides, so we will find one of these midpoints. The midpoint 



The circle passed though this midpoint 



set 
Substituting in the circle equation for 
Example Question #121 : Geometry
Find the diameter of the circle with the equation 
Start by putting the equation in the standard form of the equation of a circle by completing the square. Recall the standard form of the equation of a circle:



From the equation, we know that 
Since the radius is 

Example Question #431 : Sat Subject Test In Math Ii
A triangle has its vertices at the points with coordinates 


None of these
The circumscribed circle of a triangle is the circle which passes through all three vertices of the triangle.
In general form, the equation of a circle is

Since the circle passes through the origin, substitute 
Therefore, we know the equation of any circle passing through the origin takes the form
for some 
Since the circle passes through 

Solving for 
Now we know that the equation takes the form

for some 
Since the circle passes through 

Solving for 
The general form of the equation of the circle is therefore
Example Question #1 : Symmetry
Which of the following symmetries applies to the graph of the relation

I) Symmetry with respect to the origin
II) Symmetry with respect to the 
III) Symmetry with respect to the 
None of these
I, II, and III
III only
II only
I only
II only
The relation
is a circle with center 

In other words, it is a circle with center at the origin, translated right 

or
is a circle translated right 4 units and up zero units. The upshot is that the circle moves along the 


Example Question #1 : Transformations

Refer to the above figure.
Which of the following functions is graphed?
The correct answer is not given among the other responses.
Below is the graph of 

The given graph is the graph of 

The function graphed is therefore


Example Question #431 : Sat Subject Test In Math Ii

Refer to the above figure.
Which of the following functions is graphed?
Below is the graph of 

If the graph of 



is formed. If the graph is then shifted upward by three units, the new graph is
Since the starting graph was 

Example Question #2 : Transformations

Refer to the above figure.
Which of the following functions is graphed?
Below is the graph of 

If the graph of 



is formed. If the graph is then shifted right by four units, the new graph is
Since the starting graph was 

Certified Tutor
All SAT II Math II Resources