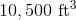All SAT II Math I Resources
Example Questions
Example Question #1 : Volume
A circular swimming pool has diameter 
The pool can be seen as a cylinder with diameter 

Example Question #1 : 3 Dimensional Geometry

The above depicts a rectangular swimming pool for an apartment. 60% of the pool is six feet deep, and the remaining part of the pool is four feet deep. How many cubic feet of water does the pool hold?
None of the other choices gives the correct answer.
None of the other choices gives the correct answer.
The cross-section of the pool is the area of its surface, which is the product of its length and its width:

Since 60% of the pool is six feet deep, this portion of the pool holds

Since the remainder of the pool - 40% - is four feet deep, this portion of the pool holds

Add them together: the pool holds

This answer is not among the choices.
Example Question #1 : 3 Dimensional Geometry
Find the volume of a cube in inches with a side of
Convert the side dimension to inches first before finding the volume.
Write the volume for a cube and substitute the new side to obtain the volume in inches.
Example Question #2 : 3 Dimensional Geometry

Figure not drawn to scale.
What is the volume of the cylinder above?
66.13 in3
56.55 in3
94.25 in3
48.79 in3
52.36 in3
56.55 in3
In order to find the volume of a cylinder, you find the area of the circular top and multiply it by the height.

The volume of the cylinder is 56.55 in3
Example Question #1 : 3 Dimensional Geometry

Figure not drawn to scale
If the volume of the cone above is 47.12 ft3, what is the radius of the base?
3.5ft
3 ft
2 ft
4 ft
5 ft
3 ft
Because we have been given the volume of the cone and have been asked to find the radius of the base of the cone, we must work backwards using the volume formula.

The radius of the base of the cone is 3 ft.
Example Question #3 : 3 Dimensional Geometry

Figure not drawn to scale.
What is the volume of the above image?
32 yd3
64 yd3
84 yd2
42 yd3
42 yd2
42 yd3

You can find the volume of a box by following the equation below:
The surface area of the box is 42 yd3 (remember that volume measurements are cubic units NOT square units)
Example Question #81 : Geometry
A regular tetrahedron is composed of four equilateral triangles. The formula for the volume of a regular tetrahedron is:


Plugging in our values we get:
Example Question #1 : 3 Dimensional Geometry
Find the volume of a tetrahedron with an edge of 
Write the formula for the volume of a tetrahedron.
Substitute in the length of the edge provided in the problem.
Rationalize the denominator.
Example Question #2 : 3 Dimensional Geometry
Find the volume of a tetrahedron with an edge of 
Write the formula for the volume of a tetrahedron.
Substitute in the length of the edge provided in the problem:
Cancel out the 
A square root is being raised to the power of two in the numerator; these two operations cancel each other out. After canceling those operations, reduce the remaining fraction to arrive at the correct answer:
Example Question #1 : 3 Dimensional Geometry
Find the volume of a tetrahedron with an edge of 
Write the formula for finding the volume of a tetrahedron.
Substitute in the edge length provided in the problem.
Cancel out the 

Expand, rationalize the denominator, and reduce to arrive at the correct answer:
Certified Tutor
All SAT II Math I Resources