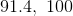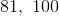The median is middle number of a set, when the numbers are arranged in order from least to greatest.

In our case we re arrange our numbers in the following fashion:

7, 7, 8, 12, 13, 13, 22, 27, 30

The middle number in our case is the 5th entry.

Therefore, the median is 13

The median is the middle number in a set of numbers when arranged in order from least to greatest.

In our case we get

3, 6, 7, 9, 11, 14

This set has two middle numbers 7 and 9. From here we need to find the average between these two numbers to find our median:

$\displaystyle \frac{7+9}{2}=\frac{16}{2}=8$

Median is to find the middle number arranging from smallest to greatest. So we have $\displaystyle 2, 8, 13, 16, 26, 34$. The two middle numbers in the set is $\displaystyle 13, 16$. We then find the average which is $\displaystyle \frac{29}{2}$ or $\displaystyle 14.5$.

The median is the middle number of a set.  If the same amount of values are used and the actual middle number is not changed, the median of the set will not change.  Changing the actual values of the two extremes did not change them position wise in the set when placed in value order.  

The median is the middle value of the set in increasing order.

In this set of 8 (or any even number) entries, the median is the mean of the two middle entries of the set in increasing order

$\displaystyle \frac{7b+9b}{2}$

or

$\displaystyle 8b$

The mean is just the average of all the test scores, which is found by adding up the scores and dividing by the number of scores ($\displaystyle 15$).  This gives $\displaystyle 91.4$ as the mean.  The mode is the score which occurs most frequently.  In this case, the mode is $\displaystyle 89$.  The median, the middle score of the sequence, is $\displaystyle 91$.

Let's think of this list of numbers:

2, 4, 6, ...

Where does it end? The first 5 even numbers goes to 10. That means that the last number in the first 20 will be the number 40. So the question is, "Where is the middle?" Well, this is an even number of values, so there is no actual middle. What we have to do, then is find the 10th and the 11th numbers and take their average. The 10th number is easy, based on what we just said. If the 5th is 10, then the 10th is 20. The 11th will just be two more than that, namely 22. To calculate the median, we just have to find the average of those two numbers:

$\displaystyle \frac{20+22}{2}=21$

If you prefer to write out the full list:

2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30, 32, 34, 36, 38, 40

To answer this question, you need to know the first ten prime numbers! Remember, prime numbers are all of the integers that are divisible only by themselves and by 1.  They do not include 1.  So, our list is:

2, 3, 5, 7, 11, 13, 17, 19, 23, 29

The median is the "middle value." There is no proper "middle" since we have an even number of values. We need to take the 5th and the 6th elements (the middle two values) and average them. The 5th term is 11 and the 6th is 13; therefore, the median is:

$\displaystyle \frac{11+13}{2}=\frac{24}{2}=12$

The easiest way to do this is first to find the total number of flowers:

$\displaystyle 12 +5+4=21$

Now, the median element is the "middle" term. To find the middle, you can divide 21 by 2:

$\displaystyle \frac{21}{2}=10.5$

Since you have an odd number of elements, you unsurprisingly get a fraction. This means that there 10 items to the left of the median and 10 to its right. The 11th term is your median. Now, your group of flowers looks like this:

1-12: 10 inches

13-17: 12 inches

18-21: 15 inches

The 11th item is going to be in that first group, meaning that the median is 10 inches.

If you prefer to write out all of the terms, it will be:

10, 10, 10, 10, 10, 10, 10, 10, 10, 10, 10, 10, 12, 12, 12, 12, 12, 15, 15, 15, 15

The first thing to do is figure out which stalk is in the "middle." Since there are an even number of stalks, there is no exact middle; there are 500 on one side and 500 on the other. This means that the 500th and the 501st are the median. These will have to be averaged.

Now, we need to determine the height of these two stalks. Consider the pattern given:

1st stalk: 10 feet

2nd stalk: 20 feet

3rd stalk: 30 feet

4th stalk: 40 feet

You should see the pattern that emerges for this problem. Each stalk is 10 times that stalk's place in the row. This means that the 500th stalk will be:

$\displaystyle 500*10 =5000$

The 501st stalk will be:

$\displaystyle 501*10=5010$

The average of these two numbers is:

$\displaystyle \frac{5000+5010}{2} = \frac{10010}{2}=5005$

5005 feet is the median.