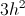Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Acute / Obtuse Triangles

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #567 : Geometry

All of the following could be the possible side lengths of a triangle EXCEPT:

Possible Answers:

6,7,9

3,4,5

2,6,7

5,10,14

5,7,12

Correct answer:

5,7,12

Explanation:

The length of the third side of a triangle must always be between (but not equal to) the sum and the difference of the other two sides.

For instance, take the example of 2, 6, and 7.

6+2=8 and 6-2=4 . Therefore, the third side length must be greater than 4 and less than 8. Because 7 is greater than 4 and less than 8, it is possible for these to be the side lengths of a triangle.

The 5, 7, 12 answer choice is the only option for which this is not the case.

7+5=12 and 7-5=2 . Therefore, the third side length must be between 2 and 12. Because it is equal to the sum, not less than the sum, it is not possible that these could be the side lengths of a triangle.

Report an Error

Example Question #21 : Acute / Obtuse Triangles

$\text{Triangle A}$ and $\text{Triangle B}$ are similar triangles. The perimeter of Triangle A is 45” and the length of two of its sides are 15” and 10”. If the perimeter of Triangle B is 135” and what are lengths of two of its sides?

Possible Answers:

${30}''\textup{ and }{45}''$

${4}''\textup{ and }{6}''$

${20}''\textup{ and }{30}''$

${13}''\textup{ and }{19}''$

Correct answer:

${30}''\textup{ and }{45}''$

Explanation:

The perimeter is equal to the sum of the three sides. In similar triangles, each side is in proportion to its correlating side. The perimeters are also in equal proportion.

Perimeter A = 45” and perimeter B = 135”

The proportion of Perimeter A to Perimeter B is 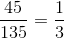$\frac{45}{135}=\frac{1}{3}$ .

This applies to the sides of the triangle. Therefore to get the any side of Triangle B, just multiply the correlating side by 3.

15” x 3 = 45”

10” x 3 = 30“

Screen shot 2016 02 16 at 10.45.30 am

Report an Error

Example Question #352 : Geometry

Find the height of a triangle if the area of the triangle = 18 and the base = 4.

Possible Answers:

Correct answer:

Explanation:

The area of a triangle = (1/2)bh where b is base and h is height. 18 = (1/2)4h which gives us 36 = 4h so h =9.

Report an Error

Example Question #334 : Plane Geometry

If triangle ABC has vertices (0, 0), (6, 0), and (2, 3) in the xy-plane, what is the area of ABC?

Possible Answers:

Correct answer:

Explanation:

Sketching ABC in the xy-plane, as pictured here, we see that it has base 6 and height 3. Since the formula for the area of a triangle is 1/2 * base * height, the area of ABC is 1/2 * 6 * 3 = 9.

Report an Error

Example Question #1 : How To Find The Area Of An Acute / Obtuse Triangle

The height, , of triangle PQR in the figure is one-fourth the length of . In terms of h, what is the area of triangle PQR ?

Possible Answers:

$3h^{2}$

$\frac{1}{2}h^{2}$

$h^{2}$

$2h^{2}$

Correct answer:

$2h^{2}$

Explanation:

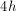
If $\dpi{100} \small h=\frac{1}{4}$ * $\dpi{100} \small \overline{PQ}$ , then the length of $\dpi{100} \small \overline{PQ}$ must be $\dpi{100} \small 4h$ .

Using the formula for the area of a triangle ( $\frac{1}{2}bh$ ), with $\dpi{100} \small b=4h$ , the area of the triangle must be $2h^{2}$ .

Report an Error

View PSAT Mathematics Tutors

Sumit
Certified Tutor

Calcutta University, Bachelor of Science, Mathematics. Indian Statistical Institute, Master of Science, Mathematics.

View PSAT Mathematics Tutors

Trenton
Certified Tutor

United States Military Academy, Bachelor of Science, Mathematics.

View PSAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in Dallas Fort Worth, GRE Tutors in Dallas Fort Worth, Reading Tutors in Atlanta, Calculus Tutors in Atlanta, SAT Tutors in Miami, LSAT Tutors in Miami, Reading Tutors in New York City, English Tutors in Seattle, LSAT Tutors in Chicago, Computer Science Tutors in Houston

Popular Courses & Classes

GRE Courses & Classes in Denver, SAT Courses & Classes in Boston, GMAT Courses & Classes in Miami, GRE Courses & Classes in Los Angeles, Spanish Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Houston, SSAT Courses & Classes in Denver, ACT Courses & Classes in Washington DC, LSAT Courses & Classes in New York City

Popular Test Prep

SAT Test Prep in Chicago, SAT Test Prep in Philadelphia, MCAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Denver, LSAT Test Prep in San Francisco-Bay Area, GRE Test Prep in Dallas Fort Worth, SSAT Test Prep in Denver, ISEE Test Prep in Houston, GMAT Test Prep in Miami, ISEE Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Acute / Obtuse Triangles

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #567 : Geometry

Example Question #21 : Acute / Obtuse Triangles

Example Question #352 : Geometry

Example Question #334 : Plane Geometry

Example Question #1 : How To Find The Area Of An Acute / Obtuse Triangle

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: