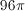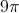All PSAT Math Resources
Example Questions
Example Question #21 : Cylinders
A vase needs to be filled with water. If the vase is a cylinder that is 

Cylinder
Example Question #11 : How To Find The Volume Of A Cylinder
A cylinder has a base diameter of 12 in and is 2 in tall. What is the volume?
The volume of a cylinder is
The diameter is given, so make sure to divide it in half.
The units are inches cubed in this example
Example Question #22 : Cylinders
What is the volume of a cylinder with a radius of 4 and a height of 5?
Example Question #181 : Geometry
Claire's cylindrical water bottle is 9 inches tall and has a diameter of 6 inches. How many cubic inches of water will her bottle hold?
The volume is the area of the base times the height. The area of the base is 
Example Question #14 : How To Find The Volume Of A Cylinder
What is the volume of a circular cylinder whose height is 8 cm and has a diameter of 4 cm?
The volume of a circular cylinder is given by 


Example Question #13 : How To Find The Volume Of A Cylinder
You have tall glass with a radius of 3 inches and height of 6 inches. You have an ice cube tray that makes perfect cubic ice cubes that have 0.5 inch sides. You put three ice cubes in your glass. How much volume do you have left for soda? The conversion factor is 
First we will calculate the volume of the glass. The volume of a cylinder is
Now we will calculate the volume of one ice cube:
The volume of three ice cubes is 
Now we will use our conversion factor:
Example Question #311 : Psat Mathematics
A water glass has the shape of a right cylinder. The glass has an interior radius of 2 inches, and a height of 6 inches. The glass is 75% full. What is the volume of the water in the glass (in cubic inches)?
The volume of a right cylinder with radius 

Since the glass is only 75% full, only 75% of the interior volume of the glass is occupied by water. Therefore the volume of the water is:
Example Question #101 : Solid Geometry
A circle has a circumference of 

Using the circumference, we can find the radius of the circle. The equation for the circumference is 
Now we can find the area of the circle using 

Finally, the surface area consists of the area of two circles and the area of the mid-section of the cylinder: 

Thus, 

Example Question #131 : Solid Geometry
A metal cylindrical brick has a height of 


To find the final volume, we will need to subtract the volume of the hole from the total initial volume of the cylinder.
The volume of a cylinder is given by the product of the base area times the height: 
Find the initial volume using the given base area and height.
Next, find the volume of the hole that was drilled. The base area of this cylinder can be calculated from the radius of the hole. Remember that the height of the hole is only half the height of the block.
Finally, subtract the volume of the hole from the total initial volume.
Example Question #132 : Solid Geometry
What is the volume of a cylinder with a diameter of 13 inches and a height of 27.5 inches?
The equation for the volume of a cylinder is V = Ah, where A is the area of the base and h is the height.
Thus, the volume can also be expressed as V = πr2h.
The diameter is 13 inches, so the radius is 13/2 = 6.5 inches.
Now we can easily calculate the volume:
V = 6.52π * 27.5 = 1161.88π in3
Certified Tutor
Certified Tutor
All PSAT Math Resources