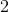PSAT Math : How to find the area of a trapezoid
Study concepts, example questions & explanations for PSAT Math
All PSAT Math Resources
Example Questions
Example Question #1 : Trapezoids
A trapezoid has a base of length 4, another base of length s, and a height of length s. A square has sides of length s. What is the value of s such that the area of the trapezoid and the area of the square are equal?
In general, the formula for the area of a trapezoid is (1/2)(a + b)(h), where a and b are the lengths of the bases, and h is the length of the height. Thus, we can write the area for the trapezoid given in the problem as follows:
area of trapezoid = (1/2)(4 + s)(s)
Similarly, the area of a square with sides of length a is given by a2. Thus, the area of the square given in the problem is s2.
We now can set the area of the trapezoid equal to the area of the square and solve for s.
(1/2)(4 + s)(s) = s2
Multiply both sides by 2 to eliminate the 1/2.
(4 + s)(s) = 2s2
Distribute the s on the left.
4s + s2 = 2s2
Subtract s2 from both sides.
4s = s2
Because s must be a positive number, we can divide both sides by s.
4 = s
This means the value of s must be 4.
The answer is 4.
Example Question #4 : Quadrilaterals
Note: Figure NOT drawn to scale.
The white region in the above diagram is a trapezoid. What percent of the above rectangle, rounded to the nearest whole percent, is blue?
The area of the entire rectangle is the product of its length and width, or
The area of the white trapezoid is one half the product of its height and the sum of its base lengths, or
Therefore, the blue polygon has area
This is
Rounded, this is 70%.
Example Question #1 : Trapezoids
Refer to the above diagram. 
Give the area of Quadrilateral 
By the Angle-Angle Similarity Postulate, since
and
and since corresponding sides of similar triangles are in proportion,
The smaller base is 
The area of the trapezoid is
All PSAT Math Resources
