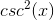We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Precalculus : Trigonometric Identities

Study concepts, example questions & explanations for Precalculus

Create An Account

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Sum And Difference Identities

According to the trigonometric identities, $\displaystyle 1+cot^2(x)=?$

Possible Answers:

tan(x) $\displaystyle tan(x)$

$\frac{sin(x)}{cos(x)}$ $\displaystyle \frac{sin(x)}{cos(x)}$

$\frac{1}{tan(x)}$ $\displaystyle \frac{1}{tan(x)}$

$\displaystyle csc^2(x)$

$\displaystyle 1$

Correct answer:

$\displaystyle csc^2(x)$

Explanation:

The trigonometric identity $\displaystyle 1+cot^2(x)=csc^2(x)$, is an important identity to memorize.

Some other identities that are important to know are:

$\frac{sin(x)}{cos(x)}=tan(x)$ $\displaystyle \frac{sin(x)}{cos(x)}=tan(x)$

$cot(x)=\frac{1}{tan(x)}$ $\displaystyle cot(x)=\frac{1}{tan(x)}$

$\displaystyle sin^2(x)+cos^2(x)=1$

Report an Error

Example Question #1 : Sum And Difference Identities

Use the sum or difference identity to find the exact value: $sin165^\circ$ $\displaystyle sin165^\circ$

Possible Answers:

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

$\frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}-\sqrt{2}}{4}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{\sqrt{6}+\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}+\sqrt{2}}{4}$

$\frac{\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{6}}{4}$

Correct answer:

$\frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}-\sqrt{2}}{4}$

Explanation:

Again, here we break up the 165 into 135+30 $\displaystyle 135+30$ and solve using the sin identity: $sin135\cdot cos30+cos135\cdot sin30\rightarrow \frac{\sqrt{2}}{2}\cdot \frac{\sqrt{3}}{2}+\frac{-\sqrt{2}}{2}\cdot \frac{1}{2}\rightarrow \frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle sin135\cdot cos30+cos135\cdot sin30\rightarrow \frac{\sqrt{2}}{2}\cdot \frac{\sqrt{3}}{2}+\frac{-\sqrt{2}}{2}\cdot \frac{1}{2}\rightarrow \frac{\sqrt{6}-\sqrt{2}}{4}$

Report an Error

Example Question #1 : Trigonometric Identities

Is the following equation an identity? $sin(x-\pi)=sin(x)$ $\displaystyle sin(x-\pi)=sin(x)$

Possible Answers:

It cannot be determined from the given information.

Yes it is an identity.

No it is not an identity.

Correct answer:

No it is not an identity.

Explanation:

$sin(x-\pi)=sinx\rightarrow sinxcos\pi-cosxsin\pi=sinx\rightarrow sinx\cdot (-1)-cosx\cdot (0)\rightarrow -sinx-0=sinx\rightarrow -sinx\neq sinx$ $\displaystyle sin(x-\pi)=sinx\rightarrow sinxcos\pi-cosxsin\pi=sinx\rightarrow sinx\cdot (-1)-cosx\cdot (0)\rightarrow -sinx-0=sinx\rightarrow -sinx\neq sinx$

and so this is again not an identity

Report an Error

Example Question #1 : Sum And Difference Identities

Is the following equation an identity? $\sin \left ( x-\pi \right )=\sin \left ( x \right )$ $\displaystyle \sin \left ( x-\pi \right )=\sin \left ( x \right )$?

Possible Answers:

No it is not an identity

The question cannot be answered based on the information provided.

Yes it is an identity

Correct answer:

No it is not an identity

Explanation:

$\sin \left ( x-\pi \right )=\sin x\rightarrow \sin x\cos \pi -\cos x\sin \pi = \sin x\rightarrow \sin x\cdot \left ( -1 \right )-\cos x\cdot \left ( 0 \right )\rightarrow -\sin x-0=\sin x\neq \sin x$ $\displaystyle \sin \left ( x-\pi \right )=\sin x\rightarrow \sin x\cos \pi -\cos x\sin \pi = \sin x\rightarrow \sin x\cdot \left ( -1 \right )-\cos x\cdot \left ( 0 \right )\rightarrow -\sin x-0=\sin x\neq \sin x$

Therefore, this is not an identity.

Report an Error

Example Question #1 : Sum And Difference Identities For Cosine

Use the sum or difference identity to find the exact value: $cos255^\circ$ $\displaystyle cos255^\circ$

Possible Answers:

$\frac{-1}{2}$ $\displaystyle \frac{-1}{2}$

$\frac{\sqrt{2}+\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}+\sqrt{6}}{4}$

$\frac{2\sqrt{2}}{4}$ $\displaystyle \frac{2\sqrt{2}}{4}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

Correct answer:

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

Explanation:

Using the identity, we can break up the 255 into and then solve: $cos300\cdot cos45+sin300\cdot sin45\rightarrow \frac{1}{2}\cdot \frac{\sqrt{2}}{2}+\frac{-\sqrt{3}}{2}\cdot \frac{\sqrt{2}}{2}\rightarrow \frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle cos300\cdot cos45+sin300\cdot sin45\rightarrow \frac{1}{2}\cdot \frac{\sqrt{2}}{2}+\frac{-\sqrt{3}}{2}\cdot \frac{\sqrt{2}}{2}\rightarrow \frac{\sqrt{2}-\sqrt{6}}{4}$

Report an Error

Example Question #1 : Trigonometric Identities

Is the following equation an identity? $cos(x-\pi)=cos(x)$ $\displaystyle cos(x-\pi)=cos(x)$

Possible Answers:

Yes it is an identity.

No it is not an identity.

It cannot be determined from the given information.

Correct answer:

No it is not an identity.

Explanation:

$cos(x-\pi)=cos(x)\rightarrow cosxcos\pi+sinxsin\pi=cosx\rightarrow cosx(-1)+sinx(0)=cosx\rightarrow -cosx\neq cosx$ $\displaystyle cos(x-\pi)=cos(x)\rightarrow cosxcos\pi+sinxsin\pi=cosx\rightarrow cosx(-1)+sinx(0)=cosx\rightarrow -cosx\neq cosx$

and due to this inequality, this is not an identity

Report an Error

Example Question #1 : Sum And Difference Identities

Use the sum or difference identity to find the exact value: $\cos 255^{\circ}$ $\displaystyle \cos 255^{\circ}$

Possible Answers:

$\frac{\sqrt{2}+\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}+\sqrt{6}}{4}$

$-\frac{1}{2}$ $\displaystyle -\frac{1}{2}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

$\frac{2\sqrt{2}}{4}$ $\displaystyle \frac{2\sqrt{2}}{4}$

Correct answer:

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

Explanation:

Using the identity, we can break up the 255 $\displaystyle 255$ into 300-45 $\displaystyle 300-45$ and then solve: $\cos 300\cdot \cos 45+\sin 300\cdot \sin 45\rightarrow \frac{1}{2}\cdot \frac{\sqrt{2}}{2}+\frac{-\sqrt{3}}{2}\cdot \frac{\sqrt{2}}{2}\rightarrow \frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \cos 300\cdot \cos 45+\sin 300\cdot \sin 45\rightarrow \frac{1}{2}\cdot \frac{\sqrt{2}}{2}+\frac{-\sqrt{3}}{2}\cdot \frac{\sqrt{2}}{2}\rightarrow \frac{\sqrt{2}-\sqrt{6}}{4}$ and so the correct answer is $\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$.

Report an Error

Example Question #2 : Trigonometric Identities

Is the following equation an identity?

$\cos \left ( x-\pi \right )=\cos \left ( x \right )$ $\displaystyle \cos \left ( x-\pi \right )=\cos \left ( x \right )$

Possible Answers:

The answer cannot be determined from the information provided.

No it is not an identity

Yes it is an identity

Correct answer:

No it is not an identity

Explanation:

$\cos \left ( x-\pi \right )=\cos \left ( x \right )\rightarrow \cos x\cos \pi +\sin x\sin \pi =\cos x\rightarrow \cos x(-1)+\sin x(0)=\cos x\rightarrow -\cos x\neq \cos x$ $\displaystyle \cos \left ( x-\pi \right )=\cos \left ( x \right )\rightarrow \cos x\cos \pi +\sin x\sin \pi =\cos x\rightarrow \cos x(-1)+\sin x(0)=\cos x\rightarrow -\cos x\neq \cos x$

Due to this inequality, this is not an identity.

Report an Error

Example Question #9 : Sum And Difference Identities

Use the sum or difference identity to find the exact value of $\sin 165^{\circ}$ $\displaystyle \sin 165^{\circ}$.

Possible Answers:

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

$\frac{\sqrt{6}}4{}$ $\displaystyle \frac{\sqrt{6}}4{}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$ $\displaystyle \frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}-\sqrt{2}}{4}$

$\frac{\sqrt{6}+\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}+\sqrt{2}}{4}$

Correct answer:

$\frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}-\sqrt{2}}{4}$

Explanation:

Here we break up the 165 $\displaystyle 165$ into 135+30 $\displaystyle 135+30$ and solve using the sin identity: $\sin 135\cdot \cos 30+\cos 135\cdot \sin 30\rightarrow \frac{\sqrt{2}}2{}\cdot \frac{\sqrt{3}}2{}+\frac{-\sqrt{2}}2{}\cdot \frac{1}{2}\rightarrow \frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \sin 135\cdot \cos 30+\cos 135\cdot \sin 30\rightarrow \frac{\sqrt{2}}2{}\cdot \frac{\sqrt{3}}2{}+\frac{-\sqrt{2}}2{}\cdot \frac{1}{2}\rightarrow \frac{\sqrt{6}-\sqrt{2}}{4}$ and so here the credited answer is $\frac{\sqrt{6}-\sqrt{2}}{4}$ $\displaystyle \frac{\sqrt{6}-\sqrt{2}}{4}$.

Report an Error

Example Question #1 : Trigonometric Identities

Use trigonometric identities to solve the following equation for $\theta$ $\displaystyle \theta$:

$tan^2\theta + sec^2\theta = 1$ $\displaystyle tan^2\theta + sec^2\theta = 1$

Possible Answers:

$\theta = \pi/4+ n\pi$ $\displaystyle \theta = \pi/4+ n\pi$

$\theta= n\pi$ $\displaystyle \theta= n\pi$

$\theta = \pi/3+ n$ $\displaystyle \theta = \pi/3+ n$

$\theta = \pi/2+ n\pi$ $\displaystyle \theta = \pi/2+ n\pi$

$\theta = \pi/3+ n$ $\displaystyle \theta = \pi/3+ n$

Correct answer:

$\theta= n\pi$ $\displaystyle \theta= n\pi$

Explanation:

Use the trigonometric identities to switch sec into terms of tan:

$tan^2\theta + sec^2\theta = tan^2\theta + (tan^2 \theta + 1) = 2tan^2\theta + 1 = 1$ $\displaystyle tan^2\theta + sec^2\theta = tan^2\theta + (tan^2 \theta + 1) = 2tan^2\theta + 1 = 1$

hence,

$2tan^2\theta = 0$ $\displaystyle 2tan^2\theta = 0$

So we have $\tan \theta = 0$ $\displaystyle \tan \theta = 0$, making $\theta= n \pi$ $\displaystyle \theta= n \pi$

Therefore the solution is $\theta = n \pi$ $\displaystyle \theta = n \pi$ for n being any integer.

Report an Error

View Pre-Calculus Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

View Pre-Calculus Tutors

Nate
Certified Tutor

University of Wisconsin-Oshkosh, non degree, Prepharmacy. University of Wisconsin-Madison, Pharmaceutical Chemist, Pharmacy.

View Pre-Calculus Tutors

Sayantan
Certified Tutor

Western Michigan University, Bachelor of Science, Applied Mathematics.

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in Atlanta, LSAT Tutors in Boston, Algebra Tutors in Houston, Computer Science Tutors in San Diego, Math Tutors in Los Angeles, MCAT Tutors in New York City, English Tutors in Miami, LSAT Tutors in Miami, Physics Tutors in Miami, ACT Tutors in Phoenix

Popular Courses & Classes

LSAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Denver, ACT Courses & Classes in Los Angeles, ISEE Courses & Classes in Denver, SAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Boston, SSAT Courses & Classes in Chicago, SSAT Courses & Classes in San Diego, SAT Courses & Classes in Seattle, SSAT Courses & Classes in Houston

Popular Test Prep

MCAT Test Prep in Dallas Fort Worth, MCAT Test Prep in Boston, ISEE Test Prep in Miami, SAT Test Prep in Chicago, GRE Test Prep in San Diego, MCAT Test Prep in Chicago, ISEE Test Prep in New York City, ACT Test Prep in Los Angeles, ISEE Test Prep in Los Angeles, ISEE Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Precalculus : Trigonometric Identities

Study concepts, example questions & explanations for Precalculus

All Precalculus Resources

Example Questions

Example Question #1 : Sum And Difference Identities

Example Question #1 : Sum And Difference Identities

Example Question #1 : Trigonometric Identities

Example Question #1 : Sum And Difference Identities

Example Question #1 : Sum And Difference Identities For Cosine

Example Question #1 : Trigonometric Identities

Example Question #1 : Sum And Difference Identities

Example Question #2 : Trigonometric Identities

Example Question #9 : Sum And Difference Identities

Example Question #1 : Trigonometric Identities

All Precalculus Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: