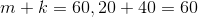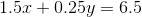All Pre-Algebra Resources
Example Questions
Example Question #1 : Word Problems With Two Unknowns
Combined, Megan and Kelly worked 60 hours. Kelly worked twice as many hours as Megan. How many hours did they each work?
Megan worked for 30 hours and Kelly worked for 60 hours
Megan worked for 20 hours and Kelly worked for 40 hours
Megan worked for 40 hours and Kelly worked for 10 hours
Megan worked for 10 hours and Kelly worked for 50 hours
Megan worked for 15 hours and Kelly worked for 45 hours
Megan worked for 20 hours and Kelly worked for 40 hours
Step 1: Megan and Kelly's total hours worked needs to add up to 60, and Kelly worked two times as long as Megan. We can put this into a formula:
Step 2: substitute 2m for k and add the variables
Step 3: isolate m
Step 4: Now that we know Megan worked 20 hours (m=20), we can multiply her hours worked by 2 to find out how long Kelly worked.
Step 5: check to make sure Megan and Kelly's hours add up to 60
Example Question #2 : Word Problems With Two Unknowns
Jamal invites 15 people to his birthday party and orders enough cupcakes so that everyone (himself included) will get two cupcakes. How many cupcakes can everyone have if only 7 friends show up to Jamal's party?
Step 1: find the number of cupcakes ordered by adding up all of the people at the party and then multiplying that number by the 2 cupcakes ordered per person.
Step 2: to find the number of cupcakes each person can have, take the number of cupcakes and divide it by the number of guests, including Jamal.
Example Question #1 : Word Problems With Two Unknowns
Michael and Tom are brothers. Their combined age is 20, and Tom is 4 years older than Michael. What are Michael and Tom's ages?
Tom is 16 years old and Michael is 4 years old.
Michael is 12 years old and Tom is 8 years old.
Michael is 10 years old and Tom is 10 years old.
Tom is 10 years old and Michael is 4 years old.
Michael is 8 years old and Tom is 12 years old.
Michael is 8 years old and Tom is 12 years old.
To solve this, we can set each of their ages as a variable. Let's say Michael's age is x.
We know Tom is 4 years older than Michael, so Tom's age is x+4.
We also know that their combined age is 20, so if we add both of their ages, we should get 20.
x + (x+4) = 20
2x+4 = 20
2x=16
x=8.
So Michael's age is 8, and Tom is 12.
Example Question #3 : Word Problems With Two Unknowns
Sarah earns $10 an hour selling calculators, and every time she sells a calculator, she earns an additional $3 comission. Jamie also sells calculators, and earns $30 an hour, but only earns an additional $1 comission for every calculator she sells.
How many calculators per hour on average would Sarah have to sell to be making as much as Jamie would per hour, if Jamie sold the same number of calculators?
Answer cannot be determined from the information given
First, set up the equations. Their base pay is constant per hour, so the variable is the number of calculators sold multiplied by their comission rate, so the earning equations will be :
and
These are Sara's earnings and Jamie's earnings respectively, with 

Because we are trying to find the number of calculators sold when both women have equal earnings, we can set 


Next, subtract 

Which simplifies to
Example Question #4 : Word Problems With Two Unknowns
Jamarcus has twenty-one coins in his piggy bank, all of which are either dimes or quarters. If Jamarcus has 
11 dimes and 10 quarters
14 dimes and 7 quarters
10 dimes and 11 quarters
7 dimes and 14 quarters
12 dimes and 12 quarters
7 dimes and 14 quarters
We can solve this problem by setting up an algebra equation. We know Jamarcus has twenty-one coins, but we don't know how many of each he has. That usually means we need a variable. Since we don't know how many dimes he has, let's label d as the number of dimes. If we want to find the number of quarters, we would subtract the number of dimes from 21, and the number we get would be the number of quarters. Therefore, if Jamarcus hs 

Now, the only other piece of information we have is that together all 21 coins add up to $4.20. At first that might not seem too helpful, but it actually allows us to solve the problem. We know that every dime is worth 10 cents, so every dime Jamarcus has contributes 10 cents towards his $4.20 total. Furthermore, each quarter contributes 25 cents to his total. Since Jamarcus has 



Next we use the distributive property to simplify, multiplying the 25 by both the 21 and the 
Simplifying further we get
We then want to combine like terms (the ds)
We then want all of our variables on one side and all of our constants on the other, which we can accomplish by subtracting 525 from both sides.

To solve for 


That means Jamarcus has 7 dimes. If we remember that he had 21 coins in all, that leaves 14 quarters. Jamarcus has 7 dimes and 14 quarters.
We can double check ourselves. Seven dimes would total $0.70, and 14 quarters would total $3.50, bringing the grand total to the correct value of $4.20.
Example Question #5 : Word Problems With Two Unknowns
The sum of two numbers is 128. The first number is 18 more than the second number. What are the two numbers?
We can solve this problem easily by using elementary algebra. We do not know either of the two numbers, so for the meantime let's label the second number as 

We can then combine like terms (our variables), giving us
We then want all of our constant terms on the right side, which we can accomplish by subtracting 18 from both sides.
The last step to solving the equation is to divide both sides by 2.
Therefore, our second number is 55. Since our first number is 18 more than that, it must equal 73. Double checking, we can confirm that the sum of 55 and 73 is indeed 128.
Example Question #2 : Word Problems With Two Unknowns
Turn the word equation into symbols.
The product of three and s and the difference of 12 and 7 is 14.
We need to translate the English words into a mathematical statement.
Product means multiply.
Difference means subtraction.
Is means equals.
Product of 3 and s is 3s.
Difference of 12 and 7 is 12 - 5.
Therefore, the equation becomes,

Example Question #7 : Word Problems With Two Unknowns
There are a total of 14 coins when dimes and nickels are combined. The total amount is 80 cents. How many dimes and nickels are there, respectively?
Write two equations to represent the scenario. There are two equations and two unknowns.
A total of 14 coins composed of dimes, 

Nickels are 5 cents, and dimes are 10 cents. The total is 80 cents. Write the second equation.
Multiply the second equation by 10 and use the elimination method to cancel out the dimes variable.
Subtract the first and the new second equation to eliminate 

Divide by 
There are 12 nickels.
Substitute this into the first equation to find the number of dimes.
There are 2 dimes and 12 nickels.
Example Question #9 : Word Problems With Two Unknowns
You go to the store and buy x bags of carrots and y bananas. Each bag of carrots costs $1.50 and each banana is $0.25. You spend $6.50. The total number of items you purchase is 11. How many bags of carrots did you buy? How many bananas did you buy?
6 bags of carrots, 5 bananas
5 bags of carrots, 6 bananas
3 bags of carrots, 8 bananas
4 bags of carrots, 7 bananas
8 bags of carrots, 3 bananas
3 bags of carrots, 8 bananas
Given the information, we have 2 equations. We know each bag of carrots is $1.50 and each banana is $0.25. We also know the total amount we spend is $6.50. So, we can write the equation
where x is the number of bags of carrots and y is the number of bananas.
We also know the total number of items we purchased is 11. We can write the equation as
where x is number of bags of carrots and y is the number of bananas.
To solve, we will solve for one variable in one equation and substitute it into the other equation. So,
Now, we can substitute the value of y into the first equation. We get,
We distribute.
We combine like terms.
We solve for x by getting x alone.
Therefore, the number of bags of carrots we bought is 3. To find the number of bananas, we simply substitute x into the equation.
Therefore, the number of bananas we bought is 8.
So we bought 3 bags of carrots and 8 bananas.
Certified Tutor
Certified Tutor
All Pre-Algebra Resources