Since Janet can pick between 10 and 20 tomatoes in an hour, there is a range for which she can pick 120 tomatoes. Therefore, to find the possible amount of time it will take Janet to pick 120 tomatoes set up two fractions.

The first fraction will use the rate of 10 tomatoes an hour.

Therefore, identifying the variables are as follows.

Substituting these into the fraction results in a possible time

The second fraction will use the rate of 20 tomatoes an hour.

Therefore, identifying the variables are as follows.

Substituting these into the fraction results in a possible time

Of the answer selections 6 hours would be the correct answer.

The algebraic expression for  being Jamie's youngest brother's age is:

Jamie's youngest brother is five, the next oldest brother is seven, and Jamie is 15.

Given the graph of record sales, to find the fraction of records that were sold in 2004 to 2010 first identify the record sales in 2004 and the record sales in 2010.

Examining the graph,

Record sales in 2004:14 million

Record sales in 2010: 13 million

From here, to find the fraction of records sold during this time period, use the following formula.

Fred has $100 in quarters and nickels initially.  We are also told that he has 260 quarters.  This is worth $65.  Thus Fred initially has $35 in nickels or 700 nickels.

Fred now exchanges some of his nickels for the dimes of a friend.  He ends up with 650 coins.  We know that Fred started with 960 coins (700 nickels + 260 quarters).  He ends up with 650 coins.  The number of quarters remains unchanged, meaning he now has 390 nickels and dimes.  These must have the same value as the initial 700 nickels, though, since he didn't lose any money.

Now we can finally set up our solution:

Thus Fred has 80 nickels and 310 dimes.

An alternative solution step is to notice that turning nickels into dimes always occurs in exactly one way: 2 nickels to 1 dime.  Every time you do this conversion, you will lose exactly one coin.  We then notice that the number of coins drops from 960 to 650, or drops by 310 coins.  We thus need to get rid of 310 coins.  Since we're only allowed to change nickels into dimes (and lose 1 coin each time), we simply do this 310 times to reach the requisite number of coin losses.  We are left with the proper number of coins with the proper value immediately.  Since every replacement replaced 2 nickels, we also lost  nickels.  Our final number of nickels is thus  nickels.

In order to find the volume of the figure, we will first need to find the volume of both cylinders.

Recall how to find the volume of the cylinder:

Now, use the given radius and height to find the volume of the larger cylinder.

Next, use the given radius and height to find the volume of the smaller cylinder.

Subtract the volume of the smaller cylinder from the volume of the larger one to find the volume of the figure.

Make sure to round to  places after the decimal.

Since both fractions in the expression have a common denominator of , we can combine like terms into a single numerator over the denominator:

This problem requires knowledge of the compound interest formula, 

Where  is the amount of money owed,  is the sum borrowed,  is the yearly interest rate,  is the amount of times the interest is compounded per year, and  is the number of years.  

We know that the student borrowed $200,000 compounded annually at a 6% interest rate, therefore by plugging in those numbers we find that after she graduates in 4 years she will owe $252,495.

This problem requires knowledge of the compound interest formula, 

Where  is the amount of money owed,  is the sum borrowed,  is the yearly interest rate,  is the amount of times the interest is compounded per year, and  is the number of years.  

We know that the student borrowed $200,000 compounded quarterly at a 6% interest rate, therefore by plugging in those numbers we find what she will owe after she graduates in 4 years.

The area of  is , the area of  is . Therefore, you can fit 5.06  in .

Alvin runs at a rate of seven miles an hour for twelve minutes, or 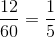 hours. The distance he runs is equal to his rate multiplied by his time, so, setting in this formula:

 miles.

One mile comprises 5,280 feet, so this is equal to 

 feet

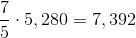
Since each side of the track measures 264 feet, this means that Alvin runs 

 sidelengths.

,

which means that Alvin runs around the track four complete times, plus four more sides of the track. Alvin stops when he is at Point E.