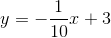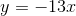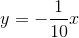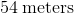All New SAT Math - Calculator Resources
Example Questions
Example Question #191 : New Sat
A truck was bought for 

The first step is to convert the depreciation rate into a decimal. 




Example Question #192 : New Sat
What is 
To convert degrees to radians, we need to remember the following formula.

Now lets substitute for degrees.
Example Question #193 : New Sat

Lines P and Q are parallel. Find the value of 
Since these are complementary angles, we can set up the following equation.
Now we will use the quadratic formula to solve for 
Note, however, that the measure of an angle cannot be negative, so 
Example Question #194 : New Sat
Find the equation of a line that goes through the points 

For finding the equation of a line, we will be using point-slope form, which is



We will pick the point
If we picked the point
We get the same result
Example Question #195 : New Sat
Find the equation of a line that passes through the point 

Since we want a line that is parallel, we will have the same slope as the line 



Example Question #196 : New Sat
A rectangular garden has an area of 

We define the variables as 

We substitute these values into the equation for the area of a rectangle and get 

Lengths cannot be negative, so the only correct answer is 


Therefore, 
Example Question #197 : New Sat
If 

Subtract 


Divide both sides by 
Example Question #198 : New Sat

What is the equation of the graph?
In order to figure out what the equation of the image is, we need to find the vertex. From the graph we can determine that the vertex is at 
Recall vertex form,





Plugging in our values, we get
To solve for 
I will pick 
Now our equation is
Let's expand this,
Example Question #199 : New Sat
Which expression is equivalent to the following quotient? For 
All we need to do is remember the quotient rule for exponents.
We apply this to each term and we get the following.
Example Question #105 : Algebra
Solve for x:
The first step is to cancel out the denominator by multiplying both sides by 7:
Subtract 3 from both sides to get 
All New SAT Math - Calculator Resources