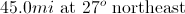Inconstant velocity implies a non-zero acceleration. A non-zero acceleration implies a non-zero force.

Circular motion is made possible by acceleration along the radius of the circle. Since there is acceleration, the linear velocity is not constant even if angular velocity is constant.

The other scenarios describe instances in which there is no net force, and thus no net acceleration or change in velocity.

Use the equation  to find the distance traveled in each part of the trip.

Part 1 (east): 

Part 2 (north): 

Part 3 (east): 

Find the total distance traveled in each direction.

Treat these components as sides of a right triangle, in which the hypotenuse represents the total displacement. Find the hypotenuse of this triangle using the Pythagorean theorem.

Now that we have the magnitude of the displacement, we still need to find the directional angle. The angle north of east can be found with an inverse trigonometry function, such as inverse tangent. We know that we are working with a triangle with sides of north and east, and the hypotenuse equal to the displacement. Orienting the angle, we can see that the opposite side is the northwards displacement, and the adjacent side is the eastwards displacement.

The average velocity is equal to displacement over time.

The displacement of the race car is zero because the ending position was the same as the starting position. The average velocity will therefore also be equal to zero. 

Remember that velocity is a vector, while speed is a scalar. Velocity is measured in terms of displacement and speed is measured in terms of distance.

Displacement is a vector quantity, so we cannot simply add the distances. Instead, we will need to triangulate the position of the plane.

We can draw the plane's path on a set of coordinate axes. The initial displacement will be in the vertical direction. The plane will then travel an equivalent distance at an angle of to the horizontal ( to the original flight path), essentially with a slope of one. By bisecting the angle created by the two flight paths, we can create two similar right triangles with hypotenuse lengths of . The measure of the bisected angle will be .

We need to solve for the side opposite the bisected angle, given the length of the hypotenuse. We will use the sine function.

This is the length on only one of the triangle sides. We need to double this distance to find the total displacement.

Since  we need to solve for the total distance around the track. 

Now, we can solve for speed using our equation. With 60 seconds per minute, 3 minutes is equal to 180 seconds.

Keep in mind that the question asked for the average speed, not velocity. Had it asked for the average velocity,  would be the correct answer, as the total displacement is zero.

Keep in mind that velocity is a vector, and a change in direction results in a change in velocity. When a runner runs in a circular manner around a track, the velocity of the runner will oscillate between a maximum positive velocity and a minimum negative velocity. As a result, this scenario matches the velocity vs. time graph appropriately. 

To solve this question, you must understand the difference between total distance and total displacement. While it is true that the baseball traveled a specific distance, the displacement is the final position of the baseball relative to the original position of the ball. The ball started and ended in the same place, so its total displacement is 0m.

At its peak height, the ball will have a velocity of ; however, the acceleration on the ball due to gravity is constantly experienced by the baseball. As a result, while in the air, the ball will always have a vertical acceleration of 

Near the earth's surface (neglecting air resistance), all objects in free-fall accelerate downwards at . This occurs even when the object's velocity is zero, for example when it is at the top of its flight.