All ISEE Upper Level Math Resources
Example Questions
Example Question #291 : Plane Geometry
Consider the rhombus below. Solve for 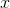

Possible Answers:
Correct answer:
Explanation:
The total sum of the interior angles of a quadrilateral is 
Example Question #292 : Plane Geometry

Note: Figure NOT drawn to scale.
The above depicts a rhombus and one of its diagonals. What is 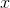
Possible Answers:
Correct answer:
Explanation:
The diagonals of a rhombus bisect the angles.
The angle bisected must be supplementary to the 
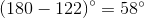

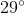
All ISEE Upper Level Math Resources
Popular Subjects
GMAT Tutors in Seattle, Calculus Tutors in New York City, Statistics Tutors in Denver, SSAT Tutors in Houston, English Tutors in Boston, Math Tutors in Denver, English Tutors in San Diego, English Tutors in Houston, SSAT Tutors in Washington DC, Computer Science Tutors in Denver
Popular Courses & Classes
ISEE Courses & Classes in New York City, Spanish Courses & Classes in Los Angeles, MCAT Courses & Classes in Seattle, LSAT Courses & Classes in Washington DC, SSAT Courses & Classes in Seattle, GMAT Courses & Classes in Boston, MCAT Courses & Classes in Washington DC, SAT Courses & Classes in New York City, ACT Courses & Classes in Houston, GMAT Courses & Classes in Houston
Popular Test Prep
GRE Test Prep in Dallas Fort Worth, SSAT Test Prep in Seattle, SAT Test Prep in San Francisco-Bay Area, GMAT Test Prep in Houston, MCAT Test Prep in Chicago, MCAT Test Prep in Phoenix, ISEE Test Prep in New York City, SSAT Test Prep in Los Angeles, GMAT Test Prep in Chicago, GMAT Test Prep in San Francisco-Bay Area
















