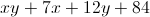This question is very simple to answer if you remember that ALL paralellograms have two pairs of equal and opposite angles, and that the four angles in any quadrilateral MUST add up to 360 degrees. 

Because the angles given are different, we know that they are supplementary and the other two missing angles MUST be the same.

Below is regular Pentagon  with center , a segment drawn from  to each vertex - that is, each of its radii drawn.

The measure of each angle of a regular pentagon can be calculated by setting  equal to 5 in the formula

and evaluating:

Specifically, 

By symmetry, each radius bisects one of these angles. Specifically, 

By the Same-Side Interior Angles Theorem, consecutive angles of a parallelogram are supplementary - that is, their measures total . However,

,

violating these conditions. Therefore, Quadrilateral  is not a parallelogram.

, making  and  supplementary. By the Converse of the Same Side Interior Angles Theorem, , it does follow that . However, without knowing the measures of the other two angles, nothing further can be concluded about Quadrilateral . Below are a parallelogram and a trapezoid, both of which have these two angles of these measures.

By the Same-Side Interior Angles Theorem, consecutive angles of a parallelogram can be proved to be supplementary - that is, their angle measures total . Specifically,  and are a pair of supplementary angles. Since they are also congruent, it follows that both are right angles. For the same reason,  and  are also right angles. The parallelogram, having four right angles, is a rectangle by definition.

The diagonals of a parallelogram are perpendicular - and, consequently,  is a right angle. - if and only if the parallelogram is a rhombus, a figure with four sides of equal length. Not all rectangles have four congruent sides.  Therefore,  need not be a right angle.

A rectangle is a parallelogram with four right angles.

Consecutive angles of a parallelogram are supplementary. If one angle of a parallelogram is given to be right, then its neighboring angles, being supplementary to a right angle, are right as well; also, opposite angles of a parallelogram are congruent, so the opposite angle is also right. All four angles must be right, making the parallelogram a rectangle by definition.

The answer is . 

You can find the perimeter by adding all of its respective sides as such:

. 

Adding like terms will result in  

 If you chose , you multiplied the two sides to find the area. 

If you chose , you only added two sides. Perimeter involves all 4 sides; so double the width and length. 

Just remember, the width is 12 added to .  Not 12 times the side of . 

If the area of a parallelogram is given as  with a height of , we can refer back to the equation for the area of a parallelogram:

, where  is height and  is the length of the base. 

This very quickly becomes a problem of substituting in values and finding the value of an unknown variable, in this case, : 

In order to find the height of this parallelogram apply the formula: 

To find the missing side of this parallelgram apply the formula: 

Thus, the solution is: