All Intermediate Geometry Resources
Example Questions
Example Question #41 : Acute / Obtuse Isosceles Triangles
Given: 

Which statement(s) must be true?
(a)
(b)
(a) but not (b)
(b) but not (a)
(a) and (b)
Neither (a) nor (b)
Neither (a) nor (b)
Neither similarity nor congruence of the two triangles follows from the statements given, as can be seen from the figure below:
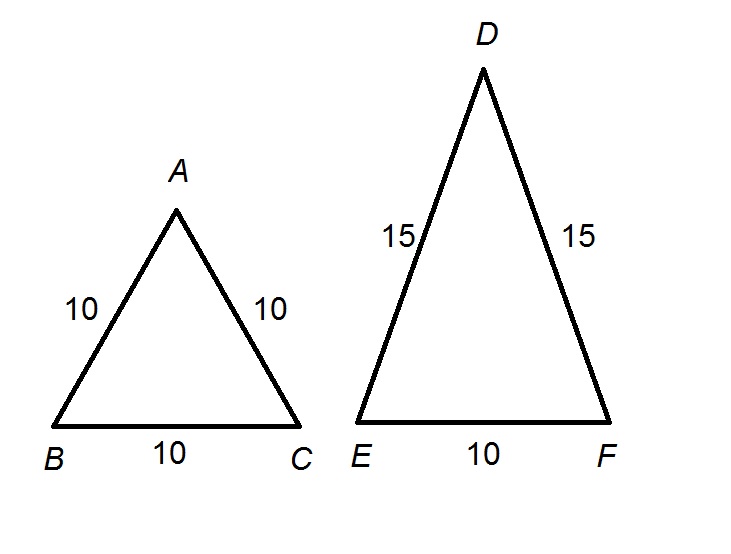

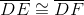

and

The triangles are not similar, and thus cannot be congruent either, so neither statement holds.
Example Question #491 : Plane Geometry
Find the perimeter of the triangle below.

Use the Pythagorean Theorem to find the base of the right triangle.
Now, because two of the angles in this triangle are the same, this is an isosceles triangle. In an isosceles triangle, the sides that are directly across from the congruent angles are also congruent.
In addition, the height in an isosceles triangle will always cut the 3rd side in half. With this information, fill out the triangle as shown below:

To find the perimeter, add up all the sides.
Example Question #51 : Acute / Obtuse Isosceles Triangles
Find the perimeter of the triangle below.

Use the Pythagorean Theorem to find the base of the right triangle.
Now, because two of the angles in this triangle are the same, this is an isosceles triangle. In an isosceles triangle, the sides that are directly across from the congruent angles are also congruent.
In addition, the height in an isosceles triangle will always cut the 3rd side in half. With this information, fill out the triangle as shown below:

To find the perimeter, add up all the sides.
Example Question #3 : How To Find The Length Of The Side Of Of An Acute / Obtuse Isosceles Triangle
A triangle has sides of lengths 14, 18, and 20. Is the triangle scalene or isosceles?
Scalene
Isosceles
Scalene
A triangle with three sides of different length is, by definition, scalene.
Example Question #52 : Acute / Obtuse Isosceles Triangles
Given: Regular Pentagon 




True or false: 
False
True
True
Below is regular Pentagon 



By symmetry, all of the radii of a regular pentagon are congruent - specifically, 
Example Question #1 : How To Find The Length Of The Side Of Of An Acute / Obtuse Isosceles Triangle
If a triangle has side lengths of 


The triangle inequality theorem states that the sum of the lengths of any two sides must be greater than the length of the third side. The relationship can be represented by the following inequalities:
The side length of 
Example Question #54 : Acute / Obtuse Isosceles Triangles
If a triangle has side lengths of 


The triangle inequality theorem states that the sum of the lengths of any two sides must be greater than the length of the third side. The relationship can be represented by the following inequalities:
The side length of 
Example Question #55 : Acute / Obtuse Isosceles Triangles
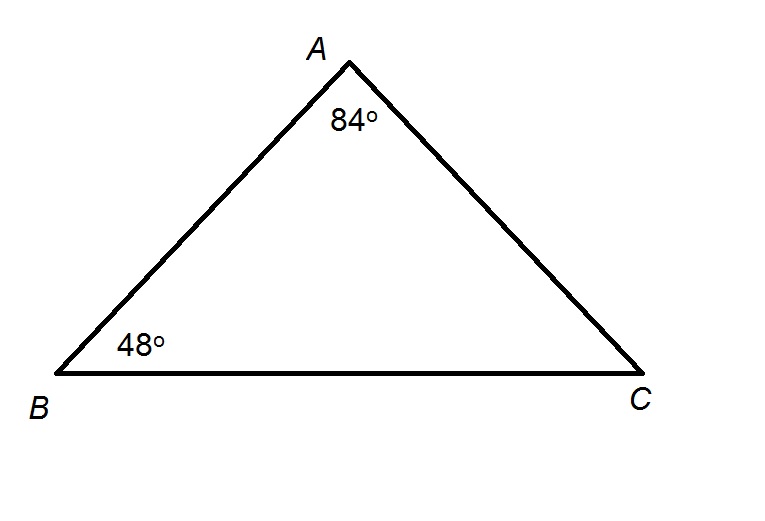
Figure NOT drawn to scale.
Refer to the above diagram.
True or false: 
False
True
True
The sum of the measures of the interior angles of a triangle is 
Substitute the given two angle measures and solve for 
Subtract 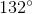

Example Question #1 : How To Find The Length Of The Side Of Of An Acute / Obtuse Isosceles Triangle

Refer to the above triangle. By what statement does it follow that 
The Converse of the Pythagorean Theorem
The Angle-Angle Postulate
The Converse of the Isosceles Triangle Theorem
The Hinge Theorem
The Angle-Side-Angle Postulate
The Converse of the Isosceles Triangle Theorem
We are given that, in 


Example Question #57 : Acute / Obtuse Isosceles Triangles
Given 



It cannot be determined from the information given.
Having three sides of different lengths, this triangle is scalene. In any scalene triangle, the angle with greatest measure is opposite the longest side, and the angle with least measure is opposite the shortest side. Therefore, since 

Certified Tutor
All Intermediate Geometry Resources