All High School Math Resources
Example Questions
Example Question #1 : Finding Domain And Range
What is the domain of the function below:
The domain is defined as the set of all possible values of the independent variable 




That means that the expression under the radical is always positive and therefore 


Example Question #11 : Functions And Graphs
This function is:
symmetric about the origin
symmetric about the x-axis
not symmetric
symmetric about the y-axis
symmetric about the y-axis
A function's symmetry is related to its classification as even, odd, or neither.
Even functions obey the following rule:
Because of this, even functions are symmetric about the y-axis.
Odd functions obey the following rule:
Because of this, odd functions are symmetric about the origin.
If a function does not obey either rule, it is neither odd nor even. (A graph that is symmetric about the x-axis is not a function, because it does not pass the vertical line test.)
To test for symmetry, simply substitute 
Thus, this equation is even and therefore symmetric about the y-axis.
Example Question #2021 : High School Math
A function is defined by the following rational equation:
What are the horizontal and vertical asymptotes of this function's graph?
To find the horizontal asymptote, compare the degrees of the top and bottom polynomials. In this case, the two degrees are the same (1), which means that the equation of the horizontal asymptote is equal to the ratio of the leading coefficients (top : bottom). Since the numerator's leading coefficient is 1, and the denominator's leading coefficient is 2, the equation of the horizontal asymptote is 
To find the vertical asymptote, set the denominator equal to zero to find when the entire function is undefined:
Example Question #1 : Function Properties
A function is defined by the following rational equation:
What line does 

This question is asking for the equation's slant asymptote. To find the slant asymptote, divide the numerator by the denominator. Long division gives us the following:
However, because we are considering 
Example Question #1 : Polynomials
Factor the polynomial if the expression is equal to zero when 
Knowing the zeroes makes it relatively easy to factor the polynomial.
The expression 
Now we need to check the answer.
We are able to get back to the original expression, meaning that the answer is 
Example Question #2031 : High School Math
A polyomial with leading term 
Since 6 is a triple root, and the degree of the polynomial is 3, the polynomial is 
Example Question #1 : Express A Polynomial As A Product Of Linear Factors
A polyomial with leading term 
Since 5 is a single root and 7 is a double root, and the degree of the polynomial is 3, the polynomial is 
Example Question #3 : Understanding Zeros Of A Polynomial
What are the solutions to 
When we are looking for the solutions of a quadratic, or the zeroes, we are looking for the values of 
Then, we are looking for the values where each of these factors are equal to zero.

and 
Thus, these are our solutions.
Example Question #1 : Finding Zeros Of A Polynomial
Find the zeros of the following polynomial:
First, we need to find all the possible rational roots of the polynomial using the Rational Roots Theorem:
Since the leading coefficient is just 1, we have the following possible (rational) roots to try:
±1, ±2, ±3, ±4, ±6, ±12, ±24
When we substitute one of these numbers for 

Since the function equals zero when 


Now we can factor the function this way:
We repeat this process, using the Rational Roots Theorem with the second term to find a possible zero. Let's try 
When we factor using synthetic substitution for 
Using our quadratic factoring rules, we can factor completely:
Thus, the zeroes of 
Example Question #11 : Functions And Graphs
Simplify the following polynomial:
To simplify the polynomial, begin by combining like terms:
All High School Math Resources


























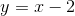





















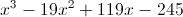

















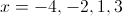

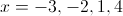


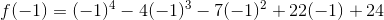





(x%5E%7B3%7D-5x%5E%7B2%7D-2x+24))
![f(-2)=[(-2)+1][(-2)^{3}-5(-2)^{2}-2(-2)+24]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/106228/gif.latex)















