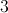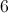Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : How to do absolute value in pre-algebra

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Do Absolute Value In Pre Algebra

Solve the expression below.

$7+\left | 5-9 \right |$

Possible Answers:

Correct answer:

Explanation:

$7+\left | 5-9 \right |=7+\left | {-4} \|$

For an absolute value, the term inside the bars will always become positive.

$7+\left | -4 \right |=7+4=11$

Report an Error

Example Question #2 : How To Do Absolute Value In Pre Algebra

Solve the expression below.

$6-3\left | 4- 6 \right |_.$

Possible Answers:

Correct answer:

Explanation:

$6-3\left | 4-6 \right |=6-3\left | -2 \right |$

For an absolute value, the term inside the bars will always become positive.

$6-3\left | -2 \right |=6-3(2)=6-6=0$

Report an Error

Example Question #3 : How To Do Absolute Value In Pre Algebra

Solve the absolute value expression.

$\small \small 6\left | 3-8 \right |+14$

Possible Answers:

Correct answer:

Explanation:

$\small \small 6\left | 3-8 \right |+14$

Simplify the absolute value, as if it were a parenthesis.

$\small \small 6\left | -5 \right |+14$

The absolute value of is . Remember that absolute values are always positive.

$\small (6)(5)+14$

$\small 30+14=44$

Report an Error

Example Question #3 : How To Do Absolute Value In Pre Algebra

Is the inequality below true or false?

$\small \small \left | -7 \right |>12-6$

Possible Answers:

The answer cannot be determined

False; the two terms are equal

True

False; the sign should be changed to "less than"

Correct answer:

True

Explanation:

An absolute value is always the positive solution.

$\small \left | -7 \right |=7$

We can compare the terms by substituting the positive value of , and solving the right side of the inequality.

$\small \small \small \left | -7 \right |>12-6$

$\small \left | -7 \right |>6$

$\small 7>6$

The inequality is a true expression.

Report an Error

Example Question #5 : How To Do Absolute Value In Pre Algebra

Evaluate $\left | -6+5-2 \right |$

Possible Answers:

Correct answer:

Explanation:

To solve problems with absolute value, first solve inside the absolute value signs. -6+5-2 = -3 .

The absolute value of is because the negative sign is inside the absolute value signs. Remember the absolute value is the distance from that number to zero on the number line. Therefore, the absolute value is always positive.

Report an Error

Example Question #6 : How To Do Absolute Value In Pre Algebra

Evaluate the expression.

$\left | 3-5\right |$

Possible Answers:

Correct answer:

Explanation:

First, treat the absolute value as a parenthesis, and evaluate the term inside.

$\left | 3-5\right |$

$\left | -2\right |$

The absolute value of any term is its distance from zero. A distance cannot be negative, thus, any negative term in an absolute value will be converted to a positive term.

$\left | -2\right |=2$

Report an Error

Example Question #4 : How To Do Absolute Value In Pre Algebra

$\left |-3 \right |=?$

Possible Answers:

Correct answer:

Explanation:

The absolute value of a number can be thought of as its distance from zero. is three units away from zero. Therefore, $\left |-3 \right |=3$ .

Report an Error

Example Question #8 : How To Do Absolute Value In Pre Algebra

$\left | 3^2-12\right |=?$

Possible Answers:

$\frac{3}{4}$

Correct answer:

Explanation:

The absolute value of a number can be thought of as its distance from zero.

We start by solving the expression inside the absolute value signs and then measure how far that is from zero.

$\left | 3^2-12\right |$

$=\left | 9-12\right |$

$=\left | -3\right |$

is three units away from zero. Therefore, $\left | 3^2-12\right |=\left |-3 \right |=3.$

Report an Error

Example Question #5 : How To Do Absolute Value In Pre Algebra

$\left | -5+3\right |=?$

Possible Answers:

$2\tfrac{1}{2}$

Correct answer:

Explanation:

The absolute value of a number can be thought of as its distance from zero.

We start by solving the expression inside the absolute value signs and then measure how far that is from zero.

$\left | -5+3\right |$

$=\left | -2\right |$

is two units away from zero. Therefore $\left | -5+3\right |=\left | -2\right |=2.$

Report an Error

Example Question #10 : How To Do Absolute Value In Pre Algebra

$\left | 12+24\right |=?$

Possible Answers:

$\frac{1}{2}$

Correct answer:

Explanation:

The absolute value of a number can be thought of as its distance from zero.

We start by solving the expression inside the absolute value signs and then measure how far that is from zero.

$\left | 12+24\right |$

$=\left | 36\right |$

is units away from zero. That means that $\left | 12+24\right |=\left | 36\right |=36.$

Report an Error

View Tutors

Anne
Certified Tutor

University of Maryland-Baltimore, Doctor of Science, Accounting.

View Tutors

Satweka
Certified Tutor

The University of Texas at Dallas, Master of Science, Biomedical Engineering.

View Tutors

Tonja
Certified Tutor

McNeese State University, Bachelor in Arts, English.

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GMAT Tutors in Phoenix, MCAT Tutors in San Francisco-Bay Area, SSAT Tutors in Atlanta, ISEE Tutors in Denver, GMAT Tutors in Miami, Spanish Tutors in Phoenix, Algebra Tutors in Boston, Reading Tutors in Denver, ACT Tutors in Boston, Computer Science Tutors in Houston

Popular Courses & Classes

GMAT Courses & Classes in Philadelphia, GMAT Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in San Francisco-Bay Area, GMAT Courses & Classes in Chicago, ACT Courses & Classes in Chicago, MCAT Courses & Classes in Denver, Spanish Courses & Classes in Philadelphia, GRE Courses & Classes in Atlanta, ISEE Courses & Classes in Washington DC, ISEE Courses & Classes in Denver

Popular Test Prep

MCAT Test Prep in Los Angeles, ACT Test Prep in Chicago, GMAT Test Prep in Chicago, GRE Test Prep in Dallas Fort Worth, ISEE Test Prep in Denver, LSAT Test Prep in San Diego, GMAT Test Prep in Washington DC, ISEE Test Prep in San Francisco-Bay Area, GMAT Test Prep in Dallas Fort Worth, LSAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : How to do absolute value in pre-algebra

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #1 : How To Do Absolute Value In Pre Algebra

Example Question #2 : How To Do Absolute Value In Pre Algebra

Example Question #3 : How To Do Absolute Value In Pre Algebra

Example Question #3 : How To Do Absolute Value In Pre Algebra

Example Question #5 : How To Do Absolute Value In Pre Algebra

Example Question #6 : How To Do Absolute Value In Pre Algebra

Example Question #4 : How To Do Absolute Value In Pre Algebra

Example Question #8 : How To Do Absolute Value In Pre Algebra

Example Question #5 : How To Do Absolute Value In Pre Algebra

Example Question #10 : How To Do Absolute Value In Pre Algebra

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: