All High School Chemistry Resources
Example Questions
Example Question #11 : Gases And Gas Laws
A gas occupies a volume of 


Charles's law of gases indicates that, at a constant pressure, the volume of a gas is proportional to the temperature. This is calculated by the following equation:
Our first step to solving this equation will be to convert the given temperature to Kelvin.
Using this temperature and the given volumes, we can solve for the final temperature of the gas.
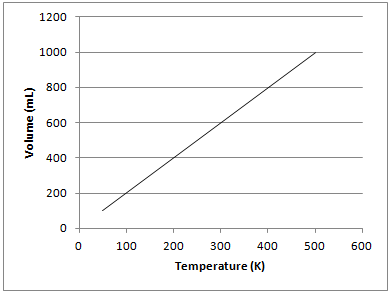
Example Question #11 : Gases And Gas Laws
The graph depicted below represents which of the gas laws?
Charles's law
Newton's third law
Boyle's law
Gay-Lussac's law
Charles's law
The graph shows that there is a directly proportional relationship between the volume of a gas and temperature in Kelvin when kept at a constant pressure. This is known as Charles’s law and can be represented mathematically as follows:
Gay-Lussac's law shows the relationship between pressure and temperature. Boyle's law shows the relationship between pressure and volume. Newton's third law is not related to gas principles and states that for every force on an object, there is an equal and opposite force of the object on the source of force.
Example Question #11 : Gases And Gas Laws
A balloon filled with room temperature air (


We expect the volume to increase since volume and temperature are directly proportional. We know that if we heat something the material will expand so we shouldn't get a value that is smaller than our initial volume. Charles Law says that
where the stuff on the left is the initial volume and temperature and the stuff on the right is the final volume and temperature. First off, we MUST convert the temperatures to Kelvin to use Charles Law. This gives
Solving for the final volume,
Example Question #12 : Gases And Gas Laws
An ideal gas exerts a pressure of 


What will be the final pressure if the volume of the container changes to 
Since the volume of the gas is the only variable that has changed, we can use Boyle's law in order to find the final pressure. Since pressure and volume are on the same side of the ideal gas law, they are inversely proportional to one another. In other words, as one increases, the other will decrease, and vice versa.
Boyle's law can be written as follows:
Use the given volumes and the initial pressure to solve for the final pressure.
Example Question #2 : Using Boyle's Law
What law is the following formula?
Charles's law
Gay-Lussac's law
Combined gas law
Boyle's law
Ideal gas law
Boyle's law
Boyle's law relates the pressure and volume of a system, which are inversely proportional to one another. When the parameters of a system change, Boyle's law helps us anticipate the effect the changes have on pressure and volume.
Charles's law relates temperature and volume:
Gay-Lussac's law relates temperature and pressure:
The combined gas law takes Boyle's, Charles's, and Gay-Lussac's law and combines it into one law:
The ideal gas law relates temperature, pressure, volume, and moles in coordination with the ideal gas constant:
Example Question #1 : Using Boyle's Law
A helium balloon has a volume of 



To solve this question we will need to use Boyle's law:
We are given the final pressure and volume, along with the initial volume. Using these values, we can calculate the initial pressure.
Note that the pressure at sea level is equal to 

Example Question #13 : Gases And Gas Laws
The graph depicted here represents which of the gas laws?
Gay-Lussac's law
Hund's law
Boyle's law
Charles's law
Boyle's law
The graph shows that there is an inverse relationship between the volume and pressure of a gas, when kept at a constant temperature. This was described by Robert Boyle and can be represented mathematically as Boyle's law:
Gay-Lussac's law shows the relationship between pressure and temperature. Charles's law shows the relationship between volume and temperature. Hund's rule (Hund's law) is not related to gases, and states that electron orbitals of an element will be filled with single electrons before any electrons will form pairs within a single orbital.
Example Question #1 : Using Boyle's Law
A sample of oxygen gas has a volume of 


To solve this question we will need to use Boyle's law:
We are given the initial pressure and volume, along with the final pressure. Using these values, we can calculate the final volume.
Example Question #14 : Gases And Gas Laws
A gas is initially in a 5L piston with a pressure of 1atm.
If pressure changes to 3.5atm by moving the piston down, what is new volume?
Use Boyle's Law:
Plug in known values and solve for final volume.
Example Question #14 : Gases And Gas Laws
A balloon of volume 


Use Boyle's law and plug in appropriate parameters:
All High School Chemistry Resources