All High School Chemistry Resources
Example Questions
Example Question #61 : Acid Base Chemistry
An arterial blood sample from a patient has a pH of 7.4. One day later, the same patient has an arterial blood pH of 7.15. How many times more acidic is the patient's blood on the second day?
The equation to calculate pH is:
The normal pH of arterial blood is around 7.4. This reflects a concentration of hydrogen ions that can be found using the pH equation.
Using similar calculations for the second blood sample, we can find the hydrogen ion concentration again.
Now that we have both concentrations, can find the ratio of the acidity of the two samples.
You may know from biological sciences that this is approaching a lethal level of acidosis.
Example Question #11 : Calculating P H And P Oh
You find a bottle in a lab that has a 
What is the pH of this solution?

Example Question #11 : Calculating P H And P Oh
Calculate the pOH and hydroxide ion concentration in an organism whose blood pH was measured to be 8.00 at 
To find the pOH note that 

We find the concentration of hydroxide ions based on the formula for pOH:
![[OH^-]=antilog(-pOH)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/470177/gif.latex)
Example Question #63 : Acid Base Chemistry
Calculate the pH of the following solution at 
Use the the dissociation constant for water 
Rearrange the dissociation constant to solve for the hydrogen ion concentration:
Plug in the given concentrations to find the actual hydrogen ion concentration:
Convert the hydrogen ion concentration to pH scale:
Example Question #61 : Acid Base Chemistry
Calculate the 


Relevant equations:

Combine equations:
Plug in values:
Example Question #61 : Acid Base Chemistry
A 100mL solution is composed of 25% ethanol by volume and water. What is the mass of the solution?
First we determine the mass of the ethanol in solution using its density. Using the percent by volume of ethanol, we know that there are 25mL of ethanol in a 100mL solution. The remaining 75mL are water.
Since the density of water is 1g/mL, we know that the mass of 75mL of water is 75g. The total mass is the sum of the ethanol and the water.
Example Question #1 : Titrations
What is the concentration if 40 g NaOH is dissolved in 1000 g of water
first of all, M = molar; m = molal- M = mol solute/ L of solution; m = mol solute/ kg solvent
you have 40 g NaOH * 1 mol/40 g = 1 mol
1000 g of water is equivalent to 1 L
1 mol/L = 1M
Example Question #1 : Identifying Unknown Concentration
80.0g NaOH is put into 50000 mL water. What is the molarity of the resulting solution?
1.6 M
0.625 M
0.00004 M
0.04 M
0.0016 M
0.04 M
Molarity = mol solute / L soution
mol solute = 80 g NaOH * 1 mol / 40 g = 2 mol
L solution = 50000 mL water * 1 L/1000 mL = 50 L
2 mol / 50 L =
Example Question #1 : Identifying Unknown Concentration
How many liters of 10M HCl are needed to make 4L of 0.4M solution?
A simple calculation can be done to perform any solution dilution problem. We know our equation 
We can rewrite this as 
Using this formula, we take the old solution and set it equal to the new solution.
We need 0.16 liters of our 10 molar solution.
Example Question #62 : Acid Base Chemistry
Which solution has the highest molarity?
This question requires us to calculate molarity for each answer choice. It is important to add everything correctly and be careful with more complex compounds.
Molarity is simply moles of solute over liters of solution. The correct answer, after trying each, is the answer with lead (II) nitrate, as it gets us a molarity of 2.
All High School Chemistry Resources







![\small \left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110187/gif.latex)
![\small 7.4 = - log\left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/98016/gif.latex)
![\small 3.98 *10^{-8}M = \left [ H^{+}\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/123319/gif.latex)
![7.15=-log[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/98017/gif.latex)
![7.08*10^{-8}M=[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110188/gif.latex)
![\frac{[H^+_2]}{[H^+_1]}=\frac{7.08*10^{-8}}{3.98*10^{-8}}=1.78](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/259310/gif.latex)


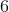



![[HNO_3]=[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/326585/gif.latex)
![pH=-log[H^+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/326586/gif.latex)


![[OH^-]=6.0*10^{-10}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471119/gif.latex)

![[OH^-]=1.0*10^{6}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471121/gif.latex)

![[OH^-]=1.0*10^{-6}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471117/gif.latex)

![[OH^-]=1.0*10^{-8}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471123/gif.latex)

![[OH^-]=8.0*10^{10}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471125/gif.latex)

![pOH=-log[OH^-]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/470176/gif.latex)
![[OH^-]=antilog(-6)=10^{-6}={1.0*10^{-6}M}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471128/gif.latex)






![K_{w}=[H^+][OH^-]=(1.0* 10^{-7})(1.0* 10^{-7})=1.0*10^{-14}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471085/gif.latex)
![[H^+]=\frac{K_{w}}{[OH^-]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/470145/gif.latex)
![[H^+]=\frac{1.0* 10^{-14}}{1.0* 10^{-5}}=1.0*10^{-9}M](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471086/gif.latex)
![pH=-log[H^+]=-log(1.0* 10^{-9}M)=9.00](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/471087/gif.latex)





![pH=-log([H^+])](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/583007/gif.latex)
![pOH=-log([OH^-])](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/583008/gif.latex)

![[H^+]=[HCl]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/583012/gif.latex)
![pOH-log([H^+])=14](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/583014/gif.latex)
![pOH-log([.0750])=14](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/584906/gif.latex)





























