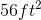GRE Math : How to multiply square roots
Study concepts, example questions & explanations for GRE Math
All GRE Math Resources
Example Questions
Example Question #54 : Arithmetic
Solve for 
You can "break apart" the fractional square root:
into:
Therefore, you can rewrite your equation as:
Now, multiply both sides by 
Now, you can square both sides of your equation and get:
Example Question #55 : Arithmetic
The length of a square courtyard is 
What is the area of the courtyard?
The area of a square is length squared. In this case, that would be 
The square root of a base squared is then just the base:
Lastly, we apply the appropriate units, therefore the area of the courtyard is 
Example Question #56 : Arithmetic
Begin by multiplying what is under the square roots together. Remember:
Therefore:
We must now try to reduce the square root. Look for factors that can be taken out that can be easily square-rooted. It's a good idea to start with small square roots 
Now take the square root of 9, and we are left with our answer:
Example Question #57 : Arithmetic
Solve:
To solve this problem, we must know how to multiply/divide with square roots. The rules involved are quite simple. When multiplying square roots together, multiply the coeffcients of the two numbers together and then multiply the two radicands together. The product of the radicand becomes the new radicand and the product of the coeffcients become the new coeffcient. The radicand is simply the number within the square root.
Dividing square roots follows the exact same rules as multiplying square roots, divide the coeffcients together, the result is the coeffcient for final answer. Do the same for the radicands.
All GRE Math Resources
