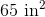Call Now to Set Up Tutoring:

(888) 888-0446

Common Core: 7th Grade Math : Solve for area of a triangle

Study concepts, example questions & explanations for Common Core: 7th Grade Math

Create An Account

All Common Core: 7th Grade Math Resources

7 Diagnostic Tests 110 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Solve For Area Of A Triangle

Calculate the area of the provided figure.

Possible Answers:

$50\textup{ in}^2$

$70\textup{ in}^2$

$35\textup{ in}^2$

$65\textup{ in}^2$

$45\textup{ in}^2$

Correct answer:

$35\textup{ in}^2$

Explanation:

In order to solve this problem, we need to recall the area formula for a triangle:

$A=\frac{b\times h}{2}$

Now that we have the correct formula, we can substitute in our known values and solve:

$A=\frac{14\times5}{2}$

$A=35\textup{ in}^2$

Report an Error

Example Question #1 : Solve For Area Of A Triangle

6 8 10

What is the area of the triangle pictured above?

Possible Answers:

Correct answer:

Explanation:

The area of a triangle is calculated using the formula $Area = \frac{1}{2}(Base\times Height)$ . Importantly, the height is a perpendicular line between the base and the opposite point. In a right triangle like this one, you're in luck: the triangle as drawn already has that perpendicular line as one of the two sides. So here we will calculate $\frac{1}{2}(8\times 6)$ . That gives us an answer of 24.

Report an Error

Example Question #2 : Solve For Area Of A Triangle

Abcd

In triangle ABC above, the distance between point A and point D is 10 inches, and the area of the triangle is 20 square inches. What is the length of side BC?

Possible Answers:

4 inches

There is not enough information to answer the question

10 inches

8 inches

2 inches

Correct answer:

4 inches

Explanation:

The area of a triangle can be calculated using the formula Area = 1/2 * Base * Height. Here you're given two of the unknowns in that formula:

Area = 20

Height = 10

So you can plug those into the area formula to solve for Base, the only remaining unknown:

20 = 1/2 * Base * 10

That means that 20 = 5 * Base, so Base = 4.

Report an Error

Example Question #3 : Solve For Area Of A Triangle

12abc

In triangle ABC, side BC measures 12 meters, and the shortest straight-line distance between point A and side BC is 5 meters long. What is the area of triangle ABC?

Possible Answers:

Correct answer:

Explanation:

The area of a triangle can be calculated using the formula $Area = \frac{1}{2}Base\times Height$ . The height of a triangle is a perpendicular line connecting the base and its opposite point; in any acute or right triangle - a triangle with no angles greater than 90 degrees - the height is also the shortest line between the base and the opposite point. Here that means that if you use BC = 12 as your base, then the distance of 5 between BC and point A is the height. That means that you can calculate the area:

$Area=\frac{1}{2}(12\times 5)=\frac{1}{2}(60)=30$

Report an Error

Example Question #4 : Solve For Area Of A Triangle

7 24 25

The pictured right triangle has sides of 7, 24, and 25. What is the area of that triangle?

Possible Answers:

125

128

Correct answer:

Explanation:

The area of a triangle can be calculated using the formula $Area = \frac{1}{2}(Base)(Height)$ . Note that the height of any triangle is a perpendicular line between the base and its opposite angle; in a right triangle that's very convenient, because the right angle gives you that perpendicular relationship between two sides. So you can use 24 as the base and 7 as the height here. That means that the area is:

$Area =\frac{1}{2}(24)(7)=84$

Report an Error

View Tutors

Rainier
Certified Tutor

Norfolk State University, Bachelors, Physics.

View Tutors

Marlene
Certified Tutor

Herbert Lehman, Bachelors, Recreation Education. Long Island University-Brooklyn Campus, Masters, Special Education.

View Tutors

Sarah
Certified Tutor

New York University, Bachelor of Fine Arts, Drama.

All Common Core: 7th Grade Math Resources

7 Diagnostic Tests 110 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in Dallas Fort Worth, LSAT Tutors in San Diego, Algebra Tutors in Chicago, Algebra Tutors in Atlanta, Algebra Tutors in San Francisco-Bay Area, Biology Tutors in San Francisco-Bay Area, GRE Tutors in Chicago, Reading Tutors in Dallas Fort Worth, French Tutors in Phoenix, LSAT Tutors in New York City

Popular Courses & Classes

ISEE Courses & Classes in Seattle, GRE Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Denver, ACT Courses & Classes in San Diego, GRE Courses & Classes in Atlanta, ISEE Courses & Classes in Phoenix, ISEE Courses & Classes in Houston, Spanish Courses & Classes in San Diego, SAT Courses & Classes in Los Angeles, LSAT Courses & Classes in Seattle

Popular Test Prep

ISEE Test Prep in Atlanta, ACT Test Prep in Boston, LSAT Test Prep in Atlanta, GMAT Test Prep in Chicago, SSAT Test Prep in Chicago, ACT Test Prep in San Diego, SSAT Test Prep in Boston, SSAT Test Prep in Phoenix, MCAT Test Prep in Denver, GRE Test Prep in San Francisco-Bay Area

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Common Core: 7th Grade Math : Solve for area of a triangle

Study concepts, example questions & explanations for Common Core: 7th Grade Math

All Common Core: 7th Grade Math Resources

Example Questions

Example Question #1 : Solve For Area Of A Triangle

Example Question #1 : Solve For Area Of A Triangle

Example Question #2 : Solve For Area Of A Triangle

Example Question #3 : Solve For Area Of A Triangle

Example Question #4 : Solve For Area Of A Triangle

All Common Core: 7th Grade Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: