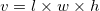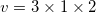All Common Core: 5th Grade Math Resources
Example Questions
Example Question #1741 : Common Core Math: Grade 5
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #1742 : Common Core Math: Grade 5
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #1743 : Common Core Math: Grade 5
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #81 : Volume Of A Three Dimensional Figure
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #82 : Volume Of A Three Dimensional Figure
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #35 : How To Find The Volume Of A Prism
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #711 : Geometry
What is the volume of the object below?

This object has 

Remember, volume is always labeled as units to the third power.
Example Question #1 : Apply The Volume Formula: Ccss.Math.Content.5.Md.C.5b
John has a gym locker that is 1 foot wide, 3 feet high, and 2 feet deep. His gym clothes can be stuffed into a gym bag with a volume of 4 cubic feet. Will John's gym bag fit into his locker?
No
Yes
Yes
Example Question #671 : Geometry
What is the volume of the shape below?

The formula for volume of a rectangular prism is
Remember, volume is always labeled as units to the third power.
Example Question #1 : How To Find The Volume Of A Prism
What is the volume of the shape below?

The formula for volume of a rectangular prism is
Remember, volume is always labeled as units to the third power.
Certified Tutor
Certified Tutor
All Common Core: 5th Grade Math Resources